Question

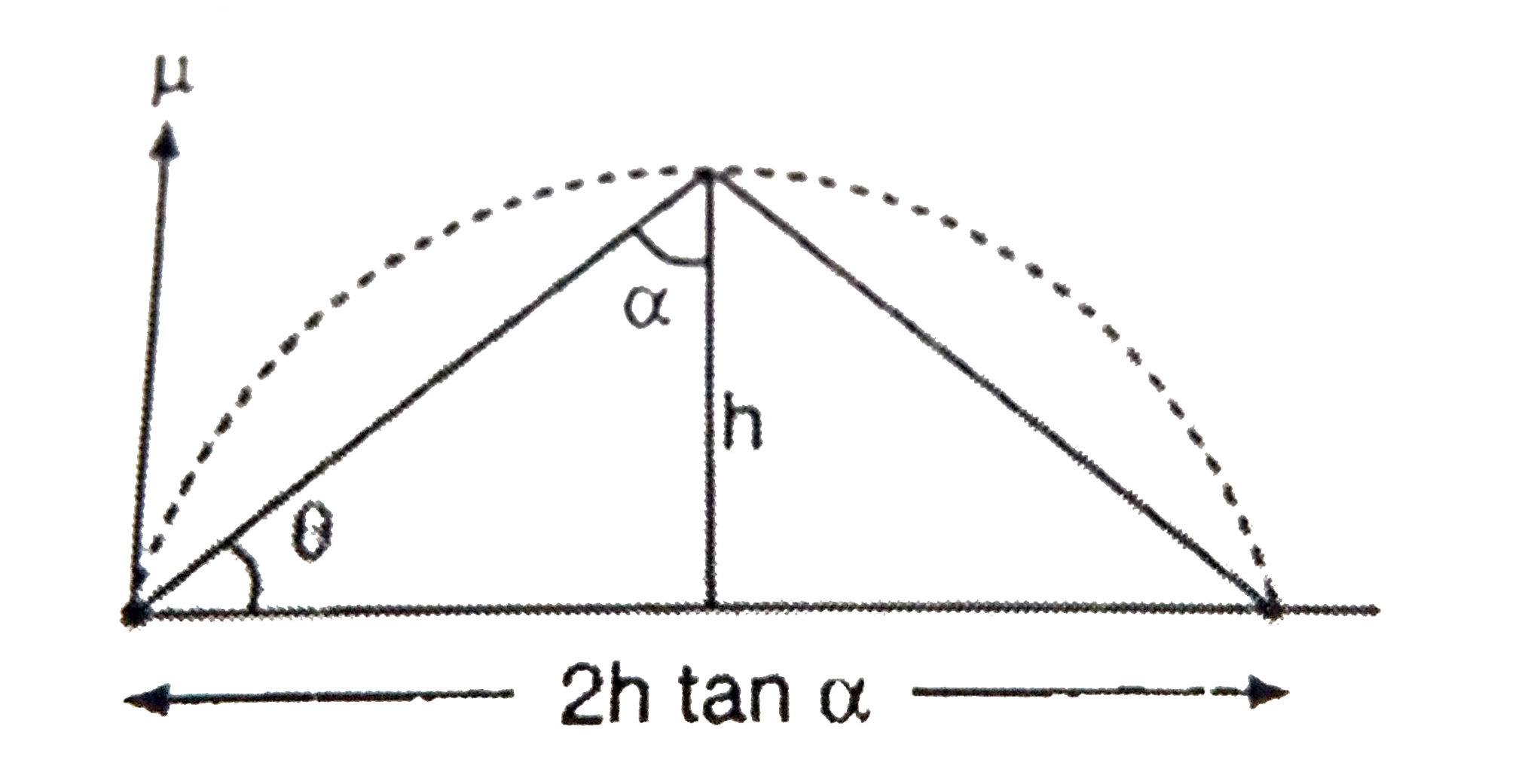
A projectile is fired from the base of cone shaped hill. The projectle grazes the vertex and strikes the hill again at the base. If ${\displaystyle \alpha }$ be the half-angle of the cone, h its height, u the initial velocity of projection and ${\displaystyle \theta }$ angle of projection, then ${\displaystyle \tan \theta \tan \alpha }$ is:

Answer

2

Explanation

Here range 2h `tan alpha=(u^(2)sin 2 theta)/(g)` and `h=(u^(2)sin^(2)theta)/(2g)`
Dividing `2 tan alpha=(2 sin 2theta)/(sin^(2)theta)rArr tan alpha=2cot theta`
`therefore u^(2)(2gh)/(sin^(2)theta)=(2gh)/(((2cotalpha)/sqrt(1+4cot^(2)alpha)))=(2gh(1+4cot^(2)alpha))/(4cot^(2)alpha)`
`gh((1)/(2)tan^(2)alpha+2)`
`tan theta=2cot alpha`