A particle is thrown over a triangle from one end of a horizontal base and after grazing the vertex falls on the other end of the base. If 30∘and60∘ be the base angles and θ the angle of projection then tanθ is
2√3
4√3
13
3
The correct Answer is:B
To solve the problem, we need to analyze the projectile motion of a particle thrown over a triangle with given angles at the base. The angles of the triangle are 30° and 60°, and we need to find the value of tanθ where θ is the angle of projection.
1. Identify the Angles:
- Let α=30∘ and β=60∘.
- The triangle has a horizontal base with these angles.
2. Set Up the Problem:
- The particle is projected from point A (one end of the base) and grazes the vertex C before landing at point B (the other end of the base).
- Let y be the height at vertex C and x be the horizontal distance from A to C.
3. Use the Tangent Function:
- For triangle ACO:
tanα=yx(1)
- For triangle COB:
tanβ=yr−x(2)
- Here, r is the total horizontal distance from A to B.
4. Combine the Equations:
- From equations (1) and (2):
y=xtanαandy=(r−x)tanβ
- Setting these equal gives:
xtanα=(r−x)tanβ
5. Rearranging the Equation:
- Rearranging gives:
xtanα+xtanβ=rtanβ
- Factoring out x:
x(tanα+tanβ)=rtanβ
- Thus:
x=rtanβtanα+tanβ(3)
6. Find tanθ:
- The equation of the trajectory of the projectile is given by:
y=xtanθ−gx22u2cos2θ
- At the vertex C, the height y can also be expressed in terms of x and tanθ:
y=xtanθ(4)
- Setting (3) and (4) equal gives:
rtanβtanα+tanβtanθ=rtanβtanα+tanβ
7. Substituting Values:
- We know:
tan30∘=1√3andtan60∘=√3
- Therefore:
tanθ=tan30∘+tan60∘=1√3+√3
8. Final Calculation:
- Combine the terms:
tanθ=1√3+√3=1+3√3=4√3
Conclusion:
Thus, the value of tanθ is:
tanθ=4√3
- Let α=30∘ and β=60∘.
- The triangle has a horizontal base with these angles.
- The particle is projected from point A (one end of the base) and grazes the vertex C before landing at point B (the other end of the base).
- Let y be the height at vertex C and x be the horizontal distance from A to C.
- For triangle ACO:
tanα=yx(1)
- For triangle COB:
tanβ=yr−x(2)
- Here, r is the total horizontal distance from A to B.
- From equations (1) and (2):
y=xtanαandy=(r−x)tanβ
- Setting these equal gives:
xtanα=(r−x)tanβ
- Rearranging gives:
xtanα+xtanβ=rtanβ
- Factoring out x:
x(tanα+tanβ)=rtanβ
- Thus:
x=rtanβtanα+tanβ(3)
- The equation of the trajectory of the projectile is given by:
y=xtanθ−gx22u2cos2θ
- At the vertex C, the height y can also be expressed in terms of x and tanθ:
y=xtanθ(4)
- Setting (3) and (4) equal gives:
rtanβtanα+tanβtanθ=rtanβtanα+tanβ
- We know:
tan30∘=1√3andtan60∘=√3
- Therefore:
tanθ=tan30∘+tan60∘=1√3+√3
- Combine the terms:
tanθ=1√3+√3=1+3√3=4√3
Thus, the value of tanθ is:
tanθ=4√3
Topper's Solved these Questions
Similar Questions
A particle is thrown over a triangle from one end of a horizontal base and after grazing the vertex falls on the other end of the base. If αandβ be the base angles and θ the angle of projection, prove that tanθ=tanα+tanβ .
View SolutionA particle is projected over a traingle from one end of a horizontal base and grazing the vertex falls on the other end of the base. If α and β be the base angles and θ the angle of projection, prove that tanθ=tanα+tanβ.
View Solution
Knowledge Check
A particle is thrown over a triangle from one end of horizontal base and grazing over the vertex falls on the other end of the base. If α,β are the base angles and θ the angle of projection, then
Atanθ=tanα−tanβBtanθ=tanβ−tanαCtanθ=tanα+tanβDNone of these
A particle is projected over a triangle from one extremity of its horizontal base. Grazing over the vertex, it falls on the other extremity of the base. If aandb are the base angles of the triangle and θ the angle of projection, prove that tanθ=tan∝+tanβ.
View SolutionA particle thrown over a triangle from one end of a horizontal base falls on the other end of the base after grazing the vertex. If αandβ are the base angles of triangle and angle of projection is θ, then prove that (tanθ=tanα+tanβ)
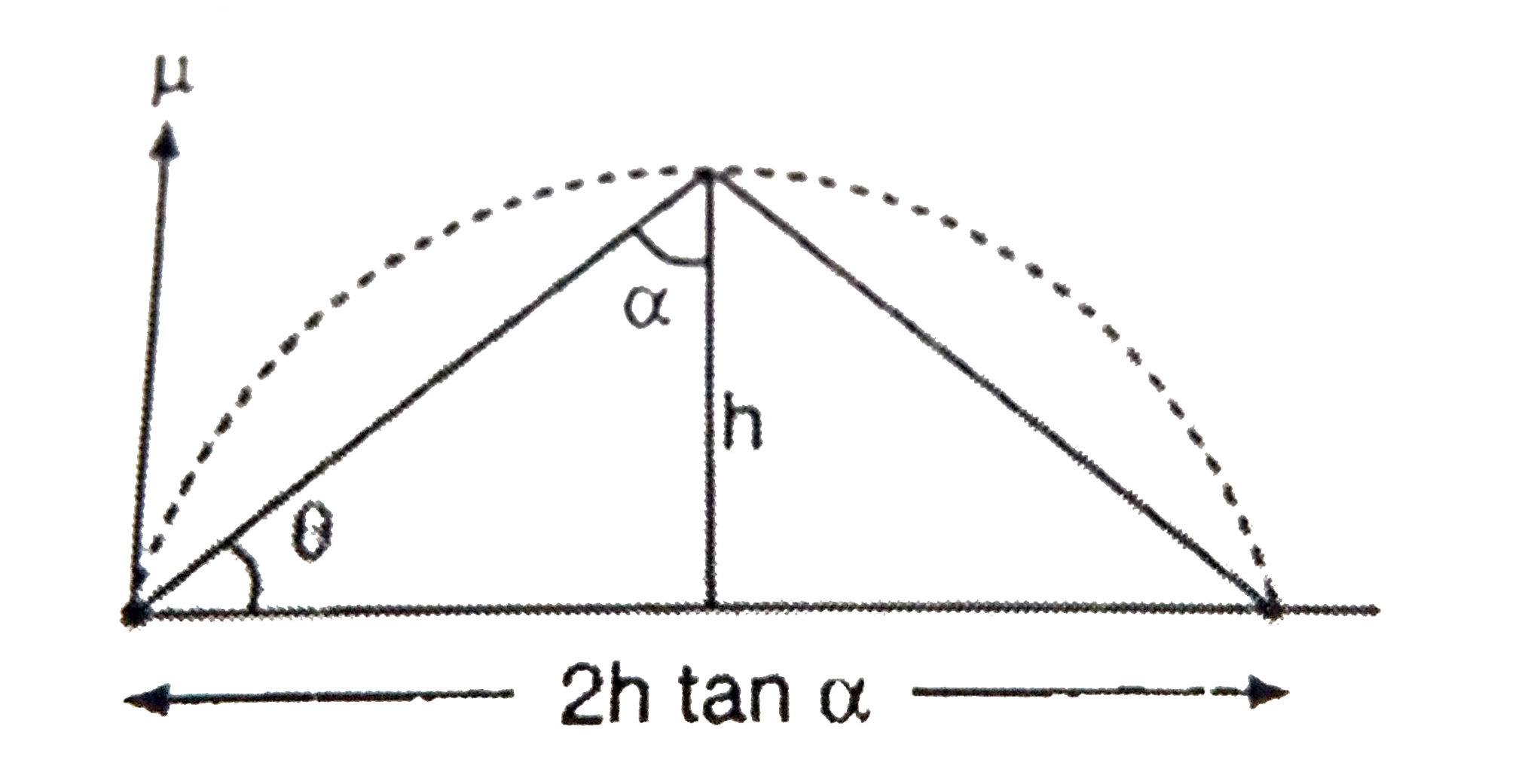
View SolutionA projectile is fired from the base of coneshaped hill. The projectile grazes the vertex and strikes the hill again at the base.If α be the half-angle of the cone, h its height, u the initial velocity of projection and θ angle of projection, then then θtanθis

View SolutionA projectile is fired from the base of cone shaped hill. The projectle grazes the vertex and strikes the hill again at the base. If α be the half-angle of the cone, h its height, u the initial velocity of projection and θ angle of projection, then tanθtanα is:
View SolutionThe vertex angle of an isosceles triangle is 80∘ , Find ot the measure of base angles .
View SolutionIn an isosceles triangle, if the vertex angle is twice the sum of the base angles, calculate the angles of the triangle.
Let each base angle be x in an isosceles △ABC
Then vertex angle be 2(x+x)=4x
Since sum of angles of a triangle is 1800
Hence,4x+x+x=1800
6x=1800
⇒x=300
Angles are 300,300,1200
View SolutionIn an isosceles triangle if the vertex angle is twice the sum of the base angles, calculate the angles of the triangle
View Solution
