A chain of mass M and length L is held vertical by fixing its upper end to a rigid support. The tension in the chain at a distance y from the rigid support is:
Mg
Mg(L−y)/L
MgL/(L−y)
Mgy/L
The correct Answer is:B
To find the tension in a chain of mass M and length L at a distance y from the rigid support, we can follow these steps:
Step 1: Understand the Setup
We have a vertical chain fixed at its upper end. The total length of the chain is L and its total mass is M. We need to determine the tension T at a point that is y distance from the upper support.
Step 2: Determine the Remaining Length of the Chain
At a distance y from the support, the length of the chain below this point is L−y.
Step 3: Calculate the Mass of the Remaining Chain
The mass per unit length of the chain can be calculated as:
Mass per unit length=ML
Thus, the mass of the remaining chain of length L−y is:
Mass of remaining chain=(ML)×(L−y)=M(L−y)L
Step 4: Calculate the Weight of the Remaining Chain
The weight of the remaining portion of the chain (which is acting downward) can be calculated using the formula:
Weight=mass×g=M(L−y)L×g
where g is the acceleration due to gravity.
Step 5: Set Up the Equation for Tension
At the point y, the tension T in the chain must balance the weight of the remaining chain below it. Therefore, we can write:
T=Weight of remaining chain
Substituting the expression for the weight:
T=M(L−y)L⋅g
Step 6: Final Expression for Tension
Thus, the final expression for the tension T at a distance y from the support is:
T=ML(L−y)g
This can also be expressed as:
T=mg(1−yL)
where m=M.
Summary
The tension in the chain at a distance y from the upper support is given by:
T=ML(L−y)g
or equivalently,
T=mg(1−yL)
---
We have a vertical chain fixed at its upper end. The total length of the chain is L and its total mass is M. We need to determine the tension T at a point that is y distance from the upper support.
At a distance y from the support, the length of the chain below this point is L−y.
The mass per unit length of the chain can be calculated as:
Mass per unit length=ML
Thus, the mass of the remaining chain of length L−y is:
Mass of remaining chain=(ML)×(L−y)=M(L−y)L
The weight of the remaining portion of the chain (which is acting downward) can be calculated using the formula:
Weight=mass×g=M(L−y)L×g
where g is the acceleration due to gravity.
At the point y, the tension T in the chain must balance the weight of the remaining chain below it. Therefore, we can write:
T=Weight of remaining chain
Substituting the expression for the weight:
T=M(L−y)L⋅g
Thus, the final expression for the tension T at a distance y from the support is:
T=ML(L−y)g
This can also be expressed as:
T=mg(1−yL)
where m=M.
The tension in the chain at a distance y from the upper support is given by:
T=ML(L−y)g
or equivalently,
T=mg(1−yL)
Topper's Solved these Questions
- BOOK - GRB PUBLICATIONCHAPTER - FORCE AND NEWTONS LAWS OF MOTIONEXERCISE - More than one choice is correct15videos
FORCE AND NEWTONS LAWS OF MOTION
- BOOK - GRB PUBLICATIONCHAPTER - FORCE AND NEWTONS LAWS OF MOTIONEXERCISE - Assertion- Reason4videos
FORCE AND NEWTONS LAWS OF MOTION
- BOOK - GRB PUBLICATIONCHAPTER - FORCE AND NEWTONS LAWS OF MOTIONEXERCISE - Problem for practice30videos
FORCE AND NEWTONS LAWS OF MOTION
- BOOK - GRB PUBLICATIONCHAPTER - BASIC MATHEMATICSEXERCISE - Problems For Practice35videos
BASIC MATHEMATICS
- BOOK - GRB PUBLICATIONCHAPTER - FRICTION AND CIRCULAR MOTION EXERCISE - Comprehension type11videos
FRICTION AND CIRCULAR MOTION
Similar Questions
A vertical hanging bar of length l and mass m per unit length carries a load of mass M at lower end, its upper end is clamped to a rigid support.The tensile stress a distance x from support is (A→ area of cross - section of bar)
View SolutionTension in the rope at the rigid support is (g=10m/s2)
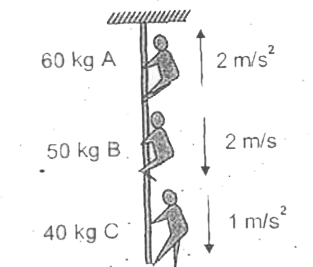 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A chain of mass M and length l is held vertically such that its bottom end just touches the surface of a horizontal table. The chain is released from rest. Assume that the portion of chain on the table does not form a heap. The momentum of the portion of the chain above the table after the top end of the chain falls down by a distance l8.
A314M√glB316M√glC716M√glD914M√gl - Question 2 - Select One
A partical of mass m is attached to a massless string of length l and is oscillating in a vrtical plane with the other end of the string fixed to a rigid support. The tension in the string at a certain instant is T=kmg .
Ak can never be geaater than 1Bk can never be less than 1Ck can never be equal than 1Dk can never be geaater than 3 - Question 3 - Select One
A uniform chain of mass M and length L is rotated in a horizontal circle about its one end at an angular frequency ω. The tension at its axis is
AMω2LBMω2L8CMω2L4DMω2L2
A very flexible uniform chain of mass M and length L is suspended vertically so that its lower and just touches the surface of a table. When the upper end of the chain is released it falls with each link coming to rest the instant it strikes the table. Find the force exerted by the chain on the table at the moment when y part of the chain has already rested on the table.
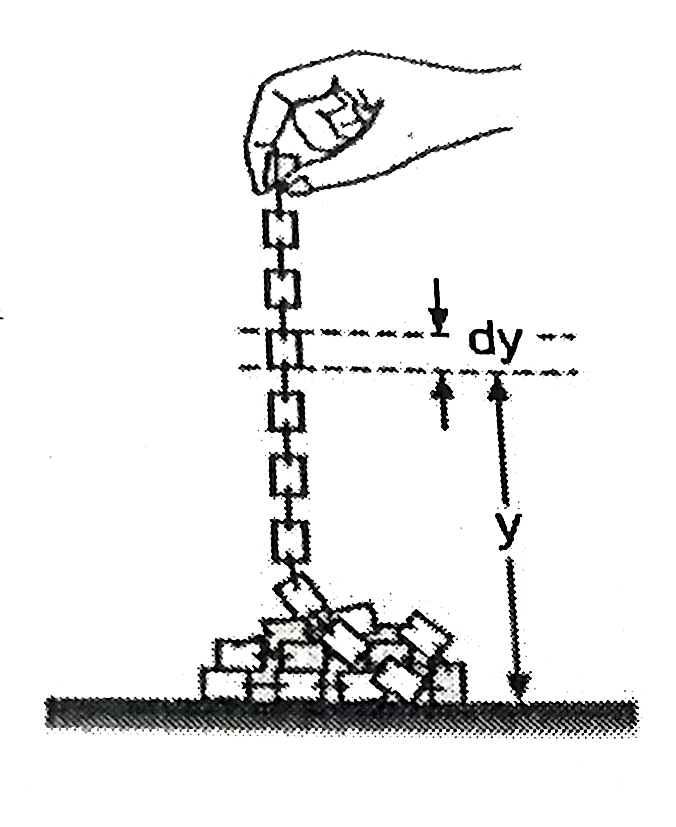 View Solution
View SolutionA chain with uniform mass per unit length lies in a vertical plane along the slope of a smooth hill. The two end of the chain are at same height. If the chain is released from this position find its acceleration.
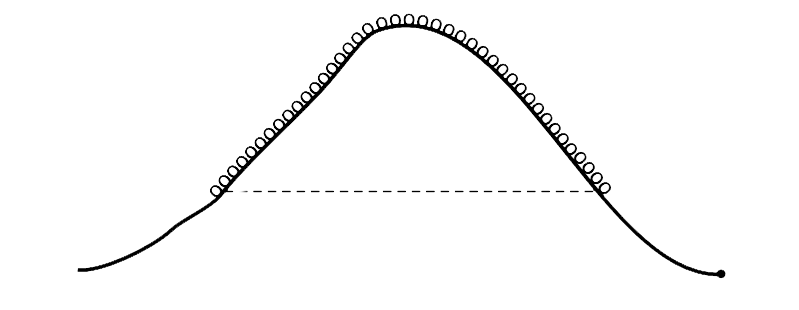 View Solution
View SolutionA stationlay unifrom sting of modlulus Y, density ρ and length 'I' is hanging from a rigid support.
The stress at a distance x from the point of tis suspension.View SolutionA block of mass m is pulled by a uniform chain of mass m tied to it by applying a force F at the other end of the chain. The tension at a point P which is at a distance of quarter of the length of the chain from the free end, will be
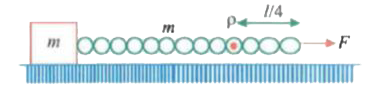 View Solution
View SolutionA block of mass m is pulled by a uniform chain of mass m tied to it by applying a force F at the other end of the chain.The tension at a point P which is at a distance of quarter of the length of the chain from the free end,will be
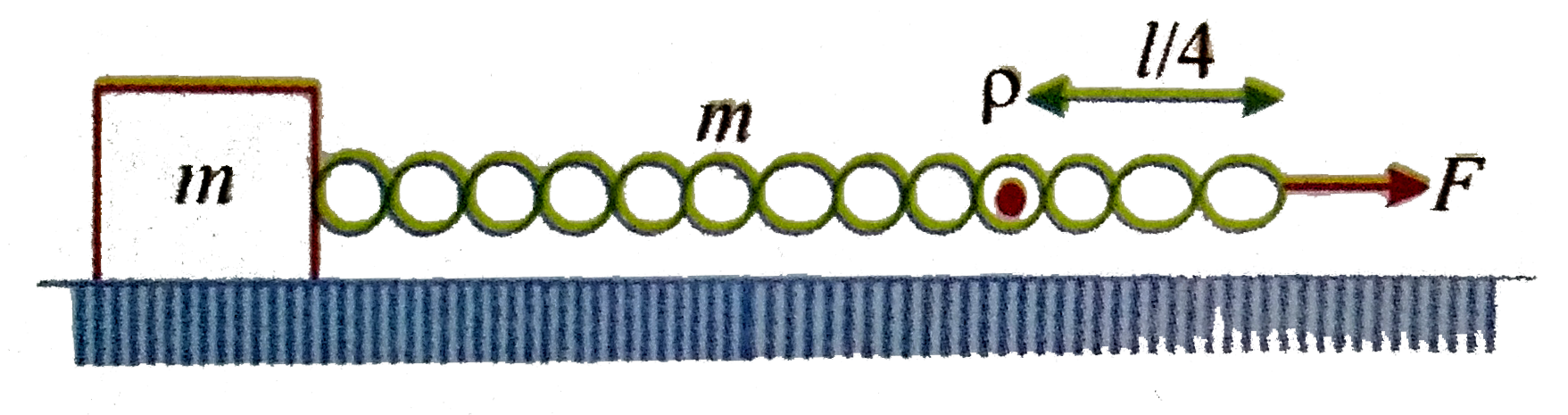 View Solution
View Solution

