ΔABD is a right triangle, right-angled at A and AC⊥BD. Prove that AB2=BC×BD.
To prove that AB2=BC×BD in triangle ΔABD where A is the right angle and AC⊥BD, we will use the properties of similar triangles.
1. Identify the triangles: We have two triangles, ΔABC and ΔABD. Note that A is the right angle in triangle ABD.
2. Establish angles: Since AC⊥BD, angle ACB is also a right angle. Therefore, we have:
∠BAD=∠ACB=90∘
3. Identify common angles: Both triangles ΔABC and ΔABD share angle ABC. Thus, we can say:
∠ABC=∠ABD
4. Use the angle sum property: Since the angles in a triangle sum up to 180∘, we can find the third angles:
∠BAC=∠ADB
5. Establish similarity: By the AA (Angle-Angle) criterion for similarity, we conclude that:
ΔABC∼ΔABD
6. Set up the proportionality: From the similarity of triangles, the sides are proportional:
ABBD=BCAB
7. Cross-multiply: To eliminate the fractions, we cross-multiply:
AB⋅AB=BC⋅BD
8. Simplify: This gives us:
AB2=BC⋅BD
9. Conclusion: Thus, we have proven that:
AB2=BC×BD
∠BAD=∠ACB=90∘
∠ABC=∠ABD
∠BAC=∠ADB
ΔABC∼ΔABD
ABBD=BCAB
AB⋅AB=BC⋅BD
AB2=BC⋅BD
AB2=BC×BD
Topper's Solved these Questions
Similar Questions
ΔABD is a right triangle, right-angled at A and AC⊥BD. Prove that AB2=BC×BD.
View SolutionABC is a right triangle right angled at C and AC = √3BC . Prove that ∠ABC=60∘ .
View SolutionABC is a right triangle right-angled at CandAC=√3BC . Prove that ∠ABC=600.
View SolutionIn the figure given below ABC is a triangle right angled at A and AC ⊥ BD . Show that
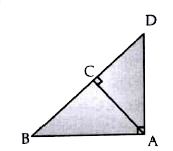
AB2=BC×BD
View SolutionABC is an isosceles right triangle, right-angled at C . Prove that: AB2=2AC2.
View SolutionABC is an isosceles right triangle right-angled at C . Prove that AB2=2AC2 .
View SolutionIn the figure given below ABC is a triangle right angled at A and AC ⊥ BD . Show that
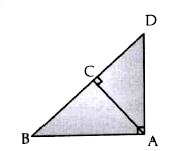
AD2=BD×DC
View SolutionIn the figure , ABD is a triangle right angled at A and AC ⊥ Bd . Show that
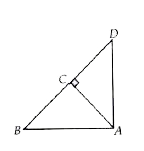
AD2=BD⋅CD
View SolutionABC is an isosceles triangle right-angled at C. Prove that AB2=2AC2.
View SolutionABC is a isosceles right angled triangle, right angled at C. prove that AB2=2AC2
View Solution
