A cubical block of wood, of length 10 cm, floats at the interface between oil of density 800kg/m3 and water. The lower surface of the block is 1.5 cm below the interface. If the depth of water is 10 cm below the interface and oil is upto 10 cm above the interface then the difference in pressure at the lower and the upper face of the wooden block is (Assume density of water ρ=1000kg/m3 ) and acceleration of gravity , g=10m/s2 )
850 Pa
780 Pa
800 Pa
830 Pa
The correct Answer is:D
Given, length of cubical block, l = 10 cm = 0.1 m
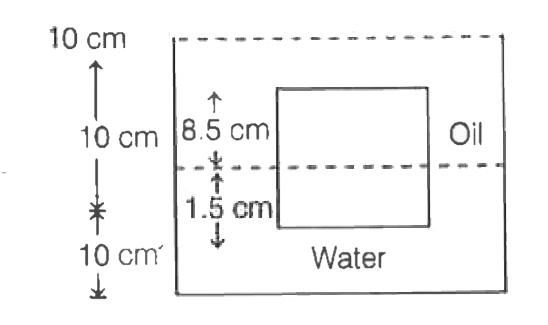 density of oil ρ0=800kg/m3
density of oil ρ0=800kg/m3
and density of water, ρw=103kg/m3
If V1 and V2 be the volume of wooden block in water and oil respectively, then
V1:V2=1.5:8.5
V1V2=1.58.5=3.17
∴V1=317V2 ...(i)
∴V1+V2=V where is the volume of the block From Eq. (1), we get
317V2+V2=V⇒V2=1720V
∴V1=320V
If ρ be the density of the block weight of block
∴ weight of oil displaced + weight of water displaced
VρG=V1ρωg+V2ρoilg
Vρg=320Vρwg+1720Vρoilg
ρ=320ρw+1720ρoil=320×10000+1720×800
=830kg/m3
∴ Pressure difference
p=ρgh=830×10×0.1=830Pa
Topper's Solved these Questions
Similar Questions
A cubical block of side 10 cm floats at the interface of an oil and water. The pressure above that of atmosphere at the lower face of the block is
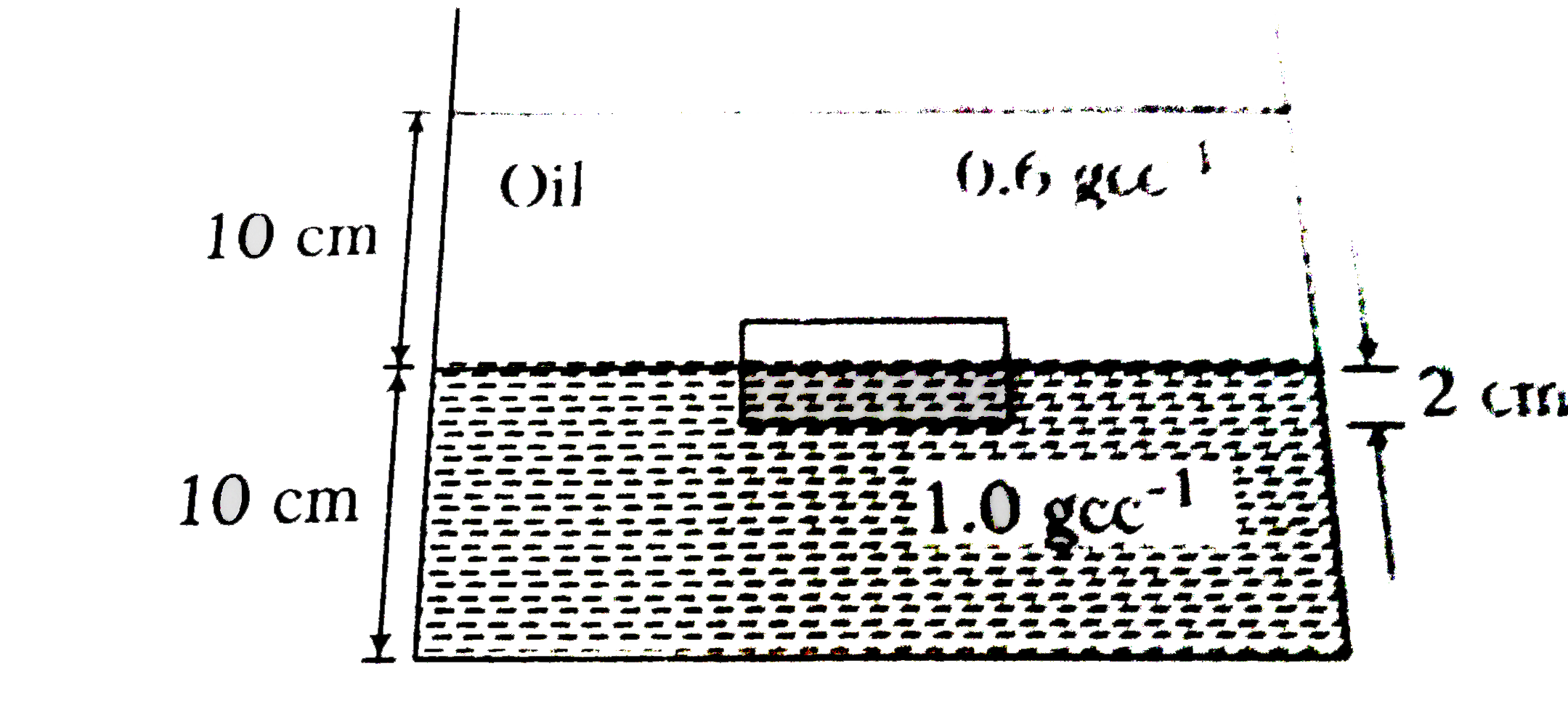
View SolutionA cubical block of wood 10cm along each side floats at the interface between an oil and water with its lowest surface 2cm below the interface. If the height of oil and water columns are 10cm each and ρoil=0.8gcc, find a the mass of the block, b. the liquid pressure at the lower surface of the block.
View Solution
Knowledge Check
A cubical block of wood 10 cm on a side floats at the interface between oil and water with its lower surface horizontal and 4 cm below the interface. The density of oil is 0.6gcm−3. The mass of block is
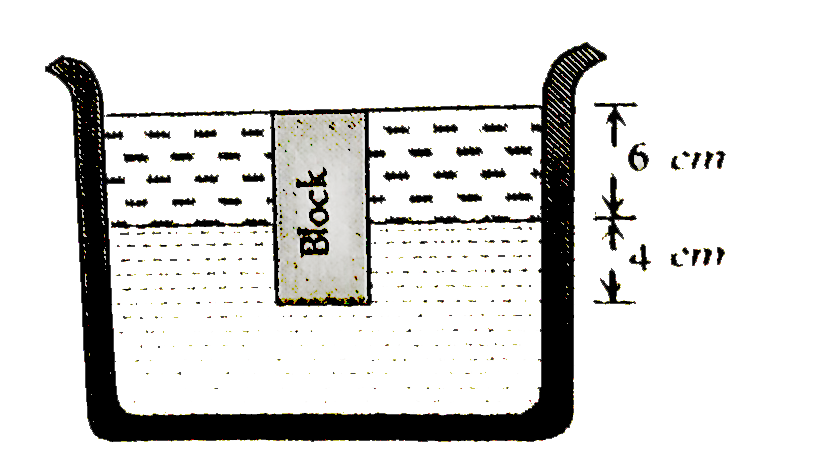 A706gB607gC760gD760g
A706gB607gC760gD760gA cubical block of side 10 cm floats at the interface of an oil and water. The pressure above that of atmosphere at the lower face of the block is
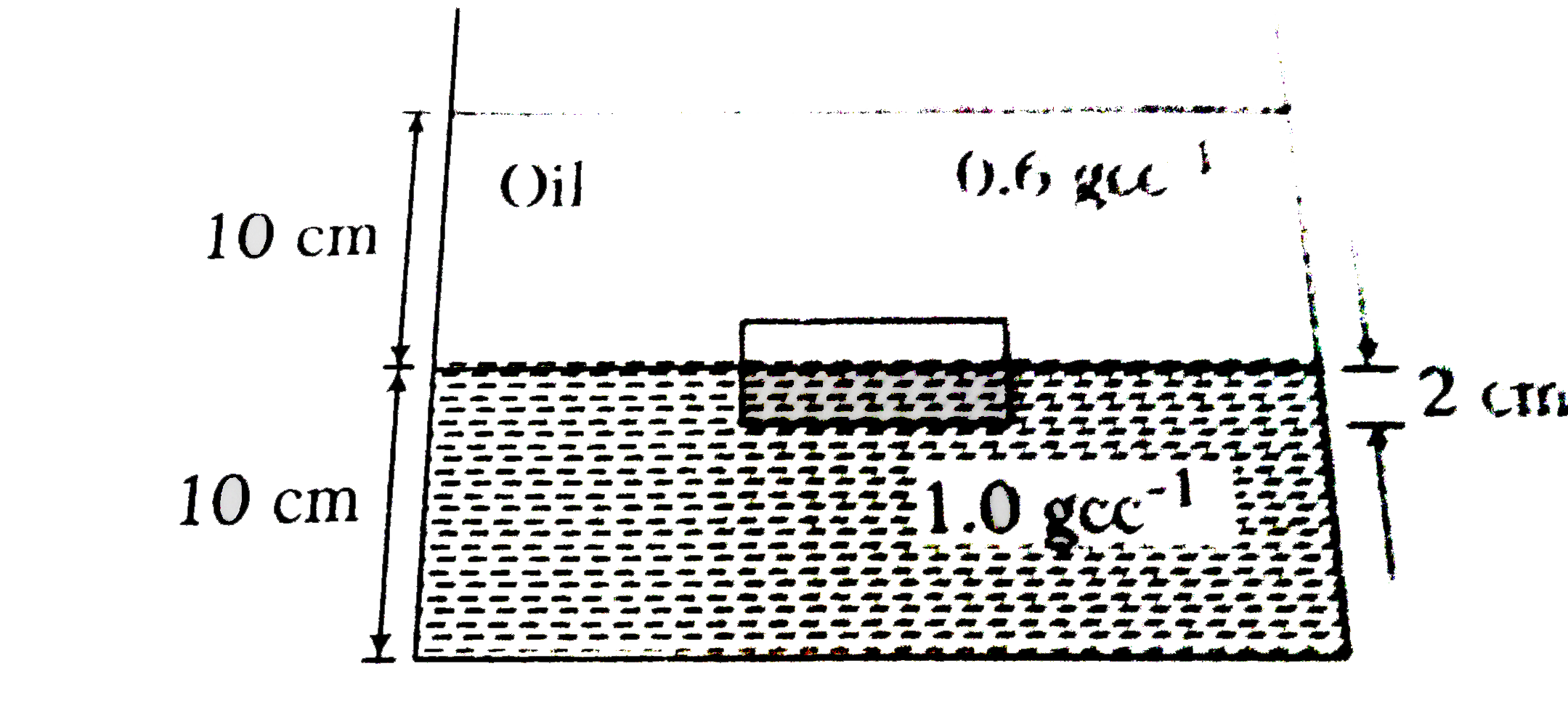 A200Nm−2B680Nm−2C400Nm−2D800Nm−2
A200Nm−2B680Nm−2C400Nm−2D800Nm−2A cubical block of wood 10cm on a side floats at the interface between oil and water, as in fig. with its lower face 2cm below the interface. The intensity of the oli is 0.6gcm−3. The mass of the block is 1kg
 A340gB680gC80gD10g
A340gB680gC80gD10g
A cubical block of wood 10cm along each side floats at the interface between an oil and water with its lowest surface 2cm below the interface. If the height of oil and water columns are 10cm each and ρoil=0.8gcc, find a the mass of the block, b. the liquid pressure at the lower surface of the block.
View SolutionA cubical block of side 10cm floats at the interface of an oil and water as shown in the figure. The density of oil is 0.6gcm−3 and the lower face of ice cube is 2cm below the interface. The pressure above that of the atomosphere at the lower face of the block is
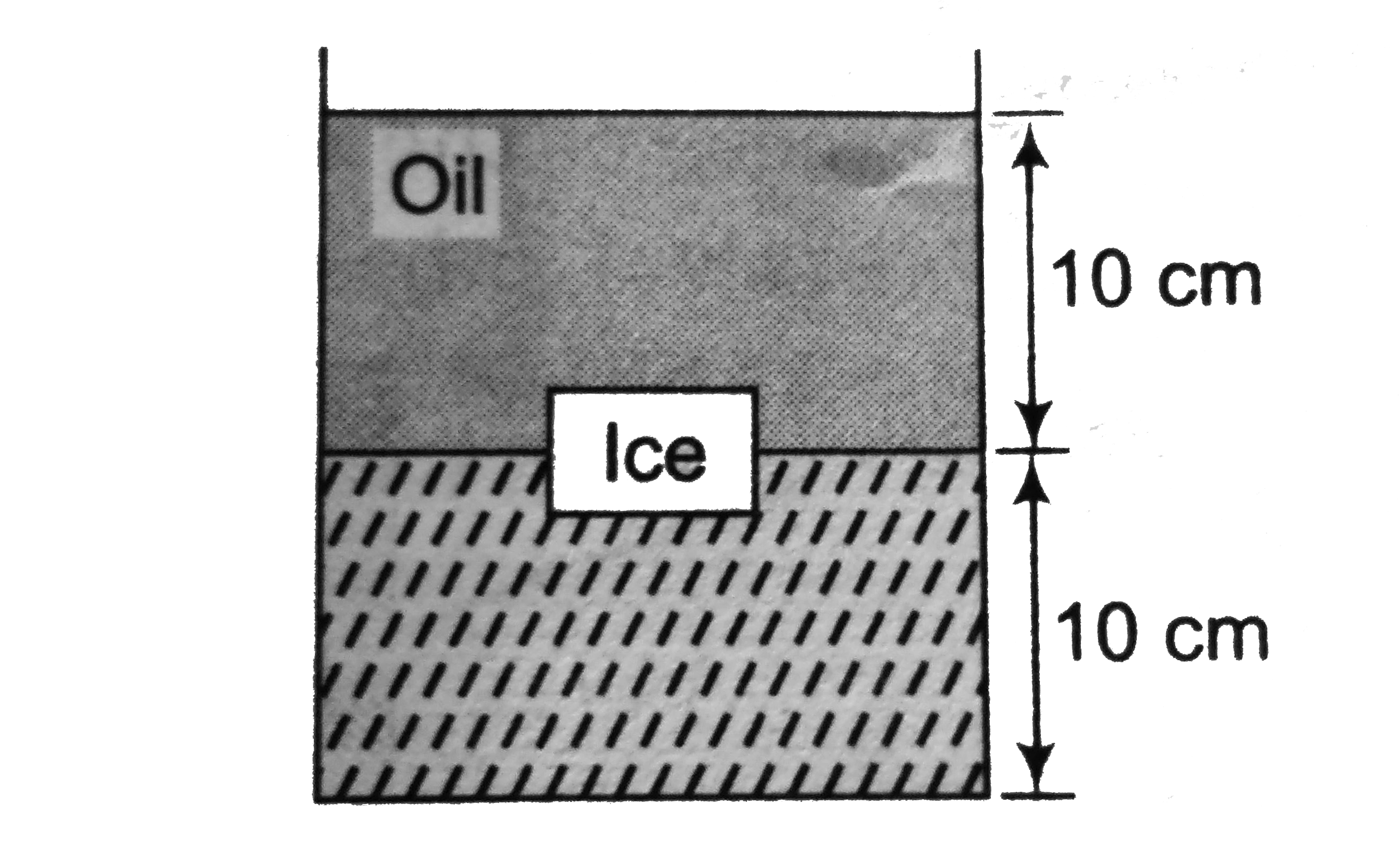
View SolutionA cubical block of wood of length 5 cm is kept on a table top. The density of the block is 800kg/m3 and g=9.8m/s2 . Find the pressure exerted by the wooden block on the table top.
View SolutionA block of wood of mass 24 kg floats in water. The volume of wood is 0.032 m. Find the volume of block below the surface of water
View SolutionA cubical block of side 10cm floats at the interface of an oil and water as shown in the figure. The density of oil is 0.6gcm−3 and the lower face of ice cube is 2cm below the interface. The pressure above that of the atomosphere at the lower face of the block is

View Solution
