Question

A uniform rod of length L is rotated in a horizontal plane about a vertical axis through one of its ends. The angular speed of rotation is $\omega$ . Find increase in length of the rod, if $\rho$ and Y are the density and Young's modulus of the rod respectively,

Options

A. `(rho omega^2 y)/(4L^2)`
B. `(4 rho omega^2 L^3)/(3Y)`
C. `(rho omega^2 L^3)/(3Y)`
D. `(rho omega^2 L^3)/(8Y)`

Answer

C

Explanation

Given,
length of uniform rod = L
angular speed = `omega`
and density of rod `= rho`
. When rod is rotated in a horizontal plane, centrifugal force is responsible for increasing the length of rod, which is also equal to stress.
If dl be the change in length of small element dx due to rotation, by application of force dF.

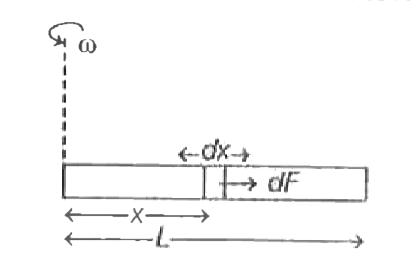
Young's modulus, ` Y = (" stress")/("strain") = (dF//A)/(dl//x)`
` rArr dl = (d.F.x)/(AY)`
` rArr dF = (AY dl)/(x) " " ...(i)`
Where, dF = centrifugal force due to mass of the element of length, dx
` dF = dm. x omega^2`
` dF = m/L . x d x omega^2 " " ....(ii) " " [ because " Change in mass dm "= m/L dx ]`
From Eqs. (l) and (ii), we get
` (AY dl)/(x) = m/L . x dx omega^2 `
` rArr dl = (mx^2 omega^2)/(LAY) .dx`
` rArr ` Total change in length
` l = int dl = int_(0)^(L) (mx^2 omega^2)/(LAY) dx`
` = (m omega^2 L^2)/(3AY) = (m)/(AL) . (omega^2 L^3)/(3Y) = (rho omega^2 L^3)/(3Y)`