Let →abe a unit vector and →ba non-zero vector not parallel to →a The angles of the triangle, two of whose sides are represented by √3(→a×→b)and(→b−(→a.→b)→a are
30∘,90∘,60∘
45∘,45∘,90∘
60∘,60∘,60∘
None of these
The correct Answer is:A
¯¯¯¯¯¯AB=√3(¯aׯb)
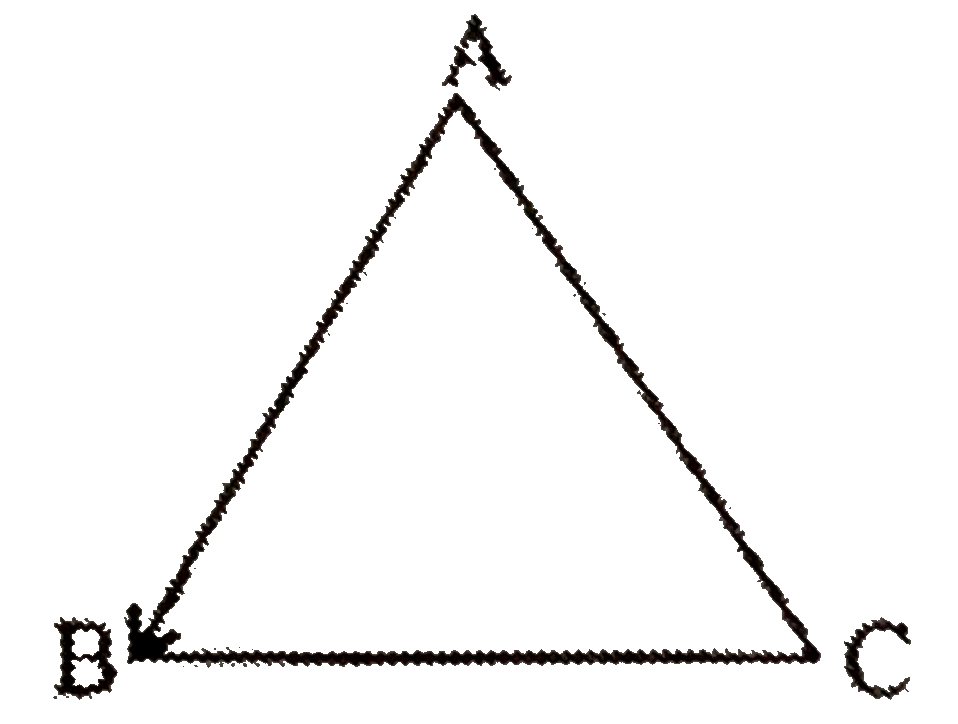
¯¯¯¯¯¯AC=→b−(→a.→b)→a , ∵|→a|=∣∣→b∣∣=1
⇒¯¯¯¯¯¯AB⊥¯¯¯¯¯¯AC
⇒∠BAC=90∘
Now, ∣∣∣−−→AB∣∣∣2=3∣∣→a×→b∣∣2
∣∣∣−−→AC∣∣∣2=|→a|2∣∣→b×→b∣∣2
⇒Ab=√3AC
⇒tanC=ABAC=√3
⇒∠C=60∘⇒∠A=30∘
Topper's Solved these Questions
Similar Questions
Knowledge Check
Angle between the vectors √3(→a×→b) and →b−(→a.→b)→a is
Aπ2B0Cπ4Dπ3If a parallelogram is formed with two sides represented by vector →a and →b, then →a+→b represents the
AMajor diagonal when the angle between vectors is acuteBMinor diagonal when the angle between vector is obtuseCBoth of the aboveDNone of the above
→aand→b are two non-collinear unit vectors →a,→b,x→a−y→b form a triangle.
View SolutionIf →a and →b are two non-collinear vectors having the same initial point. What are the vectors represented by →a+→b and →a−→b .
View SolutionArea of a triangle with adjacent sides determined by vectors →aand→b is 20. Then the area of the triangle with adjacent sides determined by vectors (2→a+3→b)and(→a−→b) is
View SolutionIf →a,→b,and→c are non-zero vectors such that →a.→b=→a.→c, then find the geometrical relation between the vectors.
View SolutionIf →a and →b are unit vectors find the angle between →a+→b and →a−→b.
View SolutionSolve the following equation for the vector →p;→p×→a+(→p.→b)→c=→b×→c where →a,→b,→c are non zero non coplanar vectors and →a is neither perpendicular to →b non to →c hence show that ⎛⎜ ⎜⎝→p×→a+[→a→b→c]→a⋅→c→c⎞⎟ ⎟⎠ is perpendicular →b−→c.
View Solution
