Given that a right angled trapezium has an inscribed circle. Prove that the length of the right angled leg is the Harmonic mean of the lengths of bases
Harmonic mean of length of bases
Geometric mean of length of bases
Arithmetic mean of length of bases
None of these
The correct Answer is:A
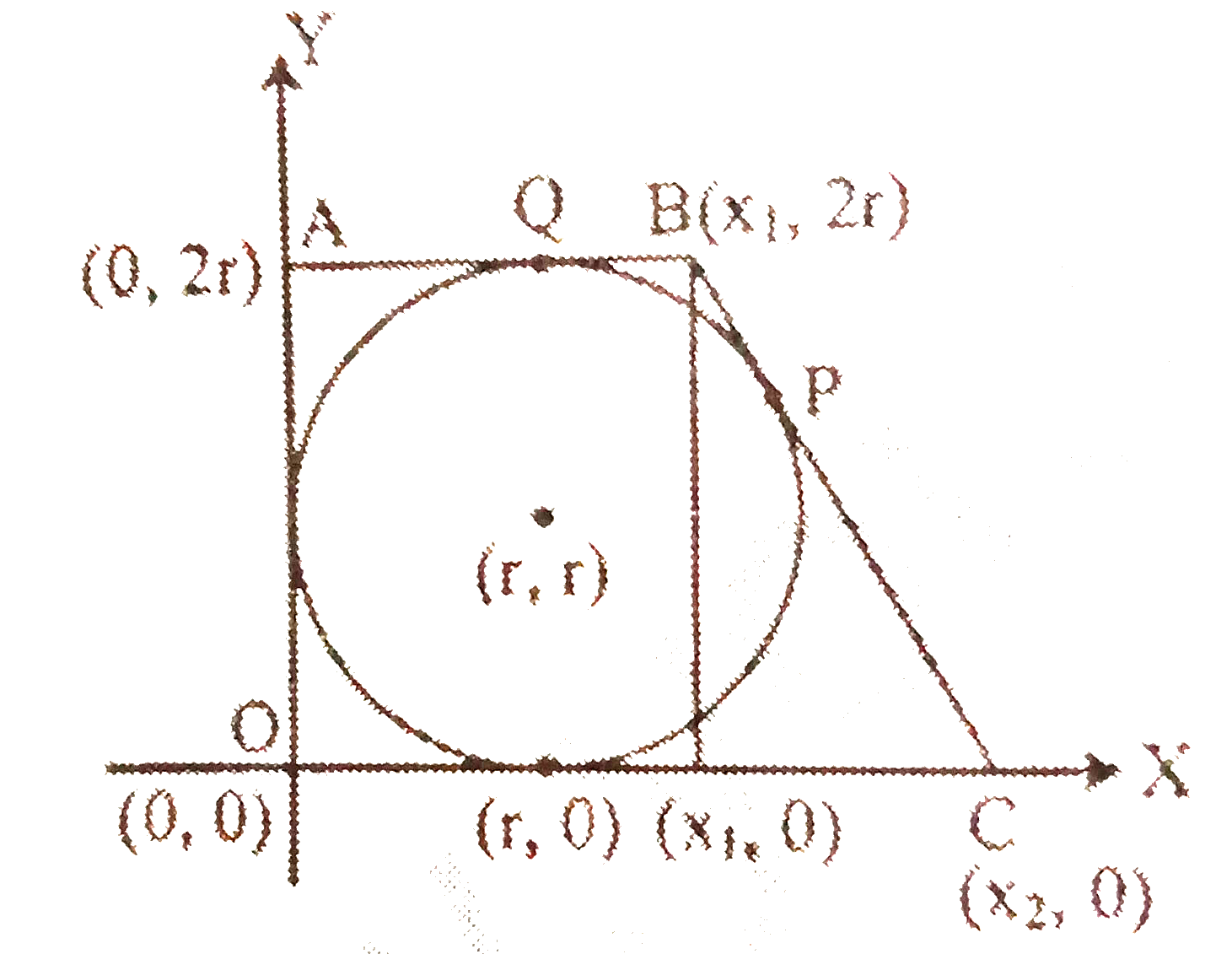
Let length of right angled leg
Equation of circle is
Length of tangent
Simliarly length of tangent
Now
Square
Topper's Solved these Questions
Similar Questions
A trapezium is inscribed in a circle. If one of its angles is double the other find the angles of the trapezium
View SolutionABC is a right angle triangle right angled at A. A circle is inscribed in it the length of the two sidescontaining right angle are 6 cm and 8 cm then the radius of the circle is
View Solution
Knowledge Check
Base of a right angled triangle is
A√(Hypotenous)2+(Perpendicular)2B√(Hypotenous)2−(Perpendicular)2C√(Perpendicular)2−(Hypotenous)2DNone of TheseIf the length of sides of right angled triangle are in AP, then their ratio is
A3:4:5B2:3:4C1:2:3Dnone of theseThe radius of a circle is 20% more than the height of a right-angled triangle. The base of the triangle is 36 cm. If the area of triangle and circle be equal, what will be the area of circle? (a) 72 cm2 (b) 128 cm2 (c) 144 cm2 (d) 216 cm2 (e) Cannot be determined
A72 sq cmB144 sq cmC216 sq cmD128 sq cm
A circle is inscribed in a trapezium in which one of the non-parallel sides is perpendicular to the two parallel sides. Then A) the diameter of the inscribed circle is the geometric mean of the lengths of the parallel sides B) the diameter of the inscribed circle is the harmonic mean of the lengths of the parallel sides C) the area of the trapezium is the area of the rectangle having lengths of its sides as the lengths of the parallel sides of the trapezium D) the area of the trapezium is half the area of the rectangle having lengths of its sides as the lengths of the parallel sides of the trapezium
View SolutionProve that the bisectors of the angles of a linear pair are at right angle.
View Solution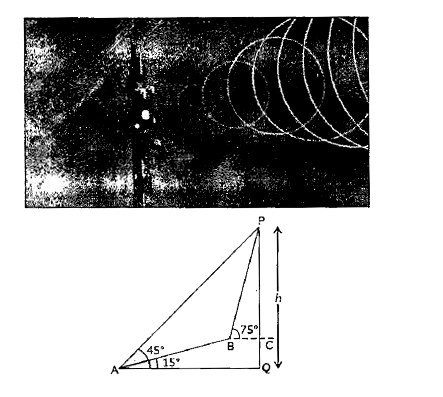
In the right angled triangle APQ, the length AP is :
View SolutionIf A be the area of a right triangle and b one of the sides containing the right angle, prove that the length of the altitude on the hypotenuse is 2AB√b4+4A2
View SolutionA right angled isosceles triangle is inscribed in a circle of radius r. What is the area of the remaining portion of the circle ?
View Solution
