A thin glass rod is bent into a semicircle of radius r. A charge +Q is uniformly distributed along the upper half and a charge -Q is uniformly distributed along the lower half, as shown in fig. The electric field E at P, the center of the semicircle, is

Qπ2ε0r2
2Qπ2ε0r2
4Qπ2ε0r2
Q4π2ε0r2
The correct Answer is:A
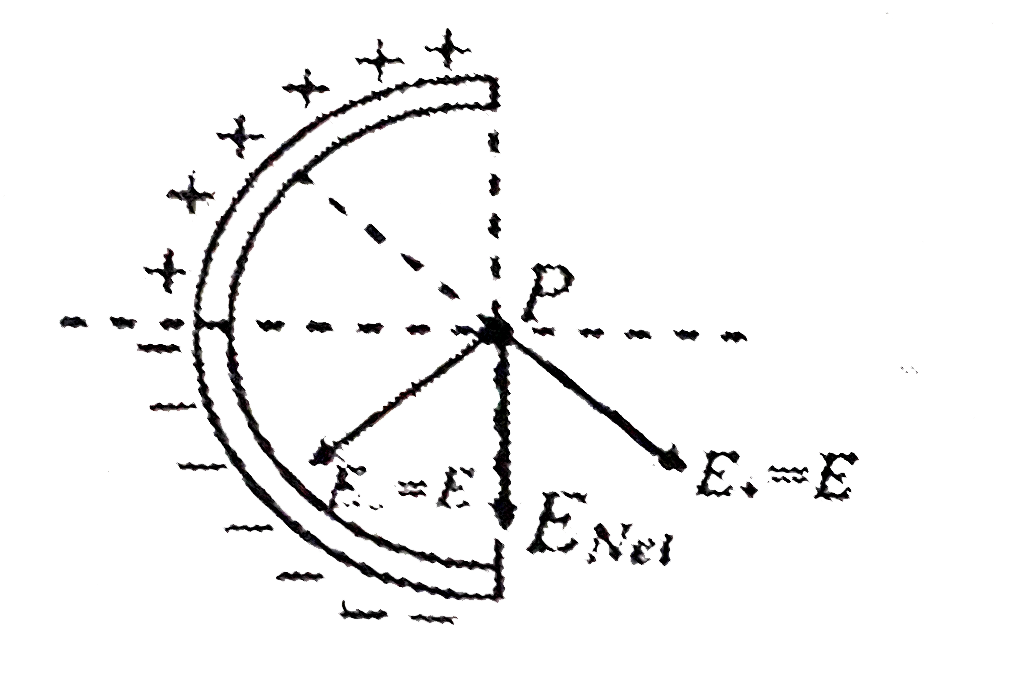
ENet=2Ecos45∘=√2E
=√22Kλrsin45∘
=2√2λ4πε0r×1√2=λ2πε0r
=Q2πε0r(πr2)=Qπ2ε0r2
Topper's Solved these Questions
Similar Questions
A wire of length l and charge q is bent in form of a semicircle. The charge is uniformly distributed over the length. The electric field at the centre of semicircle is
View SolutionCalculate potential on the axis of a ring due to charge Q uniformly distributed along the ring of readius R.
View Solution
Knowledge Check
A thin glass rod is bent into a semicircle of radius r. A charge +Q is uniformly distributed along the lower half, as shown in fig. The electric field E at P, the center of the semicircle, is
 AQπ2ε0r2B2Qπ2ε0r2C4Qπ2ε0r2DQ4π2ε0r2
AQπ2ε0r2B2Qπ2ε0r2C4Qπ2ε0r2DQ4π2ε0r2Charges q is uniformly distributed over a thin half ring of radius R. The electric field at the centre of the ring is
Aq2π2ε0R2Bq4π2ε0R2Cq4πε0R2Dq2πε0R2A charge Q is uniformly distributed only on the fourths portion of a ring of radius R. The elctric potential at centre of ring is
A3Q4πε0RBQ4πε0RC3Q2πε0RD6Q4πε0R
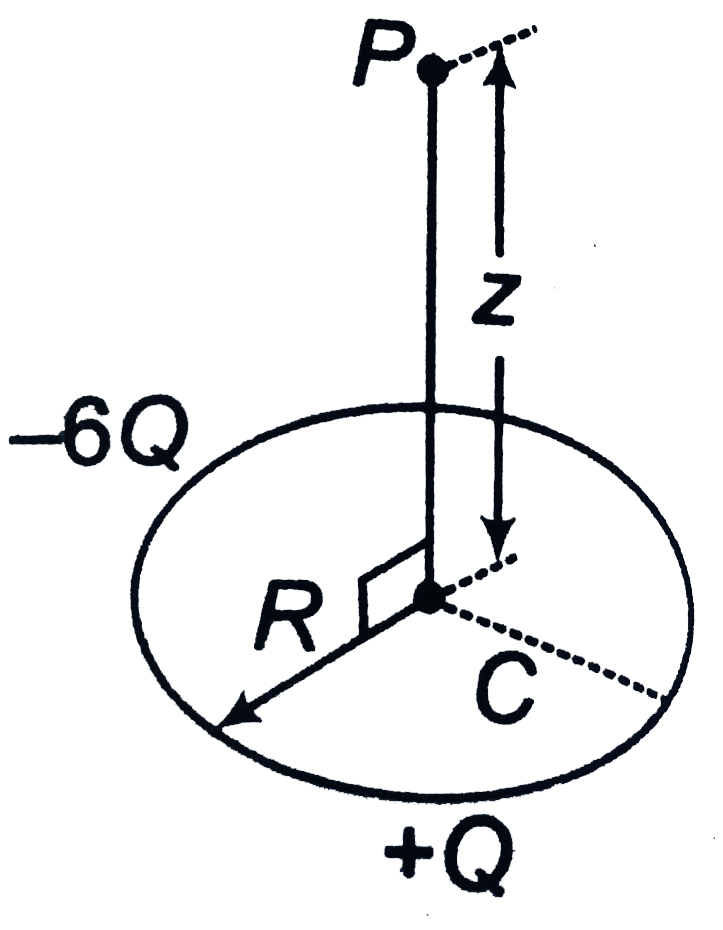
A plastic rod has been formed into a circle of radius R. It has a positive charge +Q uniformly distributed along one-quarter of its circumference and a negative charge of −6Q uniformly distributed along the rest of the circumference (figure). With V = 0 at infinity, what is the electric potential −6Q
(a) at the centre C of the circle and
(b) at point P, which is on the central axis of the circle at distance z from the centre?
View SolutionCalculate potential on the axis of a disc of radius R due to a charge Q uniformly distributed on its surface.
View SolutionA positive charge Q is uniformly distributed across a rod of length L. The rod is then bent in the form of a semicircle. What will be the electric field intensity at the centre of curvature of the semicircle thus formed?
View SolutionThere is a hemispherical shell having charge Q uniformly distributed on its surface. Radius of the shell is R. Find electric potential and field at the centre (of the sphere).
View SolutionA spherical shell of radius R has a uniformly distributed charge ,then electric field varies as
View Solution
