The coordinates of points in the table

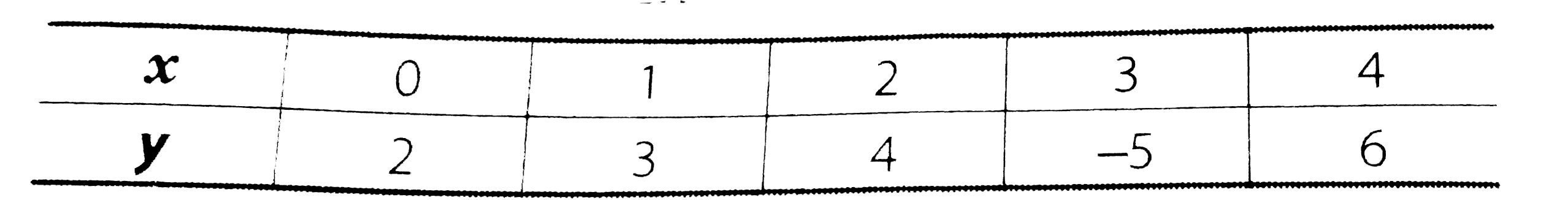
represent some of the solutions of the equation x-y+2=0.

Video Solution

Text Solution

Verified by Experts

False
The coordinates of points are $(0, 2),) (1, 3), (2, 4), (3, - 5) and (4, 6)$ .
Given equation is x-y+2=0
At point (0,2) $0 - 2 + 2 = 0 \Rightarrow 0 = 0$ , it satisfies.
At point (1,3), $1 - 3 + 2 = 3 - 3 = 0 \Rightarrow 0 = 0$ , it satisfies.
At point (2,4), $2 - 4 + 2 = 4 - 4 = 0 \Rightarrow 0 = 0$ , it satisfies.
At point $(3, - 5), 3 - (- 5) + 2 = 3 + 5 + 2 = 10 \neq 0$ , it does not satisfy.
At point $(4, 6), 4 - 6 + 2 = 6 - 6 = 0 \Rightarrow 0 = 0$ , it satsisfies.
Hence, point (3,-5) does not satisfy the equation.

Updated on:21/07/2023

The coordinates of the point where origin is shifted is (-1,2) so that the equation $2 x^{2} + y^{2} - 4 x + 4 y = 0$ become?
A $X^{2} + 2 Y^{2} = 6$
B $2 X^{2} + y^{2} = 6$
C $2 X^{2} + Y^{2} = 4$
D $X^{2} + 2 Y^{2} = 4$
The coordinates of the centre and radius of the circle represented by the equation
$(3 - 2 λ) x^{2} + λ y^{2} - 4 x + 2 y - 4 = 0$ are
A(2, 1), 3
B(-2, 1), 3
C(2, 1), 3
D(2, -1), 1