Two lines are respectively perpendicular to two parallel lines. Show that they are parallel to each other.
Given Two lines m and n are parallal and another two lines p and q are respectively perpendicular to m and n.
i.e., p⊥m,p⊥n,q⊥m,q⊥n
To prove p||q
Proof Since, m||n and p is perpendicular to m and n .
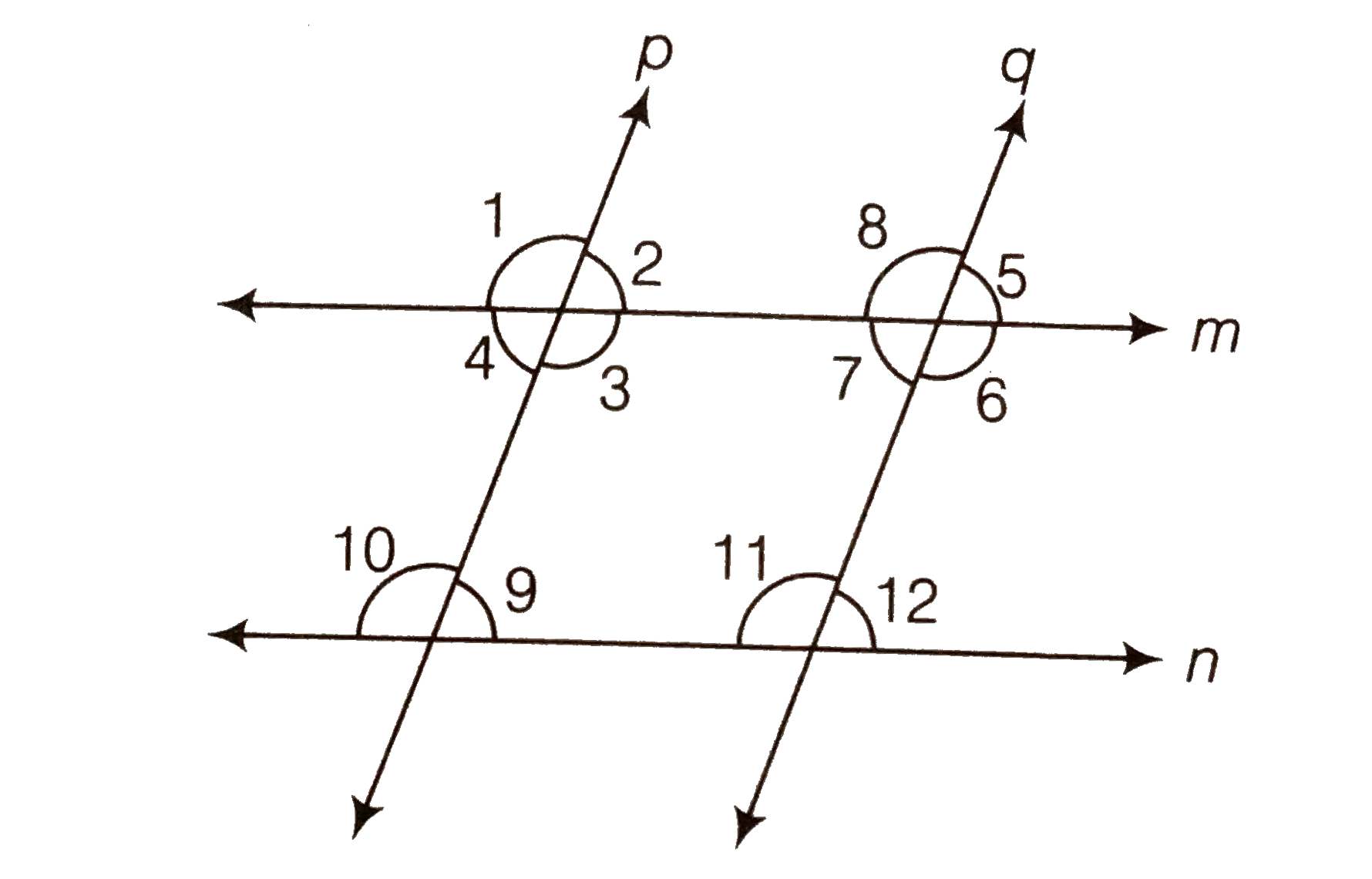
∴ ∠1=∠10=90∘ [corresponding angles]
Simillarly , ∠2=∠9=90∘ [corresponding angles] [∵p⊥mandp⊥n]
∴ ∠4=∠9=90∘and∠3=∠10=90∘ [alternate interior angles]
Similarly, if m||n and q is perpendicular to m and n .
Then ∠7=90∘and∠11=90∘
Now, ∠3+∠7=∠90∘+∠90∘=∠180∘
So, sum of two interior angles is supplementary.
we know that, if a transversal intersects two lines such that a pair of interior angles on the same side of the transversal is supplementary, then the two lines are parallel.
Hence, p||q.
Topper's Solved these Questions
Similar Questions
Transversal to two parallel lines:
View SolutionWhich of the following statements are true (T) and which are false (F)? Give reasons. (i)If two lines are intersected by a transversal, then corresponding angles are equal. (ii)If two parallel lines are intersected by a transversal, then alternate interior angles are equal. (iii)Two lines perpendicular to the same line are perpendicular to each other. (iv)Two lines parallel to the same line are parallel to each other. (v)If two parallel lines are intersected by a transversal, then the interior angle on the same side of the transversal are equal.
View SolutionProve that two lines that are respectively perpendicular to two intersecting lines intersect each other .
View SolutionProve that two lines that are respectively perpendicular to two intersecting lines intersect each other.
View SolutionProve that two lines perpendicular to the same line are parallel to each other.
View Solutionprove that two lines in a plan which ar perpandicular to the same given line the plan ar parallel to each other
View SolutionTwo straight lines are cut by a transversal. If the bisectors of a pair of co-interior angles are perpendicular to each other , prove the two straight lines are parallel to each other.
View SolutionTheorem 6.6 : Lines which are parallel to the same line are parallel to each other.
View SolutionFor what value of x will the lines l and m be parallel to each other ?
 View Solution
View SolutionFor what value of x will the lines l and m be parallel to each other ?
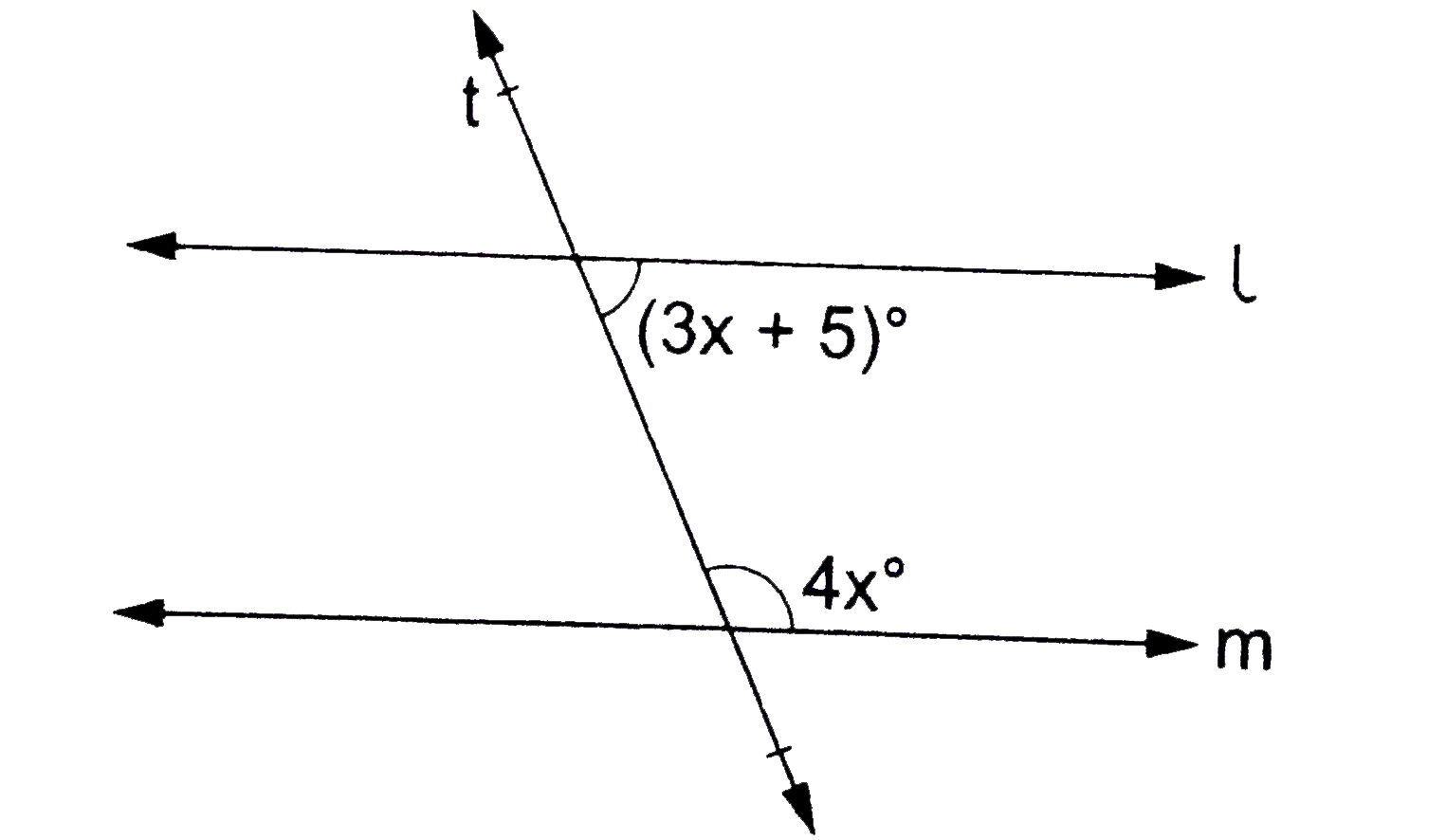 View Solution
View Solution

