A Δ ABC is right angled at A. L is a point on BC such that AL⊥ BC. Prove that ∠BAL=∠ACB.
To prove that ∠BAL = ∠ACB in triangle ABC, where triangle ABC is right-angled at A and AL is perpendicular to BC, we can follow these steps:
1. Identify the Given Information:
- Triangle ABC is right-angled at A.
- AL is perpendicular to BC, meaning ∠ALB = 90°.
2. Label the Angles:
- Let ∠BAL = x.
- Since triangle ABC is right-angled at A, we have ∠CAB = 90°.
3. Use the Angle Sum Property:
- In triangle ABC, the sum of the angles is 180°.
- Therefore, we can write:
∠CAB+∠ABC+∠ACB=180°
- Substituting ∠CAB = 90°:
90°+∠ABC+∠ACB=180°
- This simplifies to:
∠ABC+∠ACB=90°
4. Express ∠ACB in terms of x:
- Since AL is perpendicular to BC, we have:
∠BAL+∠ALC=90°
- Substituting ∠BAL = x:
x+∠ALC=90°
- This implies:
∠ALC=90°−x
5. Consider Triangle ALC:
- In triangle ALC, applying the angle sum property:
∠ALC+∠ACB+∠ALB=180°
- We know ∠ALB = 90°, so:
(90°−x)+∠ACB+90°=180°
- Simplifying gives:
180°−x+∠ACB=180°
- Thus, we find:
∠ACB=x
6. Conclusion:
- Since we have shown that ∠BAL = x and ∠ACB = x, we conclude:
∠BAL=∠ACB
- Therefore, we have proved that ∠BAL = ∠ACB.
- Triangle ABC is right-angled at A.
- AL is perpendicular to BC, meaning ∠ALB = 90°.
- Let ∠BAL = x.
- Since triangle ABC is right-angled at A, we have ∠CAB = 90°.
- In triangle ABC, the sum of the angles is 180°.
- Therefore, we can write:
∠CAB+∠ABC+∠ACB=180°
- Substituting ∠CAB = 90°:
90°+∠ABC+∠ACB=180°
- This simplifies to:
∠ABC+∠ACB=90°
- Since AL is perpendicular to BC, we have:
∠BAL+∠ALC=90°
- Substituting ∠BAL = x:
x+∠ALC=90°
- This implies:
∠ALC=90°−x
- In triangle ALC, applying the angle sum property:
∠ALC+∠ACB+∠ALB=180°
- We know ∠ALB = 90°, so:
(90°−x)+∠ACB+90°=180°
- Simplifying gives:
180°−x+∠ACB=180°
- Thus, we find:
∠ACB=x
- Since we have shown that ∠BAL = x and ∠ACB = x, we conclude:
∠BAL=∠ACB
- Therefore, we have proved that ∠BAL = ∠ACB.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
If ABC is an equilateral triangle and D is a point on BC such that AD⊥BC, then
AAB:BD=1:1BAB:BD=1:2CAB:BD=2:1DAB:BD=3:2 - Question 2 - Select One
ΔABC is right angled at A and AD⊥BC. Then BDDC=
A(ABAC)2BABACC(ABAD)2DABAD - Question 3 - Select One
ABC is a triangle right- angled at B and D is a point on BC produced (BD>BC), such that BD=2DC. Which one of the following is correct?
AAC2=AD2−3CD2BAC2=AD2−2CD2CAC2=AD2−4CD2DAC2=AD2−5CD2
A triangle ABC is right angles at A.AL is drawn perpendicular to BC . Prove that ∠BAL= ∠ACB
View SolutionABC is a triangle in which AB=ACandD is a point on AC such that BC2=AC×CD.Prove that BD=BC.
View SolutionIn a right tringle ABC, right-angled at B, D is a point on hypotenuse such that BD⊥AC. If DP⊥ABandDQ⊥BC then prove that
(a)DQ2=DP⋅QC DP2=DQ⋅AP.
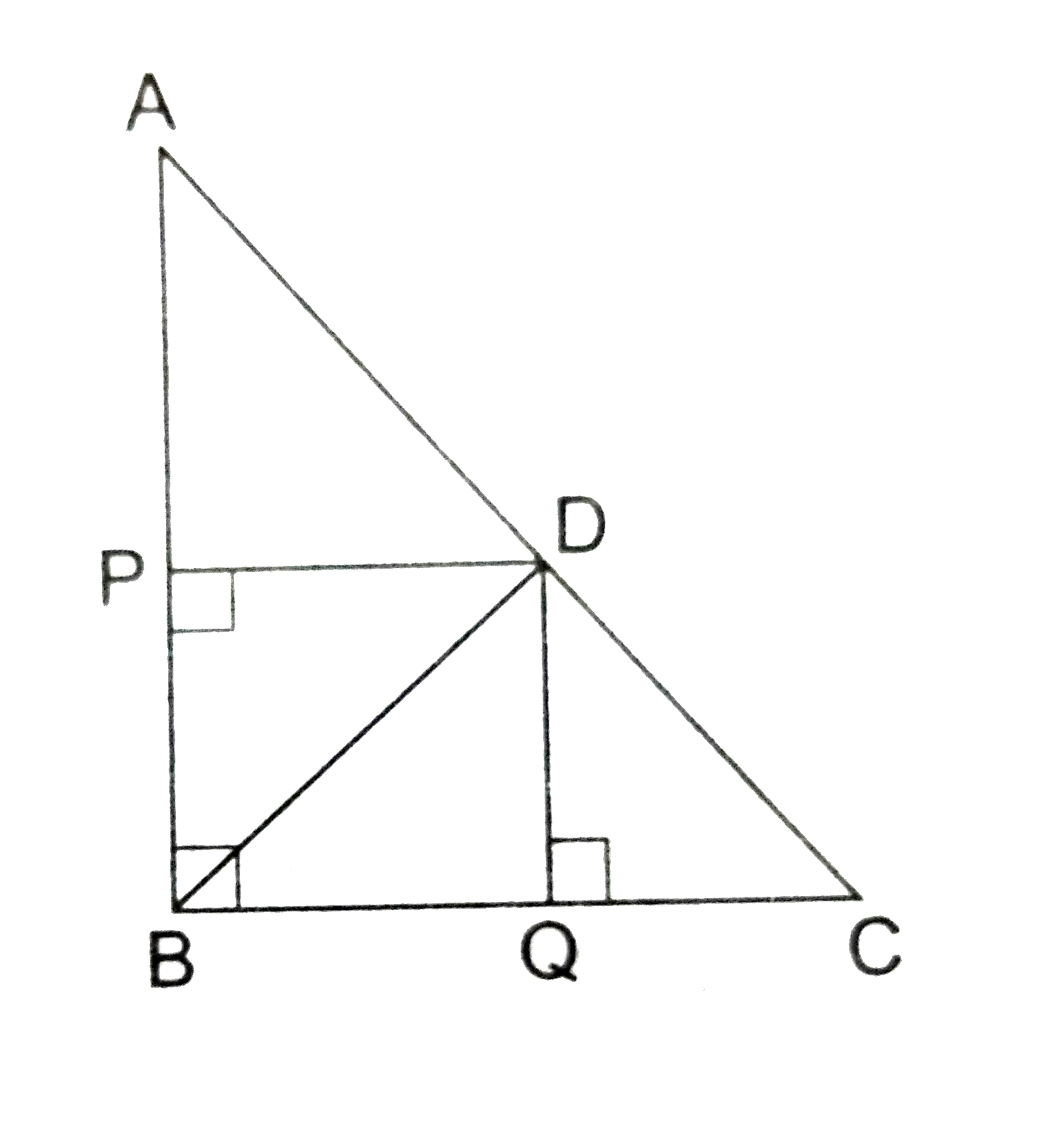 View Solution
View SolutionABC is a triangle right-angled at B and D is a point on BC produced (BD > BC), such that BD = 2 DC. Which one of the following is correct?
View SolutionTriangle ABC is right angle at A. The points P and Q are on hypotenuse BC such that BP=PQ=QC.if AP=3andAQ=4, then length BC is equal to
View Solution

