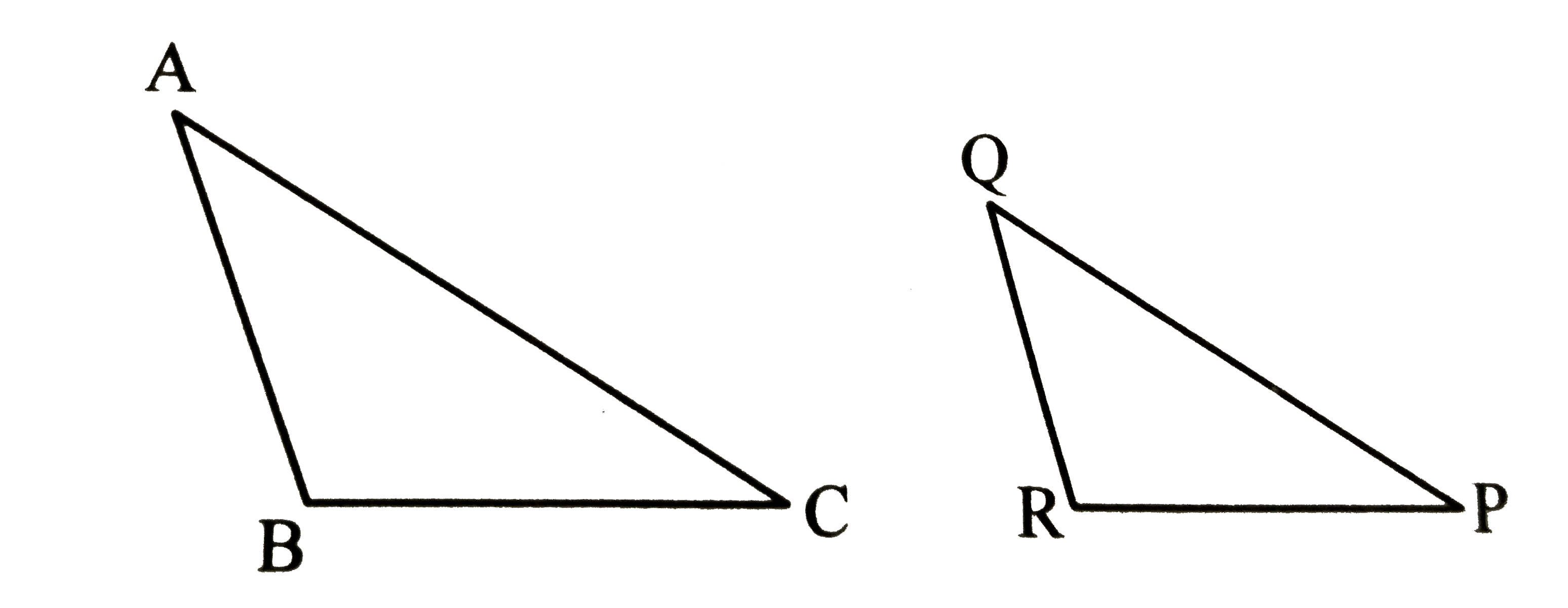
If in two ΔABC and ΔPQR, ABQR=BCPR=CAPQ, then
ΔPQR~ΔCAB
ΔPQR~ΔABC
ΔCBA~ΔPQR
ΔBCA~ΔPQR
The correct Answer is:A
To solve the problem, we need to show that the triangles ABC and PQR are similar based on the given ratios of their corresponding sides.
1. Understanding the Given Ratios:
We are given that:
ABQR=BCPR=CAPQ
This means that the ratios of the lengths of the corresponding sides of triangles ABC and PQR are equal.
Hint: Look for the relationship between the sides of the triangles to establish a similarity condition.
2. Identifying Corresponding Sides:
From the ratios, we can identify:
- Side AB corresponds to side QR
- Side BC corresponds to side PR
- Side CA corresponds to side PQ
Hint: Make sure to match each side of triangle ABC with the correct side of triangle PQR.
3. Using the Side-Angle-Side (SAS) Similarity Criterion:
Since the ratios of the corresponding sides are equal, we can apply the Side-Side-Side (SSS) similarity criterion. According to this criterion, if the ratios of the lengths of the corresponding sides of two triangles are equal, then the triangles are similar.
Hint: Remember that for triangles to be similar, the corresponding angles must also be equal.
4. Concluding Similarity:
Therefore, we conclude that:
ΔABC∼ΔPQR
This means triangle ABC is similar to triangle PQR.
Hint: Write down the similarity statement clearly to avoid confusion.
5. Identifying Other Corresponding Triangles:
Based on the similarity established, we can also state:
- Triangle BCA is similar to triangle RQP
- Triangle CAB is similar to triangle PQR
Hint: Similarity can be transitive; if one triangle is similar to another, you can find other similar pairs.
Final Answer:
Thus, we can conclude that triangles ABC and PQR are similar, and the correct option is that triangle ABC is similar to triangle PQR.
We are given that:
ABQR=BCPR=CAPQ
This means that the ratios of the lengths of the corresponding sides of triangles ABC and PQR are equal.
From the ratios, we can identify:
- Side AB corresponds to side QR
- Side BC corresponds to side PR
- Side CA corresponds to side PQ
Since the ratios of the corresponding sides are equal, we can apply the Side-Side-Side (SSS) similarity criterion. According to this criterion, if the ratios of the lengths of the corresponding sides of two triangles are equal, then the triangles are similar.
Therefore, we conclude that:
ΔABC∼ΔPQR
This means triangle ABC is similar to triangle PQR.
Based on the similarity established, we can also state:
- Triangle BCA is similar to triangle RQP
- Triangle CAB is similar to triangle PQR
Thus, we can conclude that triangles ABC and PQR are similar, and the correct option is that triangle ABC is similar to triangle PQR.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
If in two triangles ABC and PQR, ABQR=BCPR , then which of the following is true?
AΔBCA~ΔPQRBΔPQR~ΔCABCΔPQR~ΔABCDΔCBA~ΔPOR - Question 2 - Select One
If in ΔPQRandΔXYZ,PQXY=QRXZ then the triangles will be similar, when
A∠P≅∠XB∠R≅∠YC∠Q≅∠YD∠Q≅∠X - Question 3 - Select One
If ΔABC~ΔPQR with BCQR=13,then ar(ΔPRQ)ar(ΔBCA) is equal to
A91B31C13D19
In ΔABC and ΔPQR , in a one-to-one correspondance ABQR=BCPR=CAPQ then……
 View Solution
View SolutionWhich of the following pairs of triangles are congruent ? Also state the condition of congruency in each case :
(iv) In ΔABC and ΔDEF,AB=EF,BC=DF and ∠B=∠F
(v) In ΔABC and ΔPQR,AB=QR,AC=PR and ∠B=∠R
(vi) In ΔABC and ΔPQR,∠A=∠P,AC=PR and AB=PQ
(vi) In ΔABC and ΔPQR,AB=QR,∠A=∠Q and AC=QP.View SolutionΔABC~ΔPQR. If AM and PN are altitudes of ΔABCandΔPQR respectively and AB2:
PQ2=4:9 then AM:PN=View SolutionIf ΔABC~ΔPQR,A(ΔABC)=80,A(ΔPQR)=125, then fill in the blanks: A(ΔABC)A(Δ………..)=80125∴ABPQ=□□
View SolutionAssertion : In ΔABCandΔPQR,AB=PQAC=PRand∠BAC=∠QPR
∴ΔABC≅ΔPQR
Reason : Both the triangles are congruent by SSS congruence.View Solution