Ii ΔPQR, PD⊥QR such that D lies on QR, if PQ=a,PR=b,QD=c and DR=d, then prove that (a+b)(a-b)=(c+d)(c-d).
Video Solution
Text Solution
Given In ΔPQR,PD⊥QR,PQ=a,PR=b,QD=c and DR=d
To prove (a+b)(a-b)=(c+d)(c-d)
Proof In right angled ΔPDQ
PQ2=PD2+QD2 [by pythagoras theorem]
⇒a2=PD2+c2
⇒PD2=a2−c2
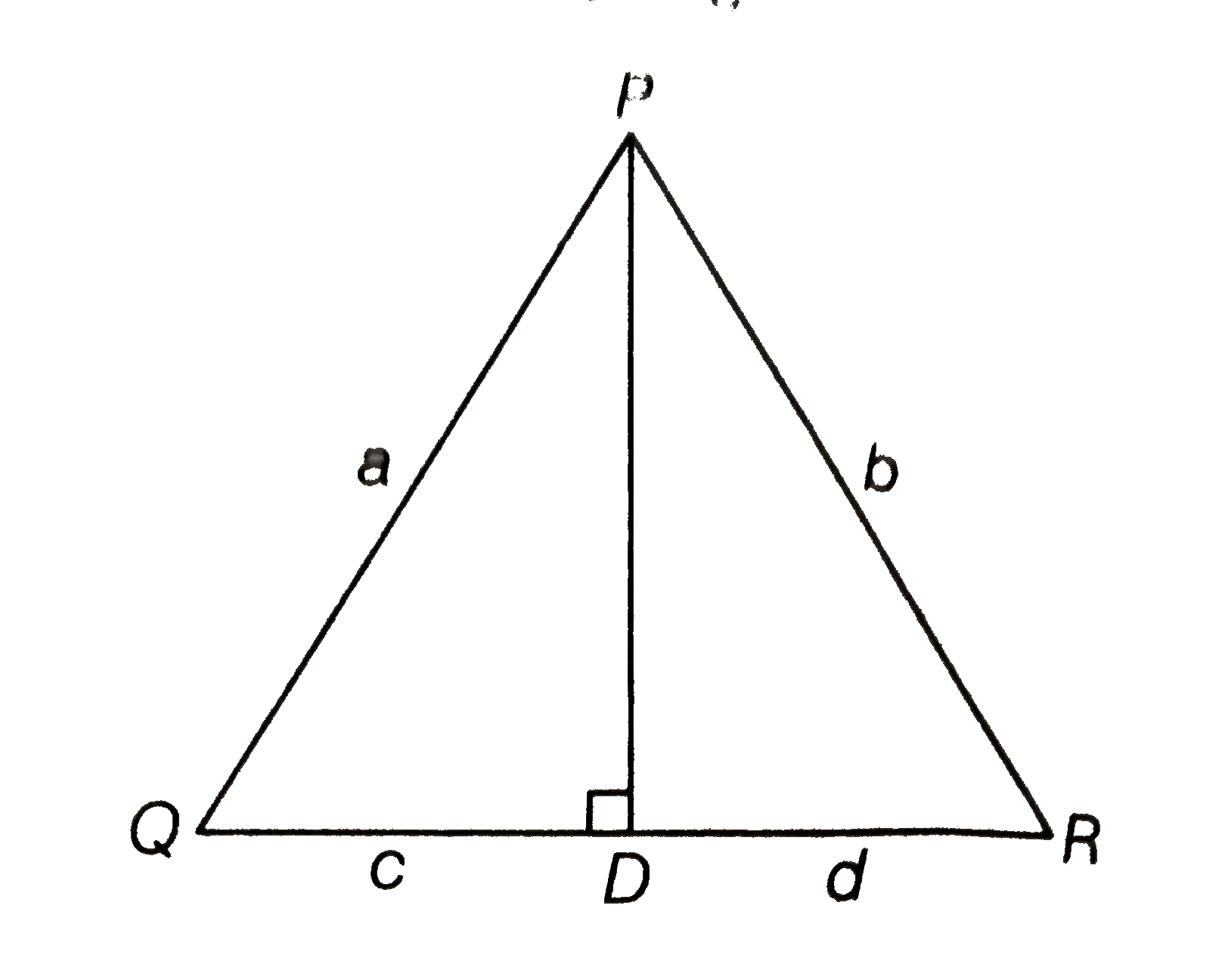
In right angled ΔPDRPR2=PD2+DR2 [by Pythagoras theorem]
⇒b2=PD2+d2
⇒PD2=b2−d2
From Eqs, (i) and (ii),
a2−c2=b2−d2
⇒a2−b2=c2−d2
⇒(a−b)(a+b)=(c−d)(c+d) Hence proved
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Ii ΔPQR, PD⊥QR such that D lies on QR, if PQ=a,PR=b,QD=c and DR=d, then prove that (a+b)(a-b)=(c+d)(c-d).
A(a−d)(a+d)=(b−c)(b+c)B(a−c)(b−d)=(a+c)(b+d)C(a−b)(a+b)=(c+d)(c−d)D(a−b)(c−d)=(a+b)(c+d)
If a, b, c, d are in G.P., then prove that:
(b−c)2+(c−a)2+(d−b)2=(a−d)2View SolutionIf a,b,c,d are in GP then prove that a+b,b+c,c+d are in GP.
View SolutionIn ΔPQR,PQ=QR,m∠PQR=120∘,Qd⊥PR,P−D−R then prove that
mPR=√3QR=√3PQView SolutionIf a, b, c, d are in GP, prove that
(b−c)2+(c−a)2+(d−b)2=(a−d)2.View SolutionIn Fig. 5.10, if AC=BD, then prove that AB=CD.
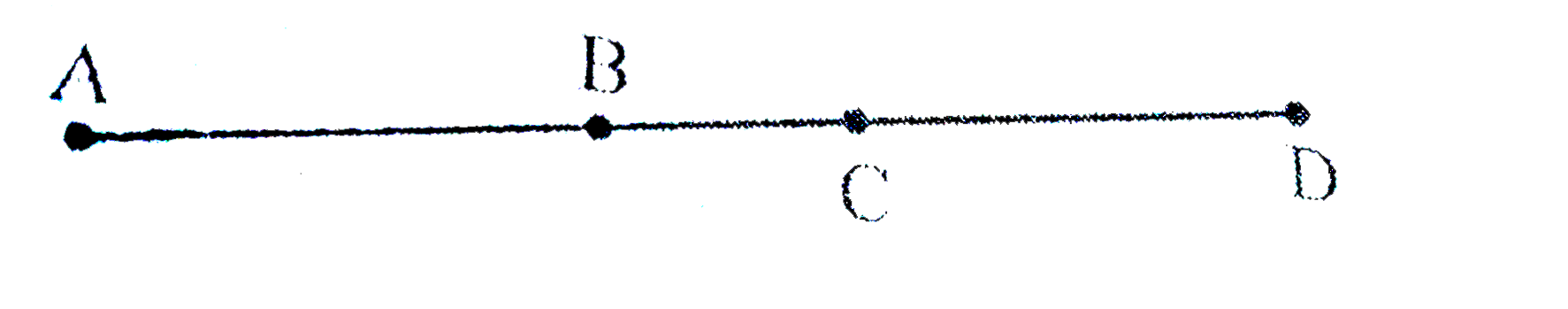 View Solution
View SolutionIf a, b, c and d are four coplanr points, then prove that [abc]=[bcd]+[abd]+[cad].
View SolutionIf A={a,b},B={c,d},C={d,e} then {(a,c),(a,d),(a,e),(b,c),(b,d),(b,e)}=
View Solution

