O is the point of intersection of the diagonals AC and BD of a trapezium ABCD with AB||DC. Through O , aline segment PQ is drawn parallel to AB meeting AD in P and BC in Q, prove that PO=QO.
Given ABCD is a trapezium, Diagonals AC and BD are intersect at O .
PQ||AB||DC
To prove PO=QO
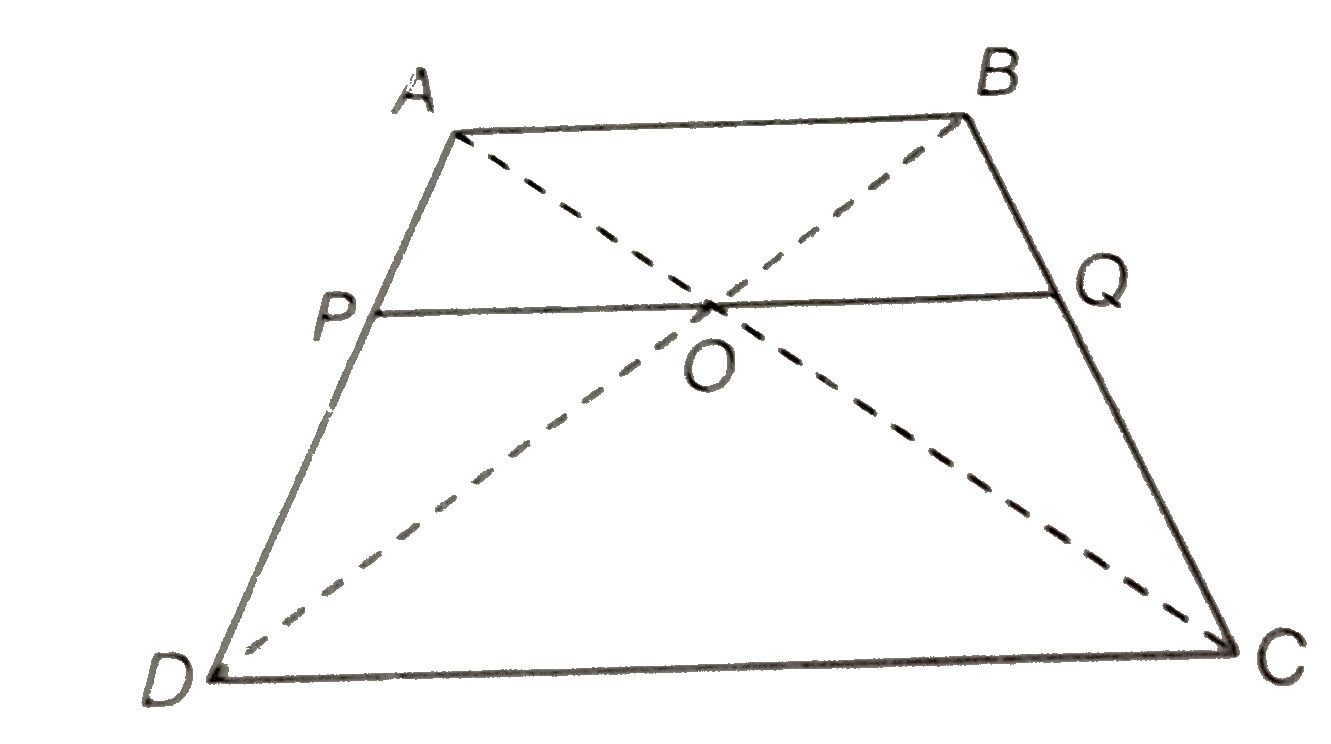
Proof in ΔABDandΔPOD,PO||AB [∵PQ||AB]
∠D=∠D [common angles]
∠ABD=∠POD [corresponding angles]
∴ΔABD~ΔPOD [by AAA similarity criterion]
Then, OPAB=PDAD...(i)
In ΔABCandΔOQC,OQ||AB [∵OQ||AB]
∠C=∠C [common angle]
∠BAC=∠QOC [corresponding angles]
∴ΔABC~ΔOQC [by AAA similarity criterion]
Then, OQAB=QCBC..(ii)
Now in ΔADCOP||DC
∴APPP=OAOC [by basic propertionality theorem]...(iii)
In ΔABC OQ||AB
∴BQQC=OAOC [by basic propertionality theorem]....(iv)
From Eqs. (iii) and (iv),
APPD=BQQC
Adding 1 on both sides, we get
APPD+1=BQQC+1
⇒AP+PDPD=BQ+QCQC
⇒ADPD=BCQC
⇒PDAD=QCBC
⇒OPAB=OQBC [from Eqs. (i) and (ii)]
⇒OPAB=OQAB [from Eq. (i)]
⇒OP=OQ Hence proved
Topper's Solved these Questions
Similar Questions
15 O is the point of intersection of the diagonals AC and BD ofa trapezium ABCD with AB || DC. Through O, a line segment PQ is drawn parallel to AB meeting AD in P and BC in Q. rove that PO=QO ,
View SolutionDiagonals ACandBD of a trapezium ABCD with AB∣∣DC intersect each other at O . Prove that ar(AOD)=ar(BOC) .
View Solution
Knowledge Check
- Question 1 - Select One
E is the mid-point of the side AD of the trapezium ABCD with AB∣∣DC. A line through E drawn parallel to AB intersects BC at F. then
APoint F trisect BCBPoint F bisect BCCAB=CDDNone
Diagonals AC and BD of a trapezium ABCD with AB || DC intersect each other at O. Prove that ar(ΔAOD) = ar(ΔBOC).
View SolutionDiagonals AC and BD of a trapezium ABCD with ABCD intersect each where at O. Prove that ar (AOD)=ar(BOC).
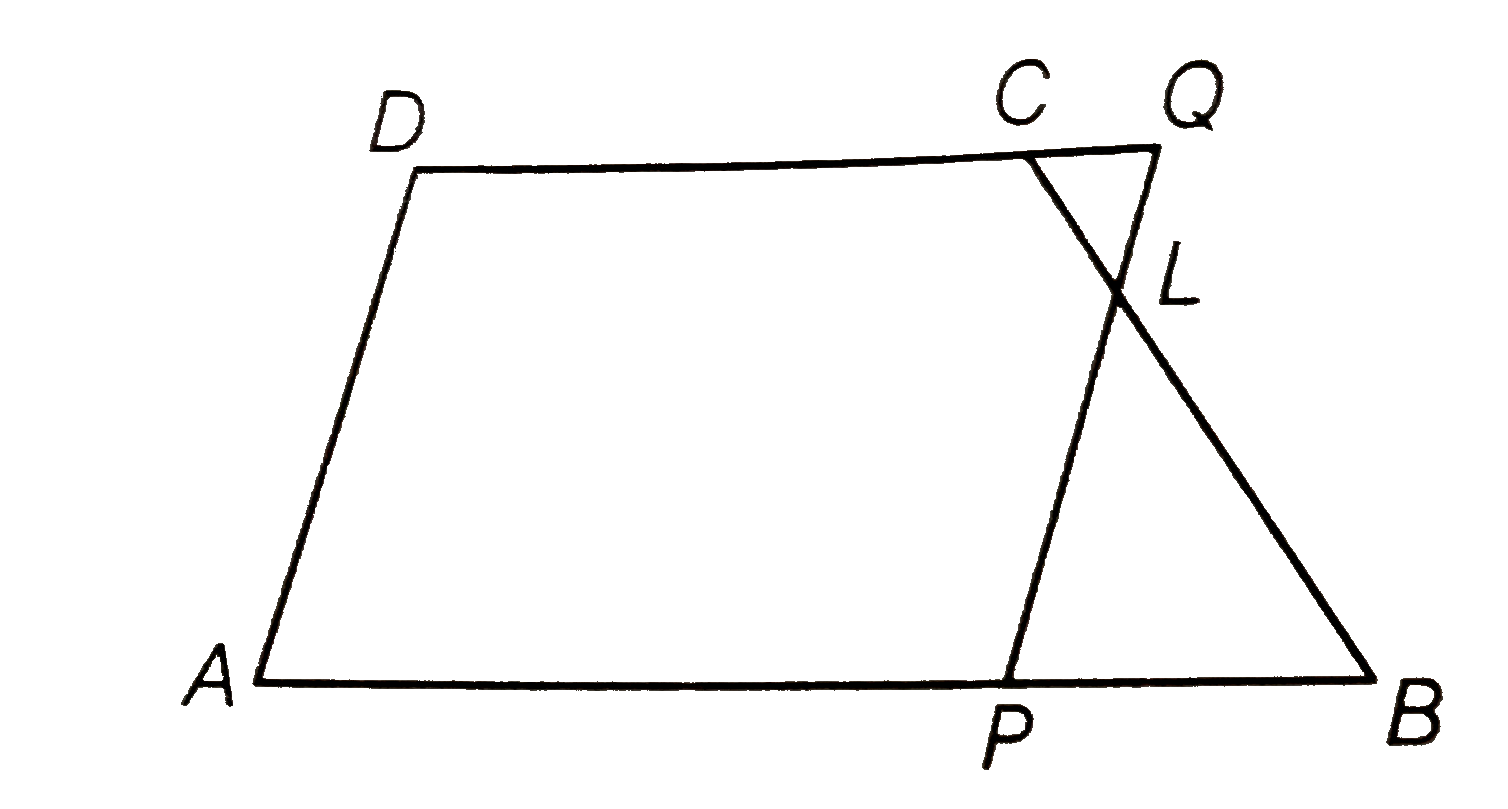
View SolutionIn trapezium ABCD, AB∣∣DC and L is the mid-point of BC. Through L, a line PQ∣∣AD has been drawn which meets AB in P and DC produced in Q. Prove that ar (ABCD) = ar (APQD).
 View Solution
View SolutionDiagonals AC and BD of a trapezium ABCD with AB || DC intersect each other at the point O. Using a similarity criterion for two triangles, show that OAOC=OBOD
View SolutionABCD is a trapezium with AB||DC. A line parallel to AC intersects AB at X and BC at Y. Prove that ar(ΔADX) = ar(ΔACY).
View SolutionIn △ ABC, the bisectors of ∠B and ∠ C intersect at I. A line drawn through I and parallel to BC intersects AB at P and AC at Q. Prove that PQ = BP + CQ.
View SolutionABCD is a trapezium with AB //DC. A line parallel to AC intersects AB at point M and BC at point N. Prove that: areaof△ADM=areaof△ACN.
View Solution

