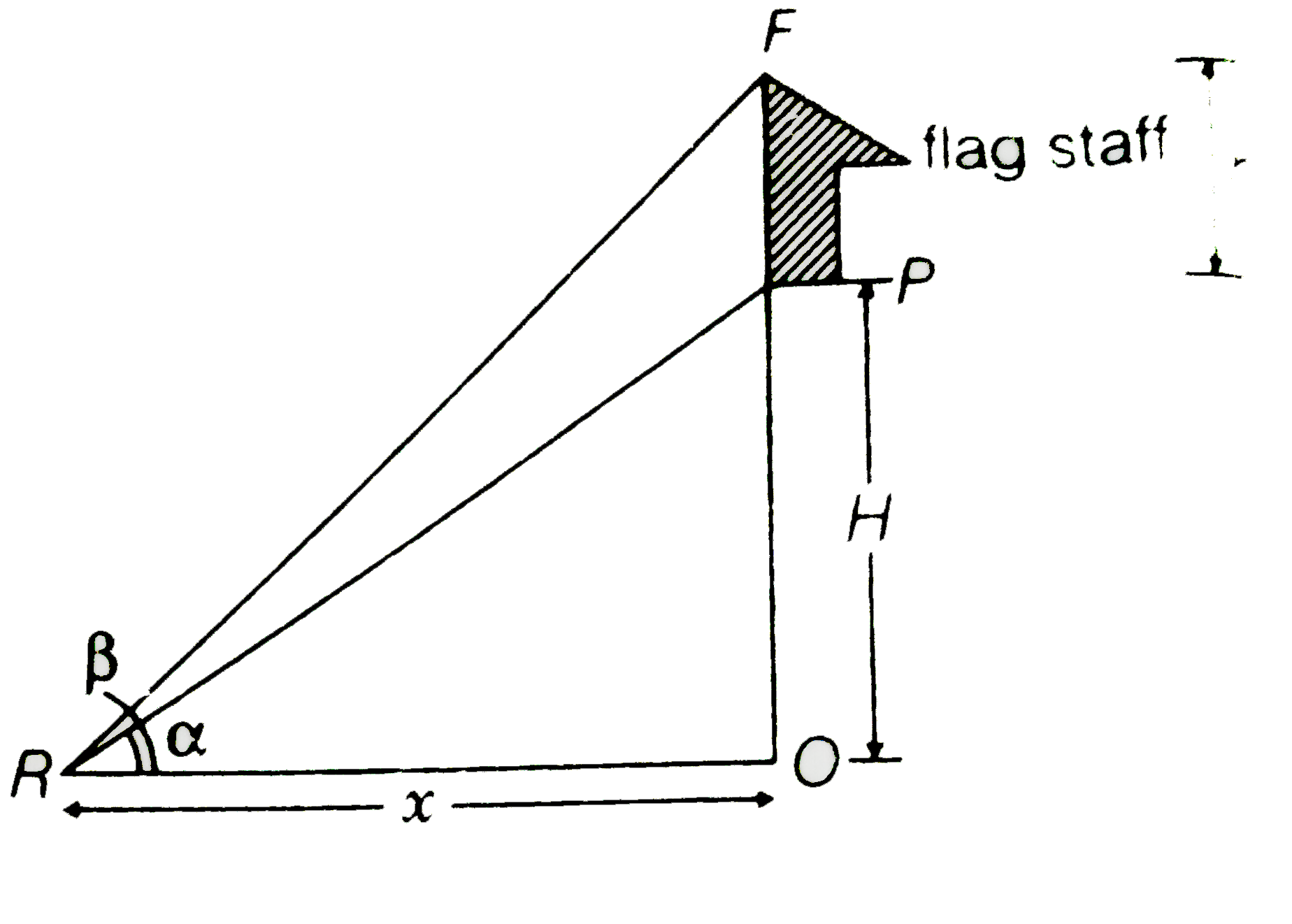
A vertical tower Stands on a horizontal plane and is surmounted by a vertical flag staff of height h. At a point on the plane, the angles of Elevation of the bottom and the top of the flag staff are $α and β$ respectively Prove that the height of the tower is $\frac{h tan α}{tan β - tan α}$

Video Solution

Text Solution

Verified by Experts

Let the height of the tower be H and OR=x
Given that, height of flag staff = h = FP and $∠ P R O = α, ∠ F R O = β$
Now, in $Δ P R O$ , $tan α = \frac{P O}{R O} = \frac{H}{x}$
$\Rightarrow x = \frac{H}{tan α}$
and in $Δ F R O, tan β = \frac{F O}{R O} = \frac{F P + P O}{R O}$
$tan β = \frac{h + H}{tan β}$ ...........(i)
From Eqs. (i) and (ii),

$\frac{H}{tan α} = \frac{h + H}{tan β}$
$H tan β = h tan α + H tan α$
$\Rightarrow H tan β - H tan α = h tan α$
$\Rightarrow H (tan β - tan α) = h tan α \Rightarrow H = \frac{h tan α}{tan β - tan α}$
Hence the required height of tower is $\frac{h tan α}{tan β - tan α}$ Hence Proved.

Updated on:21/07/2023