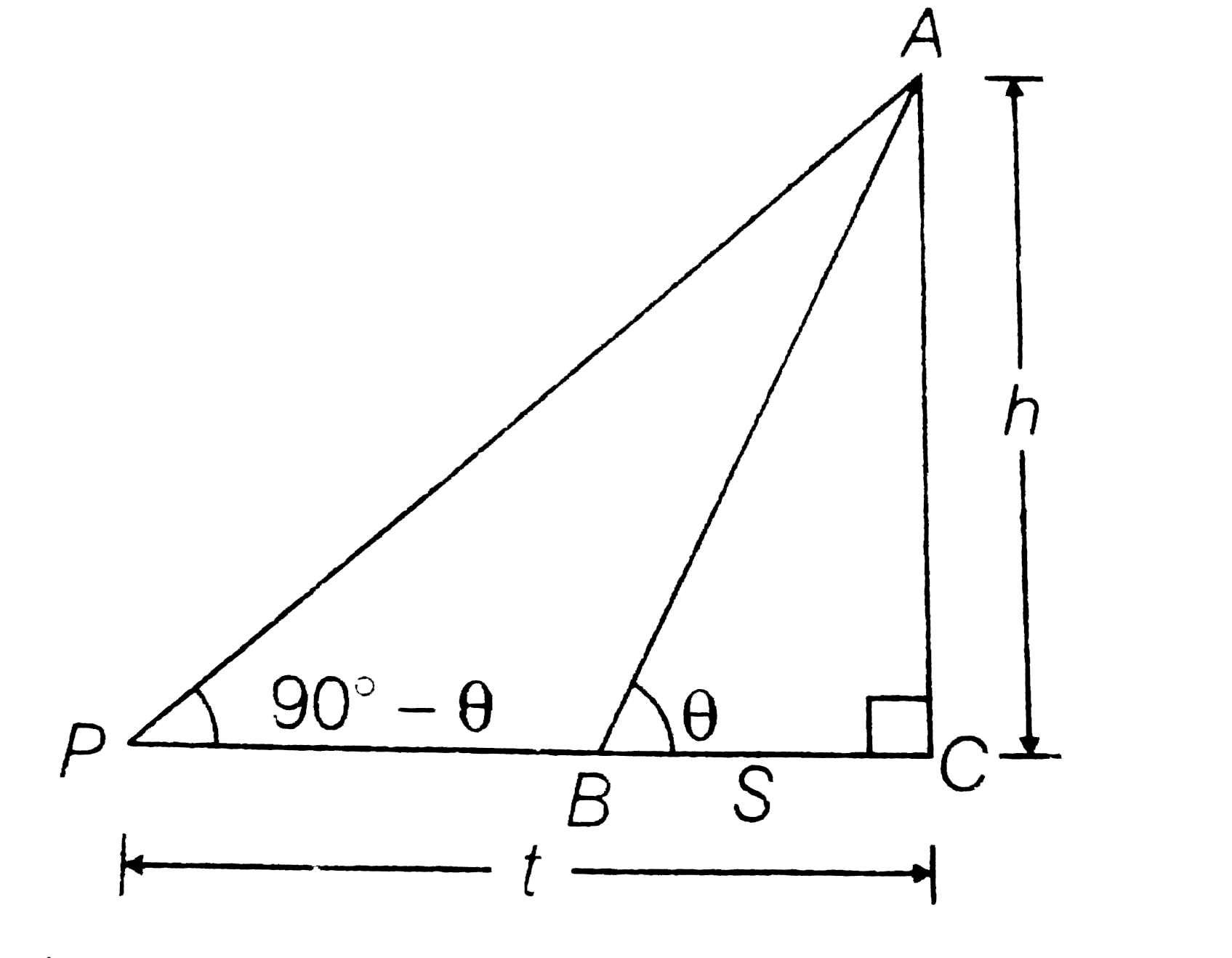
The angle of elevation of the top of a tower from two distinct points s and t from foot are complementary. Prove that the height of the tower is $\sqrt{s t}$ .

Video Solution

Text Solution

Verified by Experts

Let the height of the tower is h.
and $∠ A B C = θ$
Given that, BC=s, PC=t
and angle of elevation on both positions are complementary.
i.e., $∠ A P C = 90^{\circ} - θ$

[If two angles are complementary to each other, then the sum of both angles is equal to $90^{\circ}$ ]
Now in $Δ A B C$ , $tan θ = \frac{A C}{A B} = \frac{h}{s}$ ...................(i)
and in $Δ A P C$
$tan (90^{\circ} - θ) = \frac{A C}{P C}$ $[∴ tan (90^{\circ} - θ) = cot θ]$
$\Rightarrow cot θ = \frac{h}{t}$
$\Rightarrow \frac{1}{tan θ} = \frac{h}{t}$ $[∴ cot θ = \frac{1}{tan θ}]$ ...............(ii)
On multyplying Eqs. (i) and (ii), we get
$tan θ . \frac{1}{tan θ} = \frac{\frac{h}{s}}{\frac{h}{t}}$
$\Rightarrow \frac{h^{2}}{s t} = 1$
$\Rightarrow h^{2} = s t$
$\Rightarrow h = \sqrt{s t}$
So, the required height of the tower is $\sqrt{s t}$ Hence Proved.

Updated on:21/07/2023

If the angles of elevation of the top of a tower from two points distance s and $t (s > t)$ from its foot are 30° and 60° respectively, then the height of the tower is
A $\sqrt{s + t}$
B $\sqrt{s t}$
C $\sqrt{s - t}$
D $\sqrt{\frac{s}{t}}$
The angle of elevation from two points at a distance of x and y from the feet of a Tower are complementary, the height of the Tower is
A $\sqrt{x y}$
B $\frac{x}{y}$
C $\sqrt{\frac{x}{y}}$
D $\sqrt{x + y}$
The angle of elevation of the top of a tower from the point P and Q at distance of a and b respectively from the base of the tower and in the same straight line with it are complementary .The height of the tower is
A $\sqrt{a b}$
B $\frac{a}{b}$
Cab
D $a^{2} b^{2}$