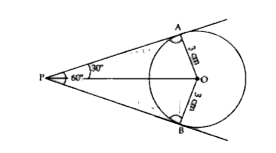
If two tangents inclinced at an angle 60∘ are drawn to a circle of radius 3 cm, then find the length of each tangent.
32√3cm
6 cm
3 cm
3√3cm
The correct Answer is:D
Let P be an external point and a pair of tangents is drawn from point P and angle between these two tangents is 60∘
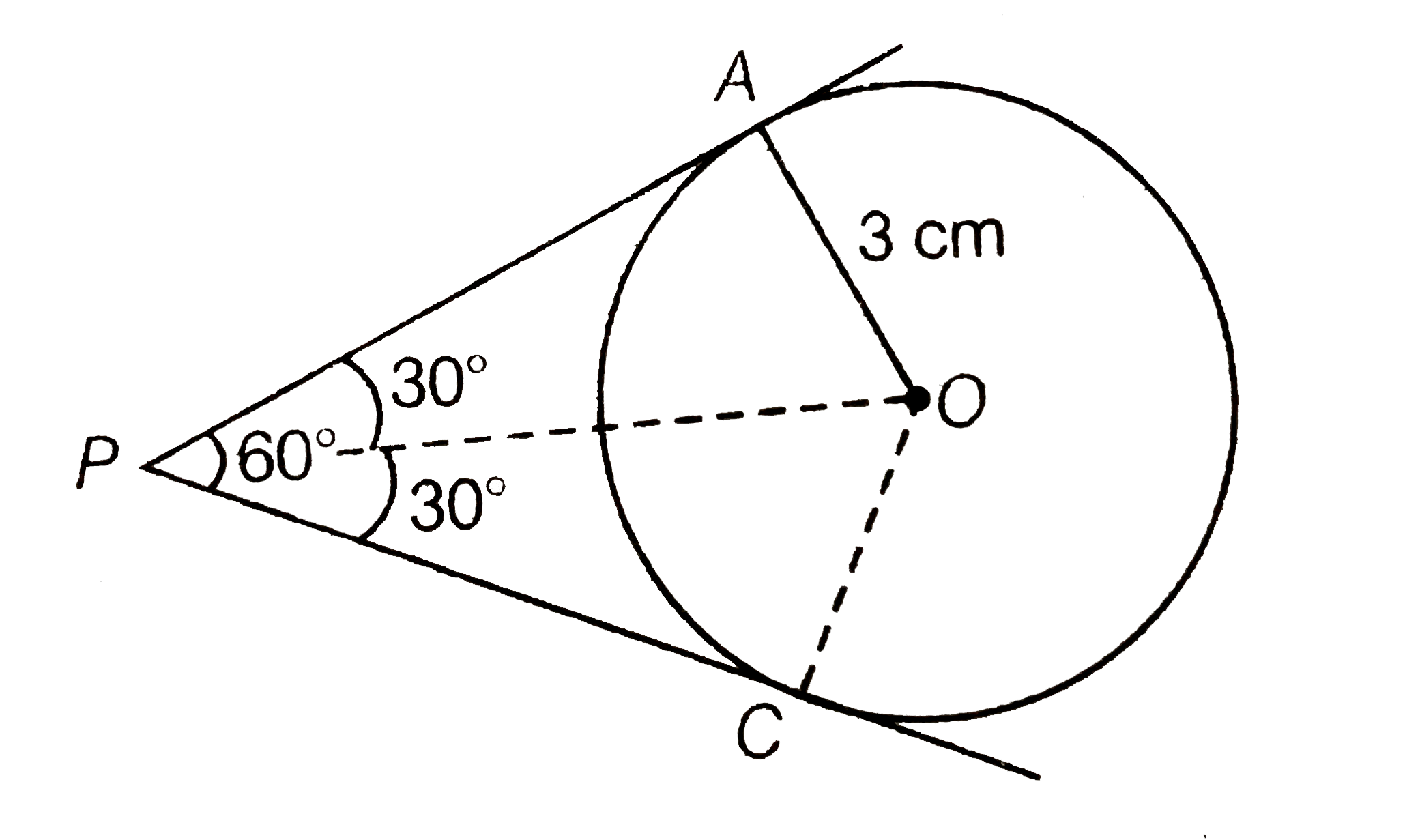
Join OA and OP.
Also, OP is a bisector line of ∠APC
∴∠APO=∠CPO=30∘
Also, OA⊥AP Tengent at any point of a circle is perpendicular to the radius through the point of contact.
In right angled ΔOAP, tan30∘=OAAP=3AP
⇒1√3=3AP
⇒AP=3√3cm
Hence, the length of each tangent is 3√3cm.
Topper's Solved these Questions
Similar Questions
Knowledge Check
IF two tangents inclined at an angle of 60∘ are drawn to a circle of radius 3 cm , then the length of each tangent is equal to
A3√32 cmB6 cmC3 cmD3√3cmIf two tangents inclined at an angle of 60∘ are drawn to a circle of radius 4 cm, then the length of each tangent is equal to :
A2√3 cmB8 cmC4 cmD4√3 cmIf two tangents inclined at an angle of 60∘ are drawn to a circle of radius 3cm then the length of each tangent is
A3cmB3√32cmC3√3cmD6cm
If two tangents inclined at an angle 60° are drawn to a circle of radius 3 cm, then what is the length of each tangent ?
View SolutionIf two tangents inclined at an angle 60∘ are drawn to a circle of radius 3 cm, then, the length of each tangent is equal to
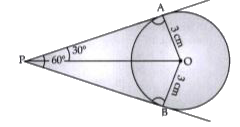
View SolutionIf two tangents are inclined at 60∘ are drawn to a circle of radius 3cm then find length of each tangent.
OR
PQ is a tangent to a circle with centre O at point P. If ΔOPQ is an isosceles triangle, then find ∠OQP.
View SolutionTwo tangents making an angle of 60∘ between them, are drawn to a circle of radius √2 cm, then find the length of each tangent.
View SolutionTwo tangents making an angle of 1200 with each other are drawn to a circle of radius 6cm, then the length of each tangent is equal to √3 cm (b) 6 √3 cm (c) √2 cm (d) 2 √3 cm
View Solution