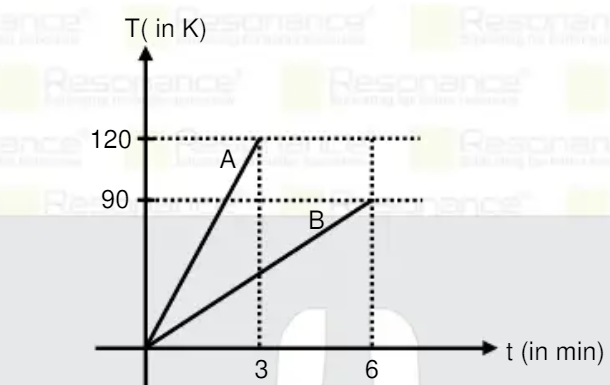
The graph shown in the adjacement diagram represents the variation of temperature (T) of two bodies x and y having the same surface area, with time (t). Both of these bodies lose heat only due to the emission of radiation. Find the correct relation between the emissive and absorptive power of the two bodies.
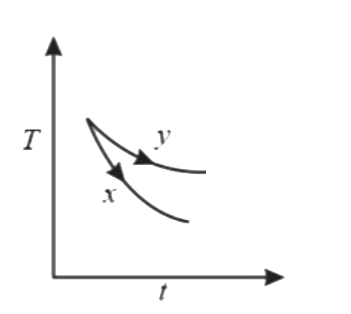
Ex>Eyandax<ay
Ex<Eyandax>ay
Ex>Eyandax>ay
Ex<Eyandax<ay
The correct Answer is:C
Step by step video, text & image solution for The graph shown in the adjacement diagram represents the variation of temperature (T) of two bodies x and y having the same surface area, with time (t). Both of these bodies lose heat only due to the emission of radiation. Find the correct relation between the emissive and absorptive power of the two bodies. <Br> by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A hot water radiator at 310 K temperature radiates thermal radiation like a black body. Its total surface area is 1.6m2. Find the thermal power radiated by it.
View SolutionTwo bodies are thrown simultaneously from the same point. One thrown straight up and the other at an angle α with the horizontal. Both the bodies have velocity equal to u. Find the separation between the bodies at time t.
View Solution
Knowledge Check
The graph, shown in the adjacent diagram, represents the variation of temperature (T) of two bodies, x and y having same surface area, with time (t) due to the emission of radiation. Find the correct relation between the emissivity and absorptivity power of the two bodies
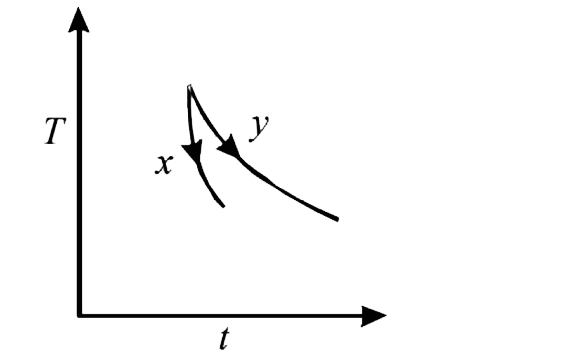 AEx>Ey&ax<ayBEx<Ey&ax>ayCEx>Ey&ax>ayDEx<Ey&ax<ay
AEx>Ey&ax<ayBEx<Ey&ax>ayCEx>Ey&ax>ayDEx<Ey&ax<ayIf the rate of emission of radiation by a body at temperature TK is E then graph between log E and log T will be .
A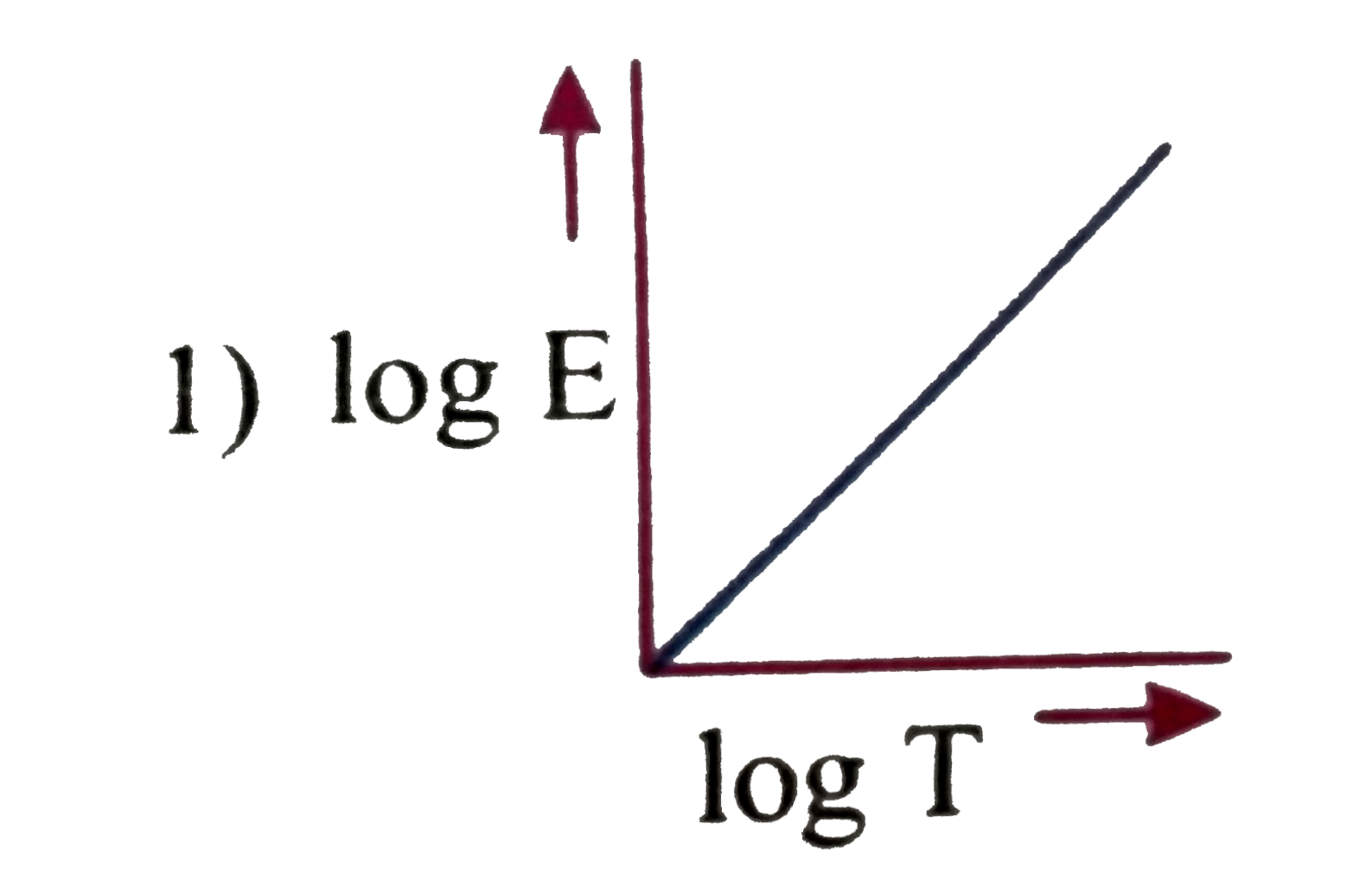 B
B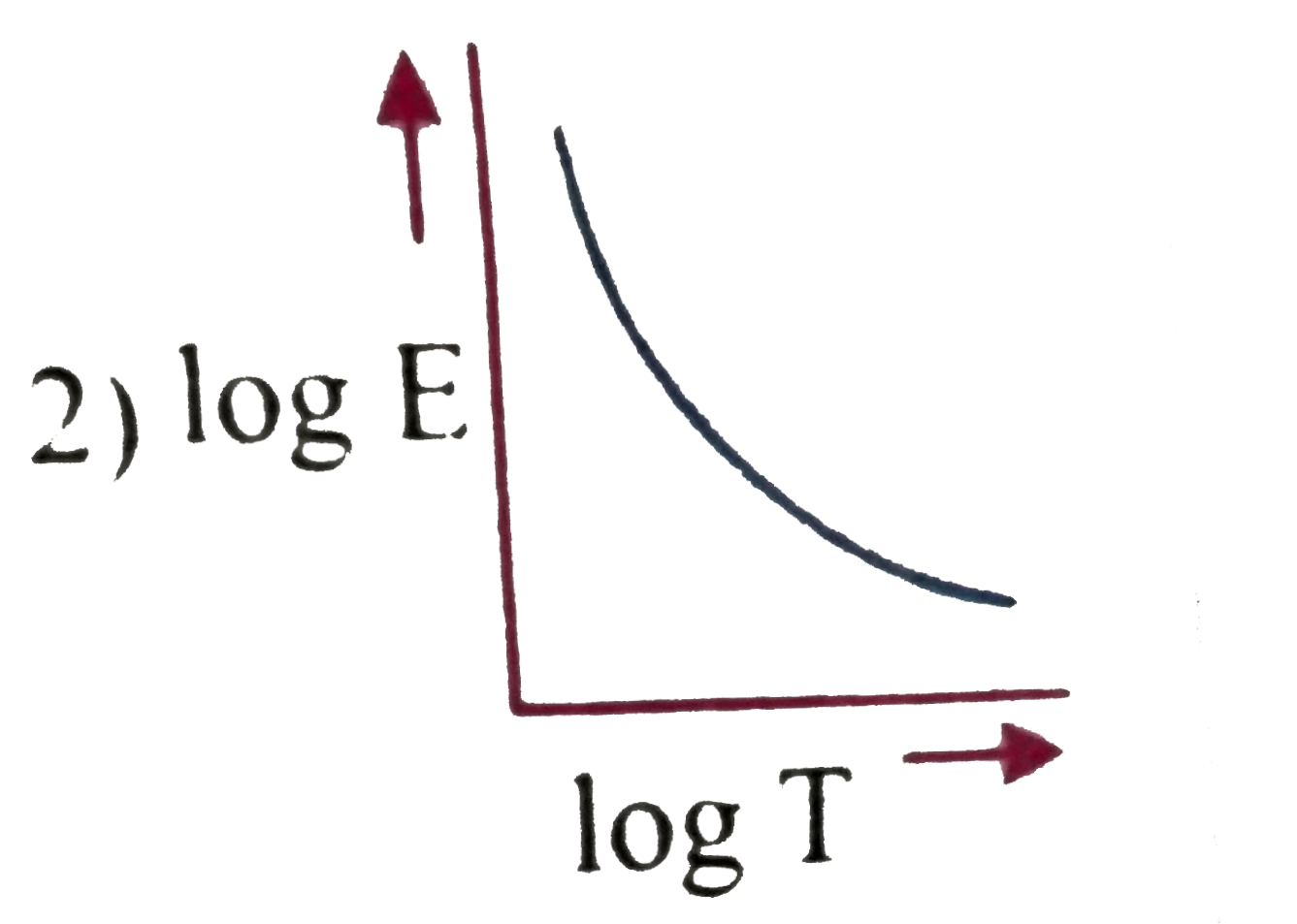 C
C D
D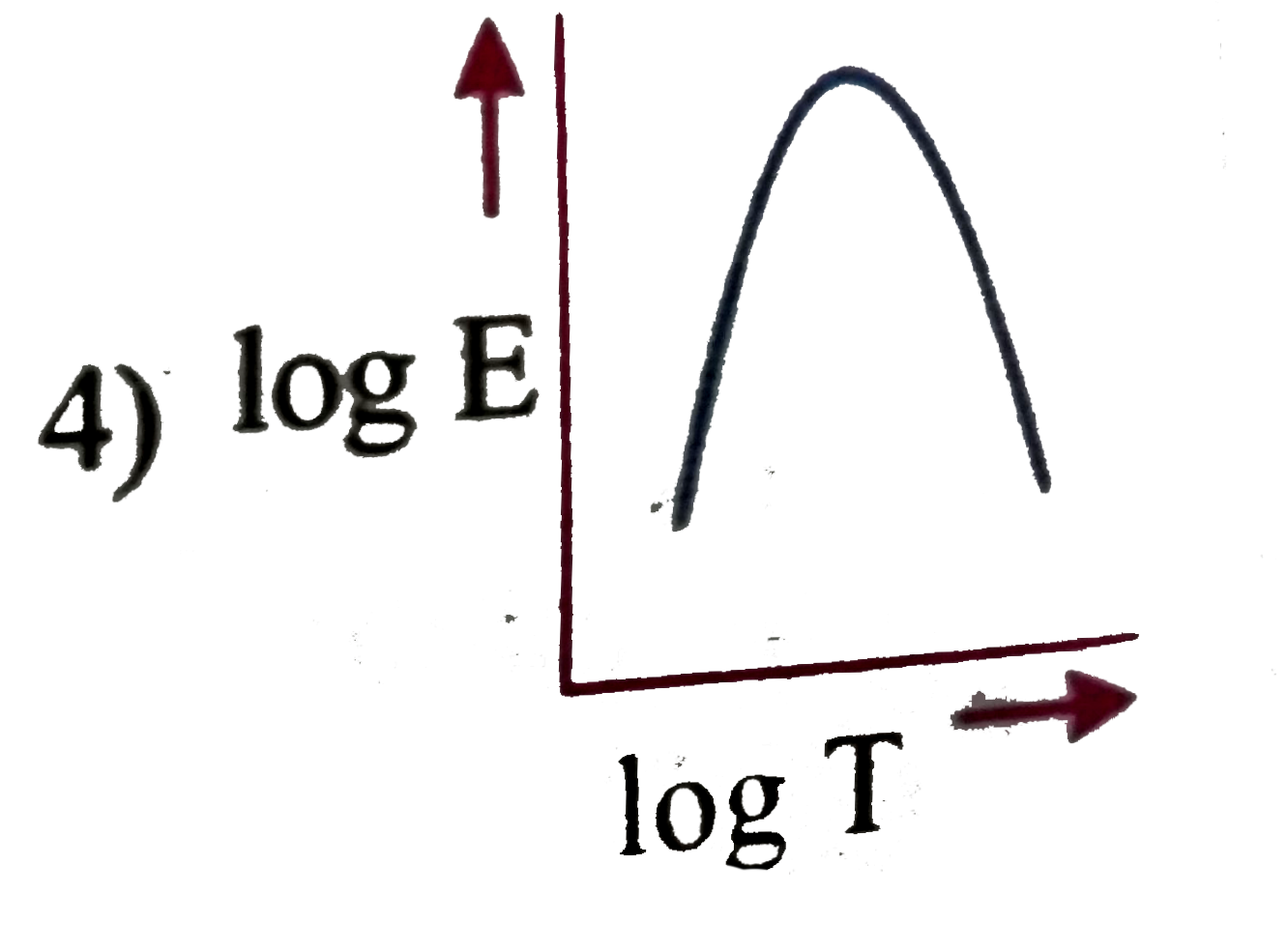
Two bodies A and B having equal surface areas are maintained at temperatures 10∘C . And 20∘C . The thermal radiation emitted in a given time by A and B are in the ratio
A1:1.15B1:02C1:04D1:16 .
The temperature vs time graph for two different gases A and B having same number of moles is as shown in figure. If heat is supplied by same rate to both the gases. Find ratio of specific heat capacity of both gases.
View SolutionA projectille can have the same rangeR for two angles of projection. If t1 and t_(2)bethetimeofflight∈thetwocases,thenf∈dtherelationbetween t_(1), t_(2)andR .
View SolutionA body is kept inside a container the temperature of the body is T1 and the temperature of the container is T2 the rate at which body absorbs the energy is α The emissivity of the body is The radiation striking the body is either absorbed or reflected
Ater a long time the temperature of the body will be .
View SolutionWhich of the following x-t graphs represents the distance-time variation of a body released from the top of a buiding?
View SolutionAt a given temperature the ratio between emissive power and absorptive power is same for all bodies and is equal to the emissive power of black body This statemetn is called .
View Solution
