A long cylindrical conductor of cross-sectional area A and radius a is made of material whose resistivity depends only on a distance r from the axis of conductor, given by ρ=cr2, where c is a constant. Find the resistance per unit length of the conductor and the electric field strength due to which a current I flows in it.
R=4αlπa3
R=2αlπa4
R=4απla3
R=2lπαa3
The correct Answer is:B
Step by step video, text & image solution for A long cylindrical conductor of cross-sectional area A and radius a is made of material whose resistivity depends only on a distance r from the axis of conductor, given by rho = c/r^(2), where c is a constant. Find the resistance per unit length of the conductor and the electric field strength due to which a current I flows in it. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A long round conductor to cross-sectional area S is made of material whose resistivity depends only on a distance r from the axis of the conductor as ρ=α/r4, where α is a constant. Find :
(a) the resistance per unit length of such a conductor,
(b) the electric field strength in the conductor due to which a current I flow though it.
View SolutionA long cylindrical conductor of radius R carries a current i as shown in the figure. The current density J varies across the cross-section as J=kr2, where, k is a constant. Find an expression for the magnetic field B at a distance r(<R) from the axis

View Solution
Knowledge Check
A solid cylinder of length l and cross-sectional area A is made of a material whose resistivity depends on the distance r from the axis of the cylinder as ρ=k/r2 where k is constant . The resistance of the cylinder is -
AπklA2B2πklA2CπklADNoneA solid cylindrical wire of length l has cross-section radius a as shown below. The specific resistance of material is given as ρ=ρ0r, where r is distance from axis of cylinder and ρ0 is a positive constant quantity. The resistance across its ends is :
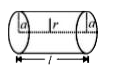 Aρ0l2πaBρ0lπa2Cρ0lπaD2ρ0lπ
Aρ0l2πaBρ0lπa2Cρ0lπaD2ρ0lπThe magnetic field B due to a straight conductor of uniform cross-section of radius 'a' and carrying a steady current varies with the distance 'r' from the centre of conductor as shown in graph .
A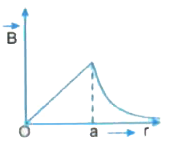 B
B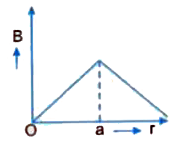 C
C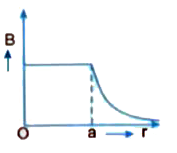 D
D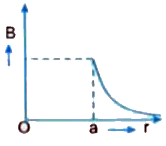
Two cylindrical conductors with equal cross-sections and different resistivites ρ1 and ρ2 are point end to end. Find the charge at the boundary of the conduction if a current I flows from conductor 1 to conductor 2
View SolutionA cylindrical conductor of length l and radius a has conductivity near its axis as σ. The conductivity of material increases linearly with the distance from axis and becomes 2σ near the surface.The resistance of conductor for longitudinal current is
View SolutionIf the current density in a linear conductor of radius 'a' varies with r according to relation J=kr2, where k is a constant and r is the distance of a point from the axis of conductor. Find the magnetic field induction at a point distance r from the axis, when (i) r<a and (ii) r>a.
View SolutionA cylindrical conductor of radius R is carrying a constant current. The plot of the magnitude of the magnetic field, B with the distance, d from the centre of the conductor, is correctly represented by the figure
View SolutionA cylindrical conductor of radius R is carrying constant current. The plot of the magnitude of the magnetic field, B with the distance, d from the centre of the conductor , is correctly represented by the figure:
View Solution
