A heavy box is slid across a rough floor with an initial speed of 4 m/s. It stops moving after 8 seconds. If the average resisting force of friction is 10 N, the mass of the box (in kg) is
40
20
5
2.5
To find the mass of the box, we can use the information provided in the question and apply Newton's second law of motion along with the equations of motion.
1. Identify the given values:
- Initial velocity (u) = 4 m/s
- Final velocity (v) = 0 m/s (since the box stops)
- Time (t) = 8 s
- Average resisting force of friction (F_friction) = 10 N
2. Calculate the acceleration (a):
We can use the equation of motion:
v=u+at
Rearranging this gives:
a=v−ut
Substituting the known values:
a=0−48=−48=−0.5m/s2
(The negative sign indicates that the acceleration is in the opposite direction of the motion, which is expected due to friction.)
3. Apply Newton's second law:
According to Newton's second law:
F=ma
The only force acting on the box is the frictional force, which is equal to the mass of the box (m) multiplied by the acceleration (a):
Ffriction=m⋅a
We know that the frictional force is 10 N, so we can set up the equation:
10=m⋅(−0.5)
4. Solve for the mass (m):
Rearranging the equation gives:
m=10−0.5=−20kg
Since mass cannot be negative, we take the absolute value:
m=20kg
Final Answer:
The mass of the box is 20 kg.
- Initial velocity (u) = 4 m/s
- Final velocity (v) = 0 m/s (since the box stops)
- Time (t) = 8 s
- Average resisting force of friction (F_friction) = 10 N
We can use the equation of motion:
v=u+at
Rearranging this gives:
a=v−ut
Substituting the known values:
a=0−48=−48=−0.5m/s2
(The negative sign indicates that the acceleration is in the opposite direction of the motion, which is expected due to friction.)
According to Newton's second law:
F=ma
The only force acting on the box is the frictional force, which is equal to the mass of the box (m) multiplied by the acceleration (a):
Ffriction=m⋅a
We know that the frictional force is 10 N, so we can set up the equation:
10=m⋅(−0.5)
Rearranging the equation gives:
m=10−0.5=−20kg
Since mass cannot be negative, we take the absolute value:
m=20kg
The mass of the box is 20 kg.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - AAKASH INSTITUTECHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-B)
44 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTECHAPTER - LAWS OF MOTIONEXERCISE - Assignment (SECTION-C)
63 Videos
- LAWS OF MOTIONBOOK - AAKASH INSTITUTECHAPTER - LAWS OF MOTIONEXERCISE - EXERCISE
70 Videos
- KINETIC THEORYBOOK - AAKASH INSTITUTECHAPTER - KINETIC THEORYEXERCISE - EXERCISE (ASSIGNMENT) SECTION - D Assertion - Reason Type Questions
10 Videos
- MAGNETISM AND MATTER BOOK - AAKASH INSTITUTECHAPTER - MAGNETISM AND MATTER EXERCISE - ASSIGNMENT (SECTION D)
26 Videos
Similar Questions
A 5kg box is moving staright across the floor at a constant speed by a force of 30N as shown in figure
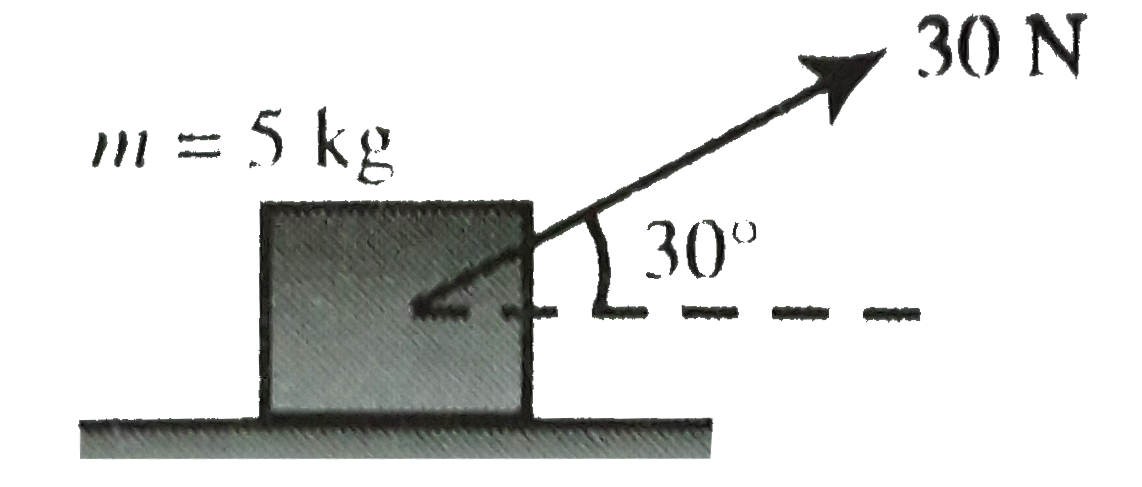
a. How large a friction force impends the motion of the box ?
b. Find μk between the box and the floor
View SolutionA box of mass 20kg is pushed along a rough floor with a velocity 2 m/s and then let go .The box moves 5m on the floor before coming to rest. What must be the frictional force acting on the box? "
(1) 4N
(2) 2N
(3) 20N
(4) 8N
View Solution
Knowledge Check
A 10 kg block is set moving with an initial speed of 6 m/s on a rough horizontal surface. If the force of friction is 20 N, approximately how far does the block travel before it stops?
A1.5mB6 mC3 mD9 mA bullet of mass 0.05 kg moving with a speed of 80m/s enters a wooden block and is stopped after a distance of 0.40 m The average resistive force exerted by the block on the bullet is:
A300 NB20 NC400 ND40 NA 42 kg block of ice moving on rough horizontal surface stops due to friction, after sometime. If the initial velocity of the decelerating block is 4ms−1, the mass of ice (in kg) that has melted due to the heat generated by the friction is (Latent heat of ice is 3.36×105Jkg−1
A1×10−3B1.5×10−3C2×10−3D2.5×10−3
A box is pushed through 4.0m across a floor offereing 100N resistance. How much work is done by the (i) applied force (ii) resisting force?
View Solution5A body of mass 15 kg moving with a velocity of 10 m/s is to be stopped by a resistive force in 15 sec, the force will be -
View SolutionA bullet of mass 40g moving with a speed of 90ms−1 enters a heavy wooden block and is stopped after a direction of 60cm. The average resistive force exerted by the block on the bullet is
View SolutionA bullet of mass 0.04 kg moving with a speed of 600 m/s penetrates a heavy wooden block. It stops after a penetration of 500 cm. The resistive force exerted by the block on the bullet is
View SolutionA man of mass 80kg pushes box of mass 20kg horizontaly. The man moves the box with a constant acceleration of 2m/s2 but his foot does not slip on the ground. There is no friction between the box and the ground. There is no friction between the box and the ground. whereas there is sufficient friction between the man's foot and the ground to prevent him from slipping.
Assertion:- The force applied by the man on the box is equal and opposite to the force applied by the force applid by the box on the man.
Reason:- Friction force applied by the ground on the man is 200N.
View Solution
