Find the area bounded by the curve y=2cosx and the X-axis from x = 0 to x=2π.
Video Solution
Text Solution
The correct Answer is:N/a
Required area of shaded region =∫2π02cosxdx
=∫π/202cosxdx+∣∣∣∫3π/2π/22cosxdx∣∣∣+∫2π3π/22cosxdx
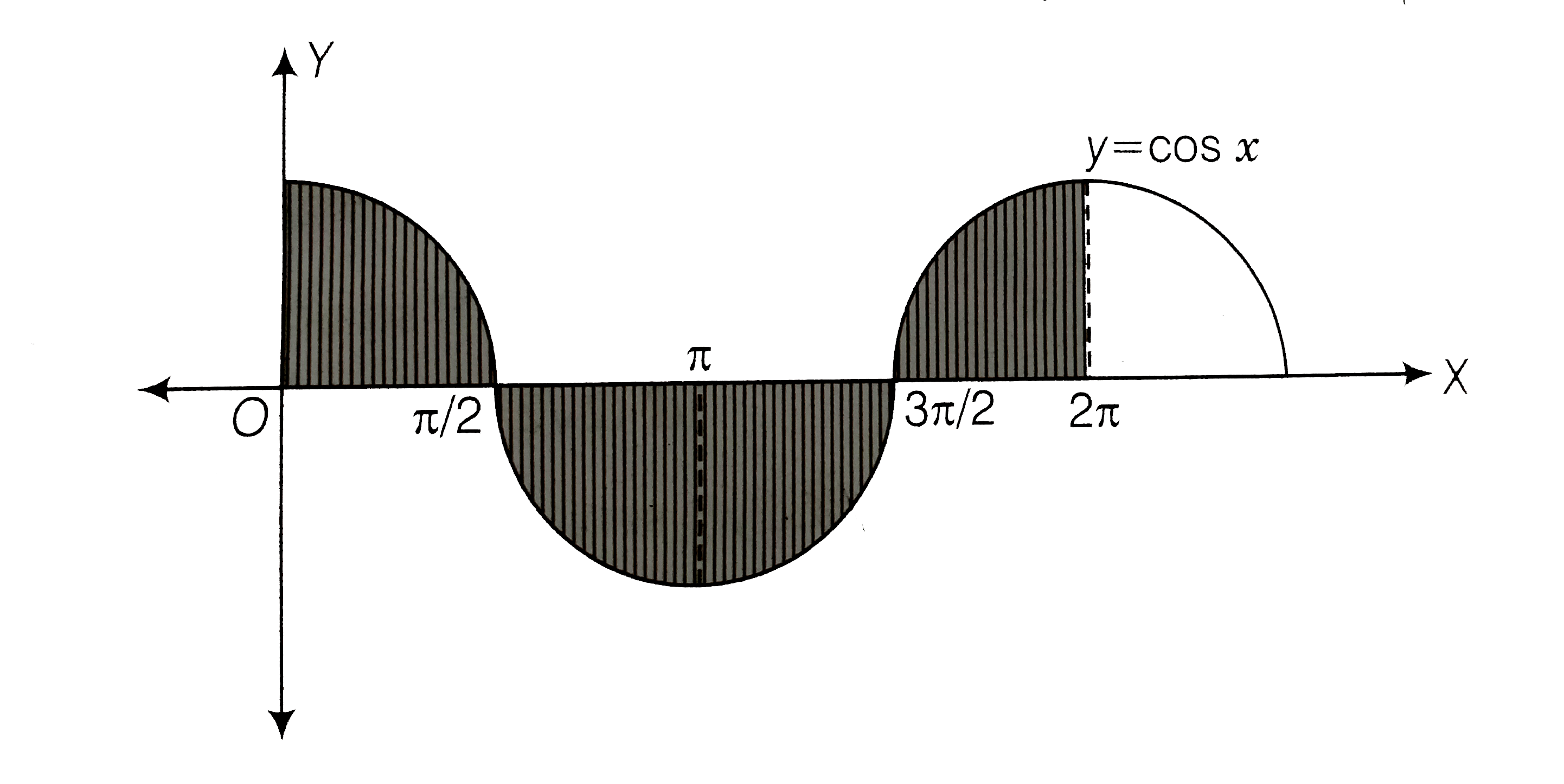
=2[sinx]π/20+∣∣2(sinx)3π/2π/2∣∣+2[sinx]2π3π/2
=2+4+2=8 sq units
|
Topper's Solved these Questions
Similar Questions
Knowledge Check
Area bounded by the curve y=x2+x−6 and the X-axis is
A1276B2256C1256DNone of theseThe area bounded by the curve y=sin−1(sinx) and the x - axis from x=0 to x=4π is equal to the area bounded by the curve y=cos−1(cosx) and the x - axis from x=−π to x=a, then the value of a is equal to
Aπ2B2πCπD3π2
Find the area bounded by the curves x+2|y|=1 and x=0 .
View SolutionFind the area bounded by the curve y=−3|x|+2 and x-axis
View SolutionFind the area bounded by the curve y=x2,x=1,x=2 and x-axis .
View SolutionFind the area bounded by the curves y=√x,2y+3=x and x-axis.
View SolutionFind the area bounded by the curve |x|+y=1 and axis of x.
View SolutionFind the area bounded by the curve y=(x+1)(x−1)(x+2) and the x-axis.
View Solution