A car of mass 1200 kg (together with the driver) is moving with a constant acceleration of 2 m/s2. How much power does the engine generate at the instance, when the speed reaches 20 m/s? (Assume that the coefficient of friction between the car and the road is 0.5).Solution in Telugu
48000 W
120000 W
168000 W
288000 W
The correct Answer is:C
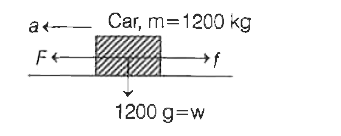
Power of engine overcomes friction and provides necessary acceleration to the car.
Now friction,f=μ mg =0.5×1200×10= 6000 N and accelerating force, F =ma=1200×2= 2400 N
So, total force produced by engine =FT=8400 N
We know that, the formula of power is
P=FTv
Then, P 8400×20=168000 W
Topper's Solved these Questions
Similar Questions
A nucleus of deuterons or deuterium is a bound atomic system best described by
View SolutionThe pressure on a circular plate is measured by measuring the force on the plate and the radius of the plate. If the errors in measurement of the force and the radius are 5% and 3% respectively, the percentage of error in the measurement of pressure is
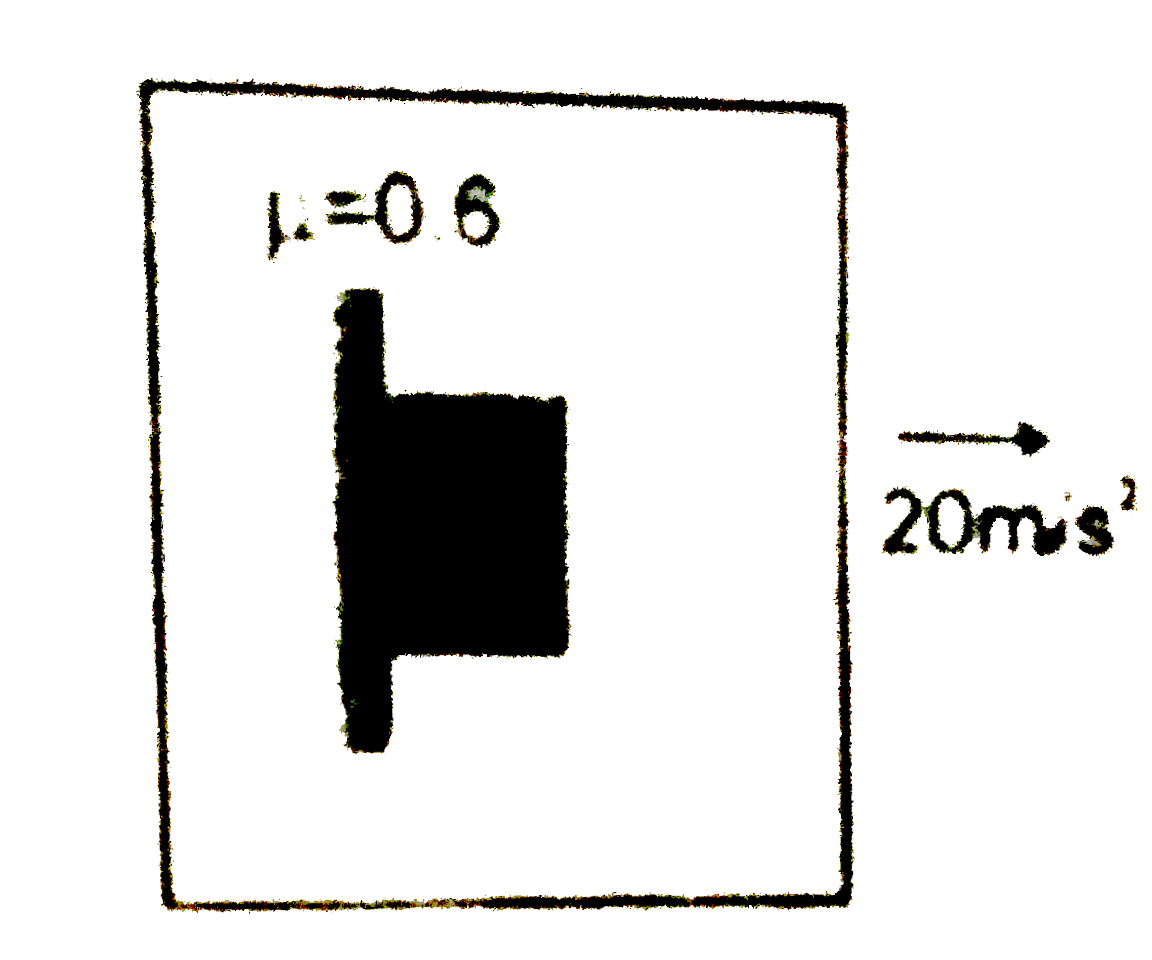
View SolutionCar is accelerating with acceleration =20m/s2. A box of mass m=10 kg that is placed inside the car, it is put in contact with the vertical wall of car as shown. The friction coefficient between the box and the wall μ=0.6
View SolutionA car is moving with speed v and is taking a turn on a circular road of radius 10 m. The angle of banking is 37∘. The driver wants that car does not slip on the road. The coefficient of friction is 0.4(g=10m/sec2)
The friction force acting when v=10m/sec and mass of car is 50 kg is :
View SolutionA car is moving on a circular road of diameter 50 m with a speed of 5 m/s. It is suddenly accelerated at a rate of 1m/s2. If the mass of he car is 500 kg, then the net force acting on the car is
View Solutionएक कार सीधी सड़क पर 20 मीटर/सेकंड की चाल से जा रही है। सड़क तथा कार के टायरों के बीच घर्षण गुणांक 0.4 है। वह न्यूनतम दूरी ज्ञात कीजिए जिसमे कार रुक जायेगी। (g = 10 मीटर/सेकंड2)
View SolutionA car is moving along a horizontal curve of radius 20 m , and coefficient of friction between the road and wheels of the car is 0.25 . If acceleration due to gravity is (9.8m/s2), then its maximum speed is
View Solution500 किग्रा की कार की चाल 20 मीटर/सेकंड से बढ़ाकर 40 मीटर/सेकंड की गयी। कार के इंजन ने कितना कार्य किया?
View SolutionA car is moving on a horizontal circular tracck of radius 0*2 km with a constant speed. If coefficient of friction between tyres of car and road is 0*45 , then speed of a car may be [ Take g = 10 m/s^(2)]
View SolutionA car of mass 1200 kg (together with driver) is moving with a constant acceleration of 2m/s2 . How much power does the speed reaches 20m/s2.? ( assume that the coefficient of friction between the car and the road is 0.5)
View SolutionA car of mass 1500 kg is moving with a speed of 12.5 m cdot s^(-1) on a circular path of radius 20 m on a level road. What should be the frictional force between the car and the road so that the car does not slip? What should be the value of the coefficient of friction this force?
View SolutionA car of mass 500 kg is moving up along an inclined surface of slope 1/(25) at a constant speed of 72 km*h^(-1) . If the coefficient of friction between the road and the car wheel is 0.1 , find the power of the car engine (g= 9.8 m*s^(-2) ).
View SolutionWhen a car of mass 1200 kg is moving with a velocity of 15 ms^(-1) on a rough horizontal road, its engine is switched off. How far does the car travel before it comes to rest if the coefficient of kinetic friction between the road and tyres of the car is 0.5? ( g=10ms^(-2) )
View SolutionWhen a car of mass 1200 kg is moving with a velocity of 15 ms^(-1) on a rough horizontal road, its engine is switched off. How far does the car travel before it comes to rest if the coefficient of kinetic friction between the road and tyres of the car is 0.5? ( g=10ms^(-2) )
View SolutionA car of mass 1000 kg is moving with a velocity 20m /s on a horizontal straight road. If the driver applies brakes to produce a constant breaking force of 5000 Newton, the acceleration of the car i s ..... m / s^(2) .
View Solution