In a tringle ABC, AB = AC and bisector of angle A meets BC at D. Prove that :
(i) ΔABD≅ΔACD (ii) AD is perpendicular to BC.
To prove the statements given in the question, we will follow a systematic approach.
Given:
- Triangle ABC where AB = AC (isosceles triangle).
- AD is the bisector of angle A, meeting BC at point D.
To Prove:
1. Triangle ABD is congruent to triangle ACD (ΔABD ≅ ΔACD).
2. AD is perpendicular to BC (AD ⊥ BC).
Proof:
Part (i): Proving ΔABD ≅ ΔACD
1. Identify the sides and angles:
- We know that AB = AC (given).
- Since AD is the angle bisector, we have:
- ∠BAD = ∠CAD (by definition of angle bisector).
- The side AD is common to both triangles ABD and ACD:
- AD = AD.
2. Applying the Side-Angle-Side (SAS) Congruence Criterion:
- We have:
- Side AB = Side AC (1st condition).
- Angle ∠BAD = Angle ∠CAD (2nd condition).
- Side AD = Side AD (3rd condition).
- Therefore, by the SAS criterion, we can conclude:
- ΔABD ≅ ΔACD.
Part (ii): Proving AD ⊥ BC
1. Using the properties of isosceles triangles:
- Since AB = AC, it follows that angles opposite to equal sides are equal:
- ∠ABC = ∠ACB.
2. Using the angle bisector theorem:
- Since AD is the angle bisector, we have:
- ∠BAD = ∠CAD.
- Let ∠BAD = ∠CAD = x.
3. Summing the angles in triangle ABC:
- The sum of angles in triangle ABC is 180°:
- ∠ABC + ∠ACB + ∠A = 180°.
- Substituting the known angles:
- ∠ABC + ∠ABC + 2x = 180° (since ∠ABC = ∠ACB).
- This simplifies to:
- 2∠ABC + 2x = 180°.
- Dividing everything by 2 gives:
- ∠ABC + x = 90°.
4. Conclusion:
- Since ∠ABC + ∠CAD = 90°, we can conclude that:
- AD is perpendicular to BC (AD ⊥ BC).
Final Conclusion:
- We have proved that ΔABD ≅ ΔACD and that AD is perpendicular to BC.
---
- Triangle ABC where AB = AC (isosceles triangle).
- AD is the bisector of angle A, meeting BC at point D.
1. Triangle ABD is congruent to triangle ACD (ΔABD ≅ ΔACD).
2. AD is perpendicular to BC (AD ⊥ BC).
- We know that AB = AC (given).
- Since AD is the angle bisector, we have:
- ∠BAD = ∠CAD (by definition of angle bisector).
- The side AD is common to both triangles ABD and ACD:
- AD = AD.
- We have:
- Side AB = Side AC (1st condition).
- Angle ∠BAD = Angle ∠CAD (2nd condition).
- Side AD = Side AD (3rd condition).
- Therefore, by the SAS criterion, we can conclude:
- ΔABD ≅ ΔACD.
- Since AB = AC, it follows that angles opposite to equal sides are equal:
- ∠ABC = ∠ACB.
- Since AD is the angle bisector, we have:
- ∠BAD = ∠CAD.
- Let ∠BAD = ∠CAD = x.
- The sum of angles in triangle ABC is 180°:
- ∠ABC + ∠ACB + ∠A = 180°.
- Substituting the known angles:
- ∠ABC + ∠ABC + 2x = 180° (since ∠ABC = ∠ACB).
- This simplifies to:
- 2∠ABC + 2x = 180°.
- Dividing everything by 2 gives:
- ∠ABC + x = 90°.
- Since ∠ABC + ∠CAD = 90°, we can conclude that:
- AD is perpendicular to BC (AD ⊥ BC).
- We have proved that ΔABD ≅ ΔACD and that AD is perpendicular to BC.
Topper's Solved these Questions
- TRIANGLESBOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLESEXERCISE - Exercise 7b
15 Videos
- TRIANGLESBOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLESEXERCISE - Revision Exercise (very Short Answer Questions)
10 Videos
- TRIANGLESBOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLESEXERCISE - Problems From NCERT/exemplar
5 Videos
- SURFACE AREA AND VOLUMEBOOK - NAGEEN PRAKASHANCHAPTER - SURFACE AREA AND VOLUMEEXERCISE - Revision Exercise (long Answer Questions)
10 Videos
Similar Questions
Knowledge Check
In a △ABC,AB = AC and AD⊥BC, then :
AAB<ADBAB>ADCAB=ADDAB≤ADIn a ΔABC, BC = 9 cm, AC = 40 cm and AB = 41 cm. If bisector of angle A meets side BC at D then ratio of area of ΔABD and ΔABC is
A41:40B9:40C9:41D41:81In a △ABC,AD is the angle bisector of ∠CAB and a median to BC. If AC=15 cm, find AB.
AA)12 cmBB)18 cmCC)15 cmDD)can't be determined
Given a ΔABD in which AB=AD and AC bisects BD. Prove that :
ΔABC≅ΔADC.
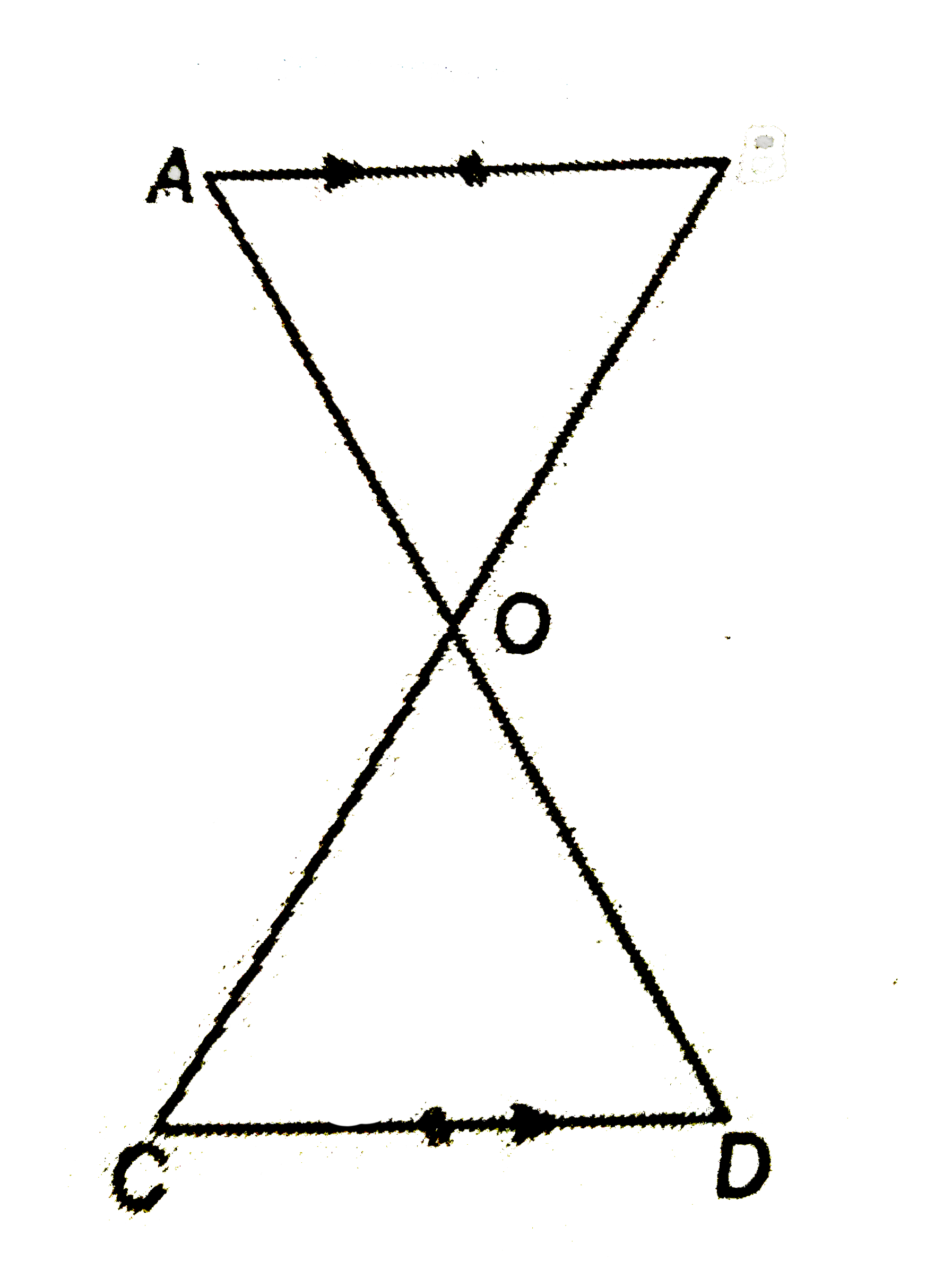
View SolutionIn the adjoining figure AB=CD and AB||CD prove that :
(i) ΔAOB≅ΔDOG
(ii) AD and BC bisect each other at point O.
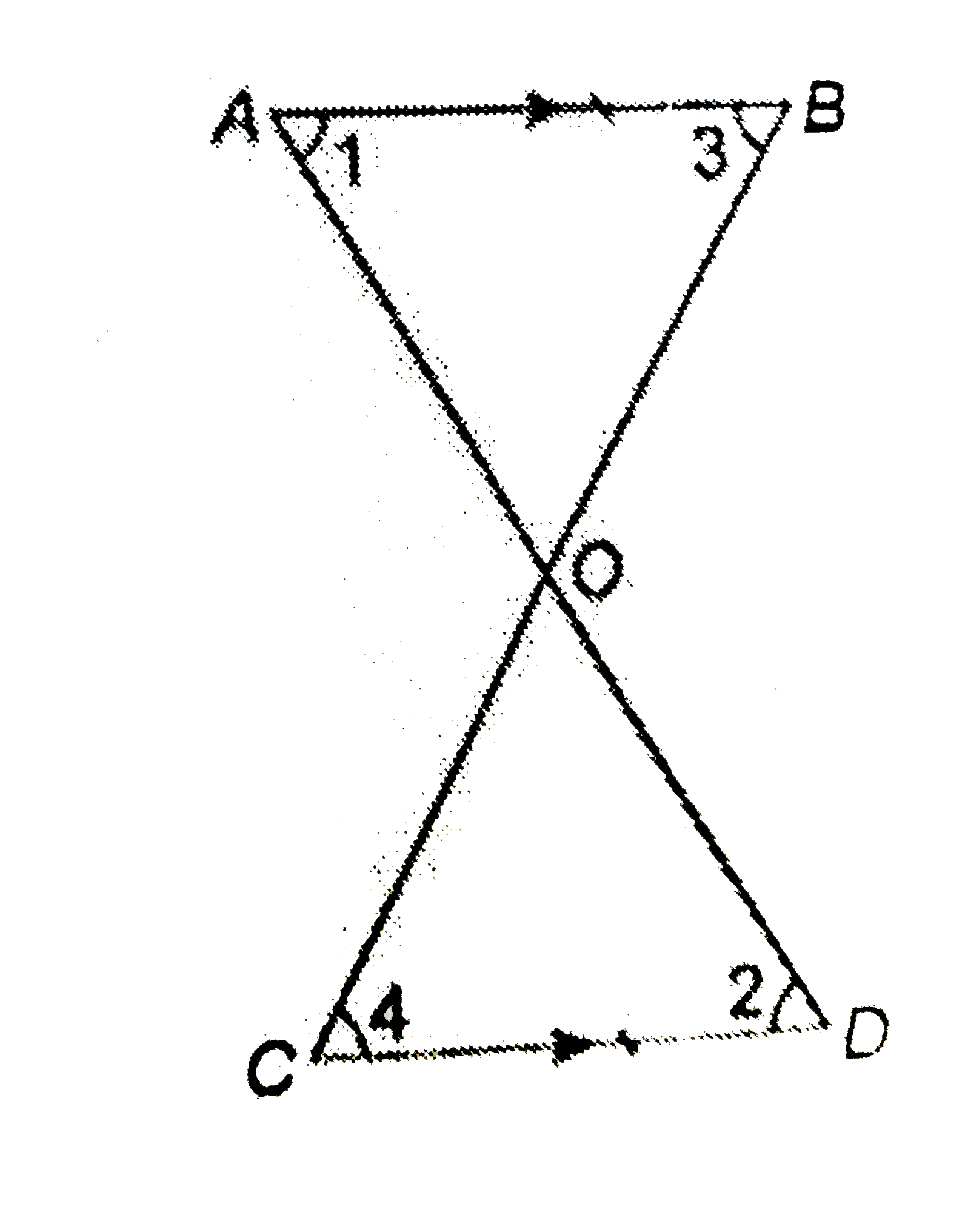
View SolutionIn the adjoining figure, AB = CD and AB||CD prove that
(i) ΔAOB≅ΔDOC
(ii) AD and BC bisect each other at the point O.
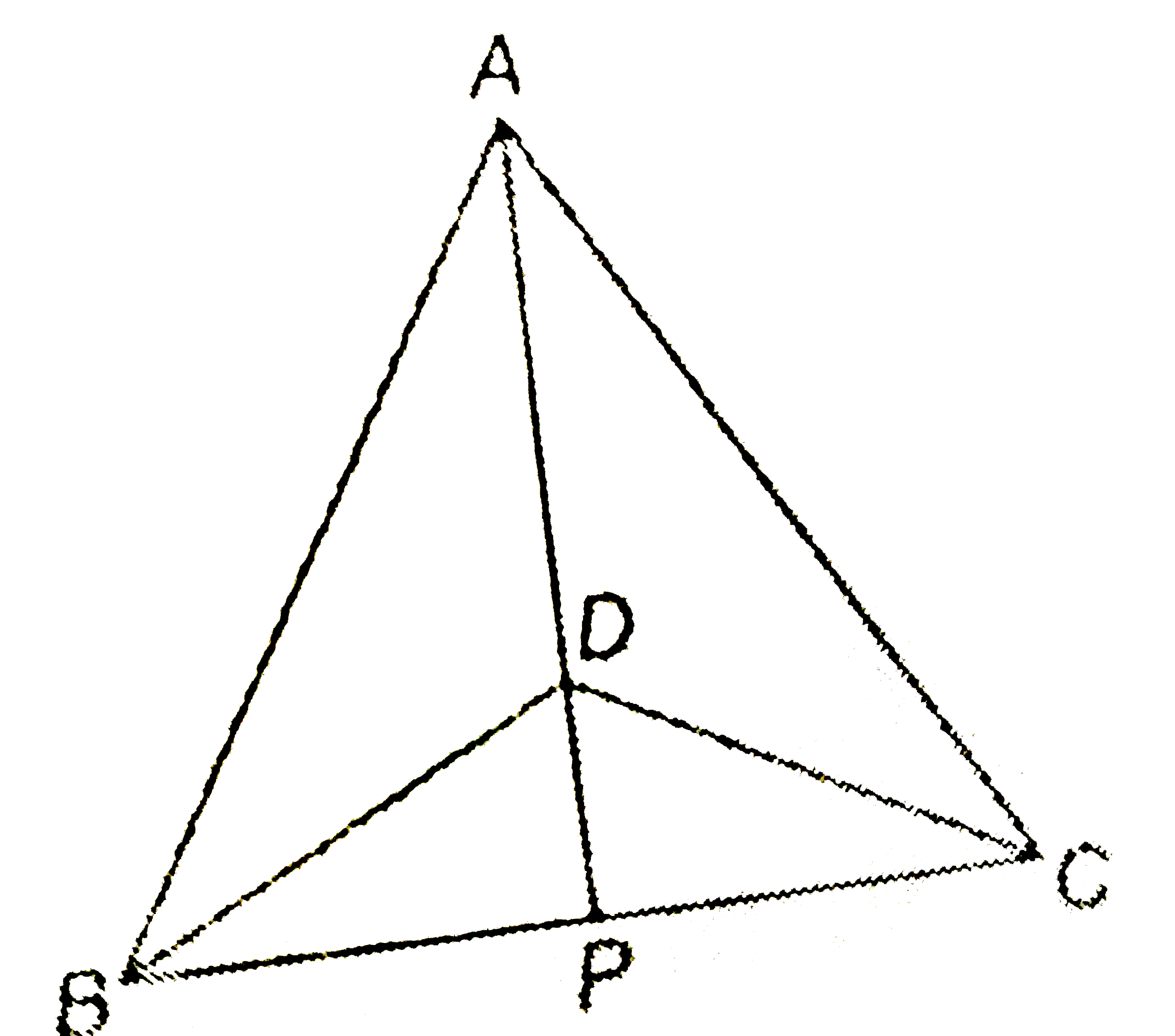
View SolutionΔABC and ΔDBC are two isosceles triangles on the same base BC and vertices A and D are on the same side of BC (see figure). If AD is extended to intersect BC at P, show that :
(i) ΔABD≅ΔACD
(ii) ΔABP≅ΔACP
(iii) AP bisects ∠A as well as ∠D
(iv) AP is the perpendicular bisector of BC.
View SolutionIn △ABC, AD is the internal bisector of ∠S, meeting the side BC at D. If BD = 5cm, BC = 7.5 cm, then AB:AC is:
View Solution
