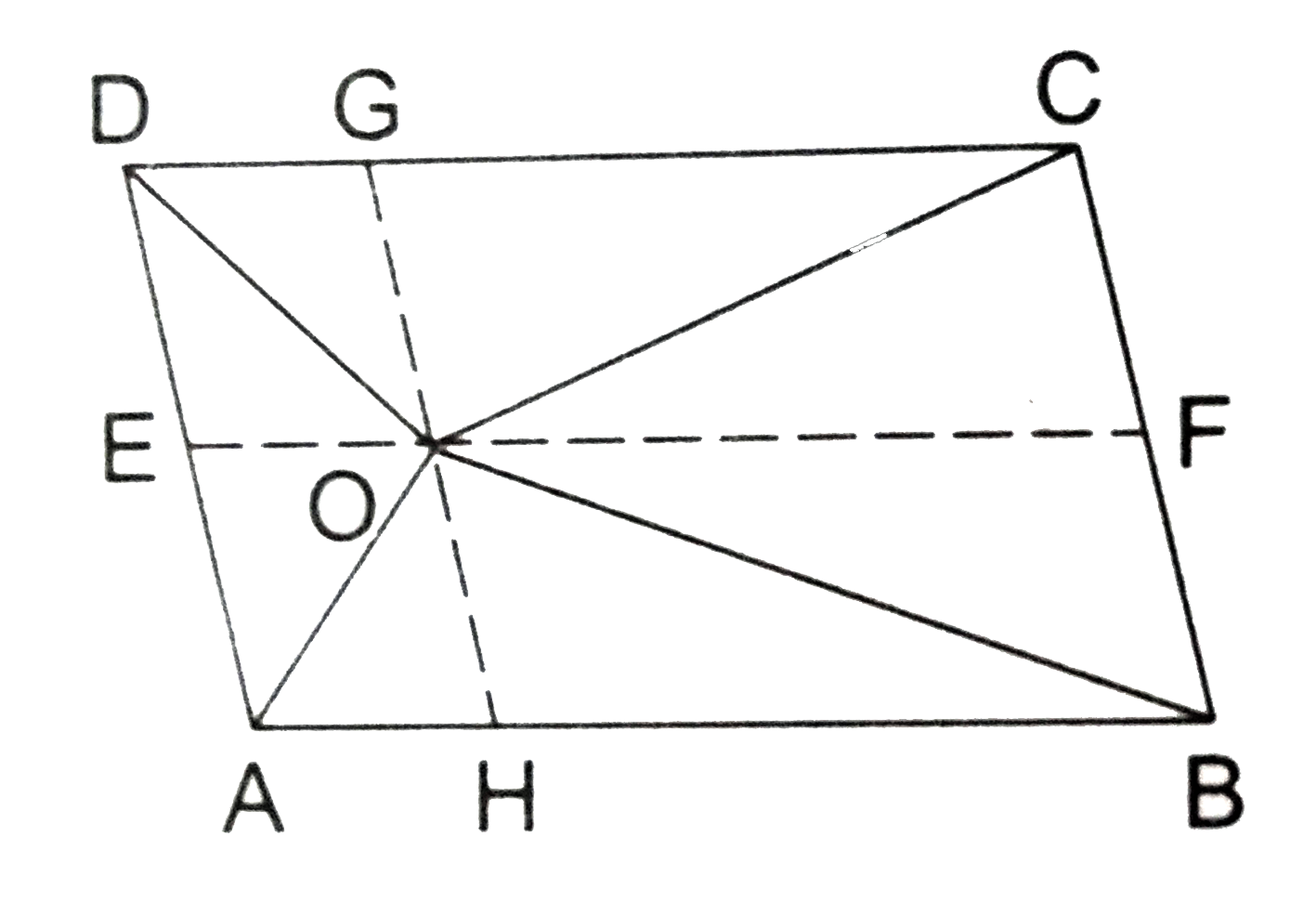
ABCD is a parallelogram. X and Y are mid-points of BC and CD. Prove that ar(△AXY)=38ar(∣∣gmABCD)
Join BD, BY and AC.
Since, X is the mid-point of BC.
∴ YX is the median for ΔCYB
∴Ar(ΔCYX)=12ar(ΔCYB)
(.: median divides the triangle into two equal area)
=12×12ar(ΔDBC)=14ar(ΔDBC)
(∴ median by divides the ΔDBC into two equal areas
=14×12ar(∣∣gmABCD)
(.: diagonal DB divides the ∣∣gm into two equal parts)
=18ar(∣∣gmABCD)....(1)
Now, ar(ΔABC)=12ar(∣∣gmABCD)
(.: diagonal divides the ∣∣gm into two triangle of equal areas)
2ar(ΔABX)=12ar(∣∣gmABCD)
(.: median AX divides the ΔABC into two equal parts)
⇒ar(ΔABX)=14ar(∣∣gmABCD)....(2)
Similarly, ar(ΔAYD)=14ar(∣∣gmABCD)....(3)
Now, using (1), (2) and (3), we get
ar(ΔAXY)=ar(∣∣gmABCD)−[ar(ΔABX)+ar(ΔAYD)+ar(ΔCYX)]
=ar(∣∣gmABCD)−(14+14+18)ar(∣∣gmABCD)
=ar(∣∣gmABCD)[2−58]=38ar(∣∣gmABCD)

Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Problems From NCERT/exemplar12videos
AREA OF PARALLELOGRAMS AND TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - AREA OF PARALLELOGRAMS AND TRIANGLESEXERCISE - Exercise34videos
AREA OF PARALLELOGRAMS AND TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - CIRCLE EXERCISE - Revision Exercise (long Answer Questions )5videos
CIRCLE
Similar Questions
Knowledge Check
- Question 1 - Select One
ABCD is a parallelogram, E and F are the mid-points of BC and CD. Find the ratio of area of parallelogram ABCD and ΔAEF
A2:1B4:1C8:3D8:1 - Question 2 - Select One
ABCD is a parallelogram and M is the mid point of BC. AB and DM are produced to meet at N, then :
AAN=√3ABBAN=2ABCAN2=32AB2DAN2=2AB2 - Question 3 - Select One
ABCD is a parallelogram and M is the mid point of BC. AB and DM are produced to meet at N, then :
AA)AN=√3ABBB)AN=2ABCC)AN2=32AB2DD)AN2=2AB2
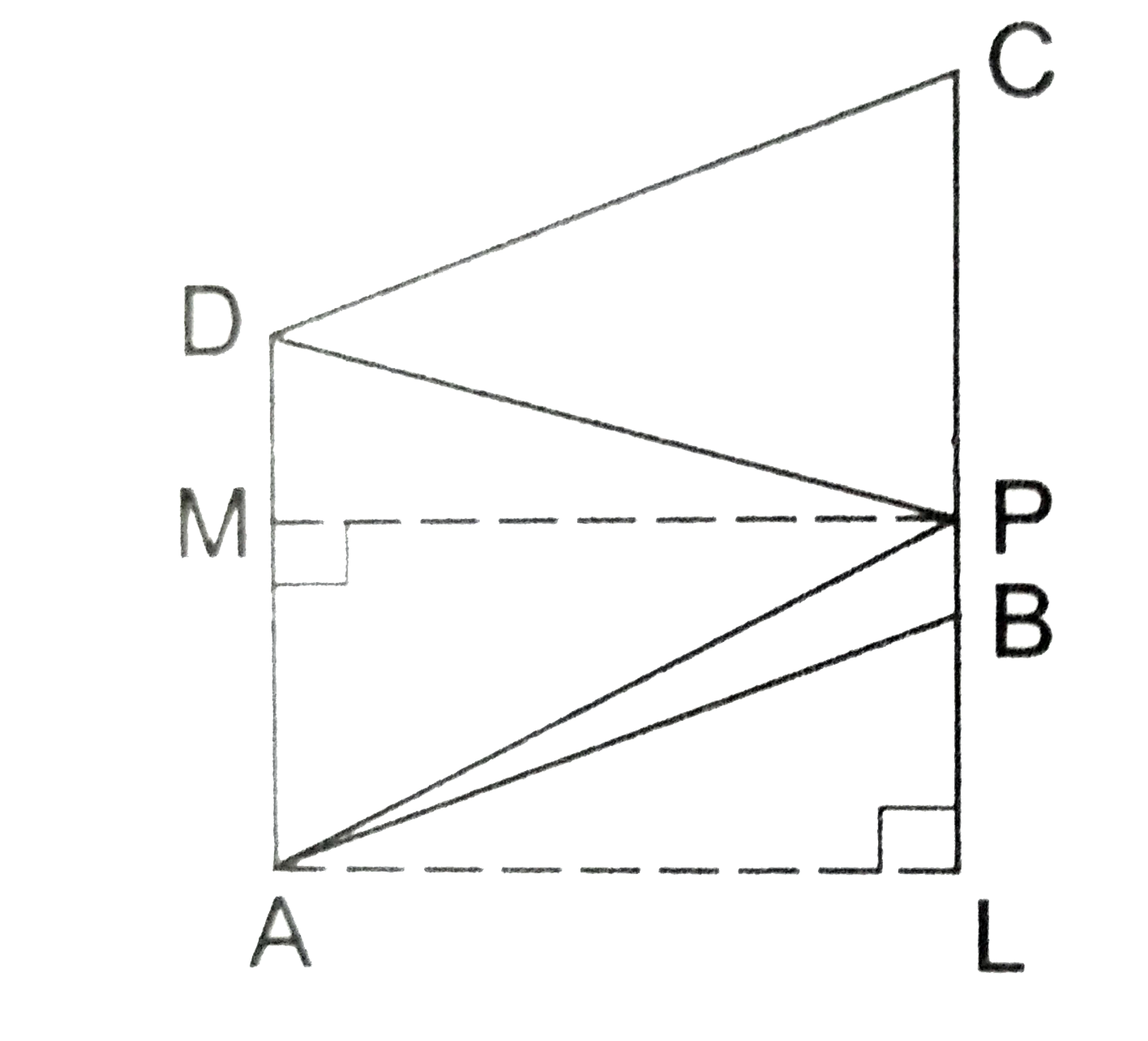
ABCD is a parallelogram and O is a point in its interior. Prove that
(i) ar(△AOB)+ar(△COD)
=12ar(||gm ABCD).
(ii) ar(△AOB)+ar(△COD)=ar(△AOD)+ar(△BOC).
 View Solution
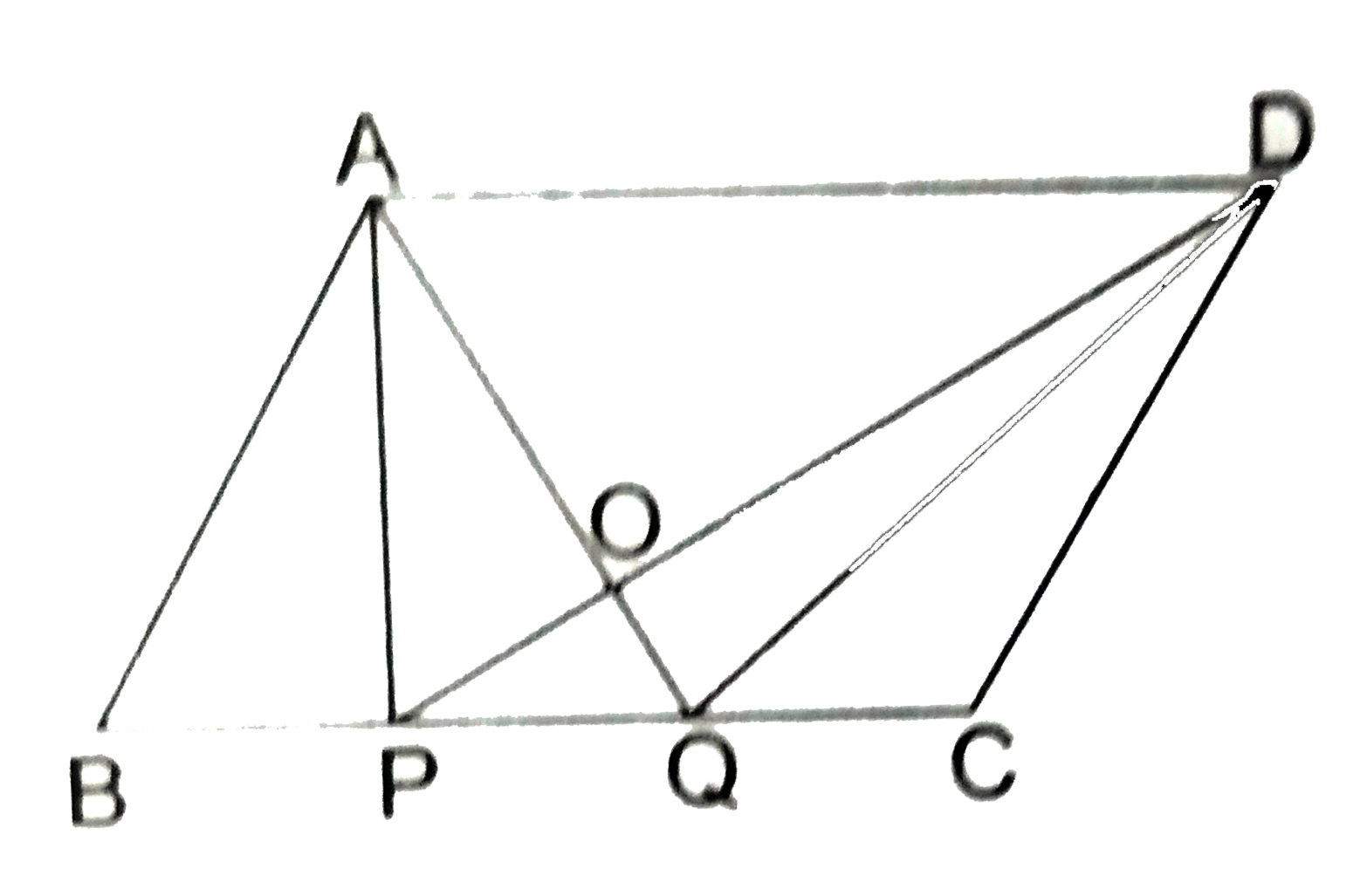
View SolutionIn the adjoining figure, ABCD is a parallelogram. Points P and Q on BC trisect BC. Prove that
ar(△APQ)=ar(△DPQ)=16ar(△ABCD).
 View Solution
View SolutionABCD is a parallelogram. P and Q are mid points of BC & CD. Find the area of ΔAPQ if area of ΔABC is 12.
View SolutionABCD is a parallelogram, E and F are the mid-points of AB and CD respectively. GH is any line intersecting AD, EF and BC at G, P and H respectively. Prove that GP=PH.
View SolutionA point E is taken on the side BC of a parallelogram ABCD. AE and DC are produced to meet at F. Prove that ar(ΔADF)=ar(ABFC).
View Solution