Using Biot Savart's law, derive the expression for the magnetic field at a point on the axis of a circular current loop.

Text Solution

Verified by Experts

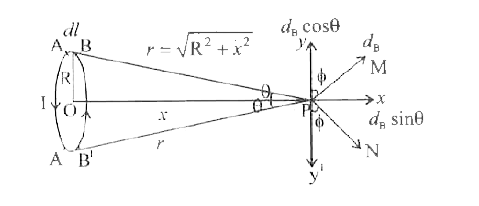
Consider a circular loop of radius R carrying a current I. Let P be a point at a distance x from the centre the , of the loop on axis. AB is current element of length dl. The distance of the point P from current element is r.
$∴$ From figure
$r = \sqrt{R^{2} + x^{2}} sin θ = \frac{R}{r}$
According to Biot-Savart.s law, magnetic field at the pont P due to the current element AB is
$d B = \frac{μ_{0}}{4 π} \frac{I d l}{r^{2}} sin ϕ$
Since $ϕ = 90^{\circ}$
$d B = \frac{μ_{0}}{4 π} \frac{I d l}{r^{2}}$ sin $90^{\circ}$ ( $∵$ sin $90^{\circ} = 1$ )
$d B = \frac{μ_{0}}{4 π} \frac{I d l}{r^{2}}$ along PM
dB due to the current element A.B. at the point P is $d B = \frac{μ_{0}}{4 π} \frac{I d l}{r^{2}}$ along PN.
The magnetic field dB along PM can be resolved into two rectangular components dB cos $ϕ$ along Py and dB sin $ϕ$ along Px. Similarly the magnetic field $d_{B}$ along PN can be resolved into two components .
If we consider more small elements cos $θ$ components cancel each other out .
$Σ d B cos ϕ = 0 α Σ d_{B} cos θ # 0$
$B = \int d B sin ϕ$
$B = \int \frac{μ_{0}}{4 π} \frac{I d l}{r^{2}} sin ϕ$
Substituting the values of $r = \sqrt{R^{2} + x^{2}}$ and sin $ϕ = \frac{R}{r}$ in the equation , we get
$B = \int \frac{μ_{0}}{4 π} \frac{I d l}{R^{2} + x^{2}} \frac{R}{r} = \int \frac{μ_{0}}{4 π} \frac{I d l}{R^{2} + x^{2}} \frac{R}{\sqrt{R^{2} + x^{2}}} = \frac{μ_{0}}{4 π} \frac{I R}{{(R^{2} + x^{2})}^{3 / 2}} \int d l$
But $\int d l = 2 π R \to$ circumference of the loop
$∴ B = \frac{μ_{0} I R}{4 π {(x^{2} + R^{2})}^{3 / 2}} \times 2 π R$
$∴ B = \frac{μ_{0}}{4 π} \frac{2 π I R^{2}}{{(x^{2} + R^{2})}^{3 / 2}}$

Updated on:21/07/2023

|

Class 12 PHYSICS SUPER MODEL QUESTION PAPER 1

Topper's Solved these Questions

Similar Questions

SUBHASH PUBLICATION-SUPER MODEL QUESTION PAPER 1-QUESTION

Home
Ask Doubt
Profile