There exists a uniform magnetic field ¯¯¯B=B0t¯k in a region. A circular conducting loop of radius r and resistance R is placed with its plane in x-y plane. Determine the current through the loop and sense of the current.
To solve the problem of determining the current through a circular conducting loop placed in a uniform magnetic field, we will follow these steps:
Step 1: Identify the Magnetic Field and Loop Orientation
The magnetic field is given as ¯B=B0t^k, where B0 is a constant, t is time, and ^k is the unit vector in the z-direction. The circular loop lies in the x-y plane.
Step 2: Calculate the Magnetic Flux
The magnetic flux Φ through the loop is given by:
Φ=¯B⋅¯A
where ¯A is the area vector of the loop. The area of the circular loop is A=πr2 and its area vector points in the direction of ^k. Thus, we can write:
Φ=B⋅A=B0t⋅(πr2)=B0tπr2
Step 3: Apply Faraday's Law of Electromagnetic Induction
According to Faraday's law, the induced electromotive force (EMF) E in the loop is given by the negative rate of change of magnetic flux:
E=−dΦdt
Substituting the expression for Φ:
E=−ddt(B0tπr2)=−B0πr2
Step 4: Calculate the Induced Current
Using Ohm's law, the current I through the loop can be calculated as:
I=ER
Substituting the expression for E:
I=−B0πr2R
The negative sign indicates the direction of the induced current according to Lenz's law.
Step 5: Determine the Sense of the Current
According to Lenz's law, the direction of the induced current will be such that it opposes the change in magnetic flux. Since the magnetic field is increasing in the positive z-direction, the induced current will flow in a direction that creates a magnetic field opposing this increase. Therefore, the current will flow in a counterclockwise direction when viewed from above (the positive z-axis).
Final Answer
The current through the loop is:
I=B0πr2R
and the sense of the current is counterclockwise when viewed from the positive z-axis.
---
The magnetic field is given as ¯B=B0t^k, where B0 is a constant, t is time, and ^k is the unit vector in the z-direction. The circular loop lies in the x-y plane.
The magnetic flux Φ through the loop is given by:
Φ=¯B⋅¯A
where ¯A is the area vector of the loop. The area of the circular loop is A=πr2 and its area vector points in the direction of ^k. Thus, we can write:
Φ=B⋅A=B0t⋅(πr2)=B0tπr2
According to Faraday's law, the induced electromotive force (EMF) E in the loop is given by the negative rate of change of magnetic flux:
E=−dΦdt
Substituting the expression for Φ:
E=−ddt(B0tπr2)=−B0πr2
Using Ohm's law, the current I through the loop can be calculated as:
I=ER
Substituting the expression for E:
I=−B0πr2R
The negative sign indicates the direction of the induced current according to Lenz's law.
According to Lenz's law, the direction of the induced current will be such that it opposes the change in magnetic flux. Since the magnetic field is increasing in the positive z-direction, the induced current will flow in a direction that creates a magnetic field opposing this increase. Therefore, the current will flow in a counterclockwise direction when viewed from above (the positive z-axis).
The current through the loop is:
I=B0πr2R
and the sense of the current is counterclockwise when viewed from the positive z-axis.
Similar Questions
A uniform magnetic field B=B0tˆi in a region exists. A circular conducting loop of radius r and resistance R is placed with its plane in yz-plane. Determine the current through the loop and sense of the current.
View SolutionA space is divided by the line AD into two regions. Region I is field free and the region II has a uniform magnetic field B directed into the paper. ACD is a semicircular conducting loop of radius r with centre at O, the plane of the loop being in the plane of the paper. The loop is now made to rotate with a constant angular velocity about an axis passing through O, and perpendicular to the plane of the paper in the clockwise direction. The effective resistance of the loop is R.
Show the direction of the current when the loop is entering into the region II.
View Solution
Knowledge Check
A circular loop of radius r, carrying a current I lines in y - z plane with its centre at the origin. The net magnetic flux through the loop is
Adirectly proportional to r.BzeroCinversely proportional to r.Ddirectly proportional to I.A circular loop of radius R , carrying I, lies in x−y plane with its origin . The total magnetic flux through x−y plane is
Adirectly proportional to IBdirectly proportional to RCinversly proportional to RDzeroIn the figure shown there exists a uniform time varying magnetic field B=[(4T/s)t+0.3T] in a cylinderical region of radius 4m. An equilateral traingualr conducting loop is placed in the magnetic field with its centroide on the axis of the field and its plane perpendicular to the field.
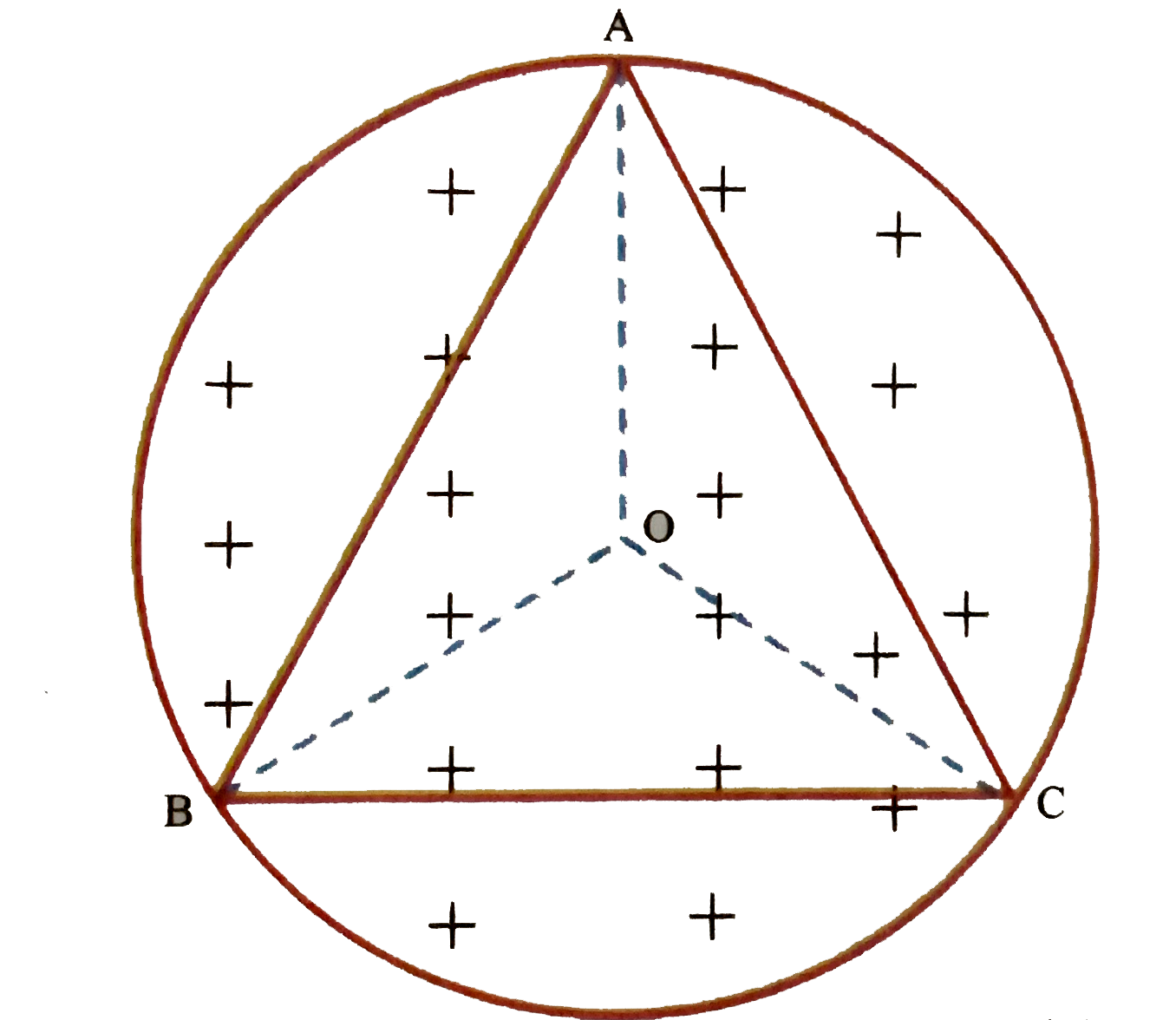 Ae.m.f. induced in any one rod is 16VBe.m.f. incduced in the complete ΔABC is 48√3VCe.m.f. induced in the complete ΔABC is 48VDe.m.f. induced in any one rod is 16√3V
Ae.m.f. induced in any one rod is 16VBe.m.f. incduced in the complete ΔABC is 48√3VCe.m.f. induced in the complete ΔABC is 48VDe.m.f. induced in any one rod is 16√3V
A space is divided by the line AD into two regions. Region I is field free and the region II has a uniform magnetic field B directed into the paper. ACD is a semicircular conducting loop of radius r with centre at O, the plane of the loop being in the plane of the paper. The loop is now made to rotate with a constant angular velocity about an axis passing through O, and perpendicular to the plane of the paper in the clockwise direction. The effective resistance of the loop is R.
Obtain an expression for the magnitude of the induced current in the loop.
View SolutionA space is divided by the line AD into two regions. Region I is field free and the region II has a uniform magnetic field B directed into the paper. ACD is a semicircular conducting loop of radius r with centre at O, the plane of the loop being in the plane of the paper. The loop is now made to rotate with a constant angular velocity about an axis passing through O, and perpendicular to the plane of the paper in the clockwise direction. The effective resistance of the loop is R.
Plot a graph between the induced emf and the time of rotation for two periods of rotation.
View SolutionWhat will be magnetic field at centre of current carrying circular loop of radius R?
View SolutionMagnetic field B in a cylindrical region of radius r varies according to the law B=B0t as shown in the figure. A fixed conducting loop ABCDA of resistance R is lying in the region as show . The current flowing through the loop is
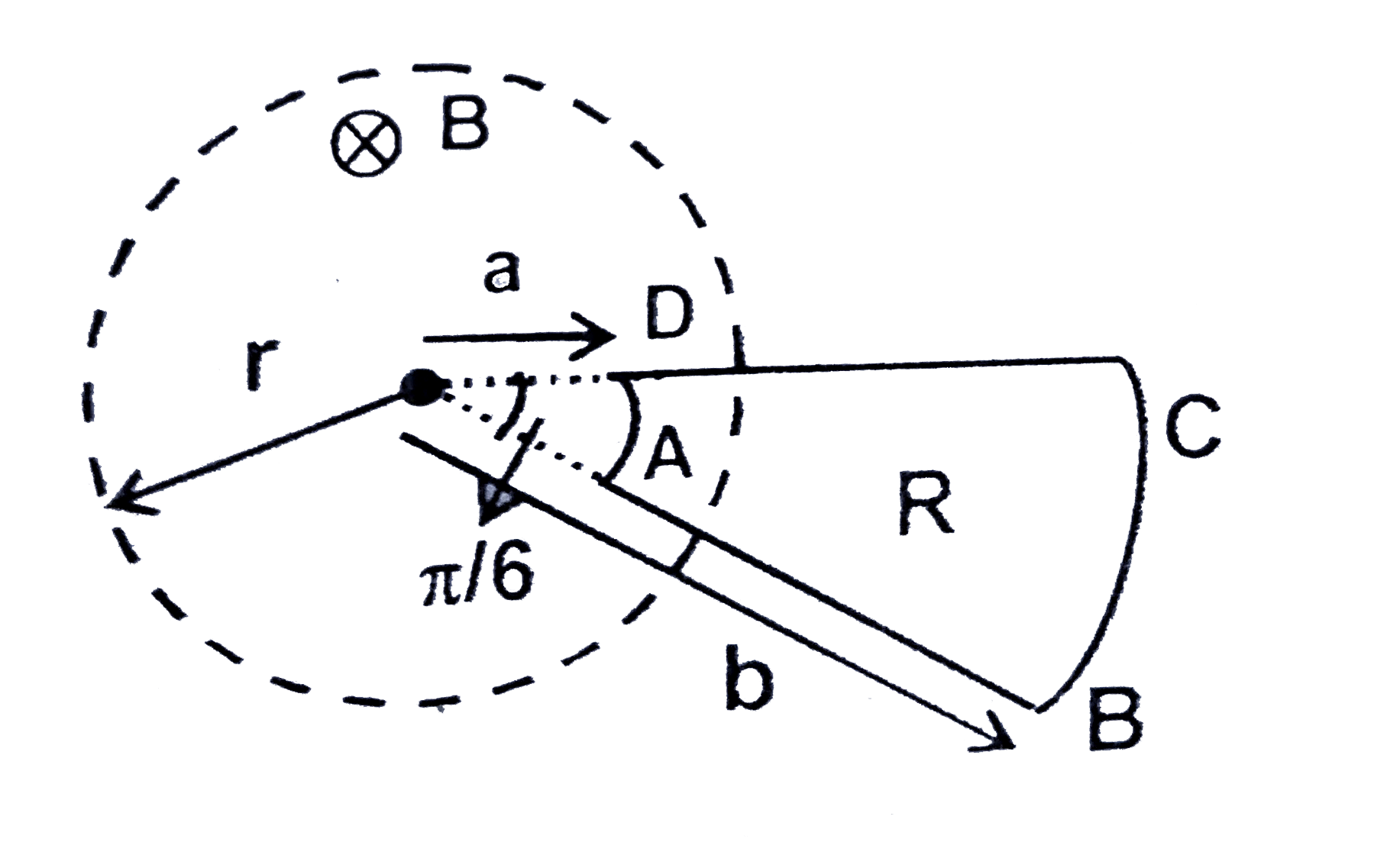
View SolutionAn external magnetic field is decreased to zero, due to which a current is induced in a circular wire loop of radius r and resistance R placed in the field. This current will not become zero at the instant when B stops changing
View Solution
