A space is divided by the line AD into two regions. Region I is field free and the region II has a uniform magnetic field B directed into the paper. ACD is a semicircular conducting loop of radius r with centre at O, the plane of the loop being in the plane of the paper. The loop is now made to rotate with a constant angular velocity about an axis passing through O, and perpendicular to the plane of the paper in the clockwise direction. The effective resistance of the loop is R.
Obtain an expression for the magnitude of the induced current in the loop.
To solve the problem, we need to find the expression for the induced current in a semicircular conducting loop rotating in a magnetic field. Here are the steps to derive the expression:
Step 1: Understand the Setup
We have a semicircular conducting loop (ACD) of radius r rotating with a constant angular velocity ω in a uniform magnetic field B directed into the paper. The loop is rotating about an axis perpendicular to the plane of the paper.
Step 2: Determine the Area of the Loop in the Magnetic Field
As the loop rotates, the angle θ that the loop has rotated at time t is given by:
θ=ωt
The area A of the semicircular loop that is within the magnetic field can be expressed as:
A=12r2θ=12r2(ωt)
Step 3: Calculate the Magnetic Flux
The magnetic flux Φ through the loop is given by:
Φ=B⋅A=B⋅(12r2ωt)=Br2ωt2
Step 4: Find the Induced EMF
According to Faraday’s law of electromagnetic induction, the induced electromotive force (EMF) E is given by the negative rate of change of magnetic flux:
E=−dΦdt
Calculating the derivative:
E=−ddt(Br2ωt2)=−Br2ω2
Thus, the induced EMF is:
E=−Br2ω2
Step 5: Calculate the Induced Current
Using Ohm's law, the induced current I can be calculated as:
I=ER
Substituting the expression for E:
I=−Br2ω2R=−Br2ω2R
The magnitude of the induced current is:
I=Br2ω2R
Final Expression
The magnitude of the induced current in the loop is:
I=Br2ω2R
We have a semicircular conducting loop (ACD) of radius r rotating with a constant angular velocity ω in a uniform magnetic field B directed into the paper. The loop is rotating about an axis perpendicular to the plane of the paper.
As the loop rotates, the angle θ that the loop has rotated at time t is given by:
θ=ωt
The area A of the semicircular loop that is within the magnetic field can be expressed as:
A=12r2θ=12r2(ωt)
The magnetic flux Φ through the loop is given by:
Φ=B⋅A=B⋅(12r2ωt)=Br2ωt2
According to Faraday’s law of electromagnetic induction, the induced electromotive force (EMF) E is given by the negative rate of change of magnetic flux:
E=−dΦdt
Calculating the derivative:
E=−ddt(Br2ωt2)=−Br2ω2
Thus, the induced EMF is:
E=−Br2ω2
Using Ohm's law, the induced current I can be calculated as:
I=ER
Substituting the expression for E:
I=−Br2ω2R=−Br2ω2R
The magnitude of the induced current is:
I=Br2ω2R
The magnitude of the induced current in the loop is:
I=Br2ω2R
Topper's Solved these Questions
- BOOK - MOTIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE-125videos
ELECTROMAGNETIC INDUCTION
- BOOK - MOTIONCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - EXERCISE-2 (LEVEL-I)16videos
ELECTROMAGNETIC INDUCTION
- BOOK - MOTIONCHAPTER - ELECTRO MAGNETIC WAVES EXERCISE - EXERCISE - 3 (SECTION - B)8videos
ELECTRO MAGNETIC WAVES
- BOOK - MOTIONCHAPTER - ELECTROMAGNETISMEXERCISE - Exercise136videos
ELECTROMAGNETISM
Similar Questions
A flux of 1m Wb passes through a strip having an area A=0.02m2. The plane of the strip is at an angle of 60∘ to the direction of a uniform field B. The value of B is-
View SolutionThe dimension of the ratio of magnetic flux and the resistance is equal to that of:
11:49View SolutionWhich of the following is not an electromagnetic wave?
01:56View SolutionThe variation of electric potential with distance from a fixed point is shown in figure. What is the value of electric field at x=2m -
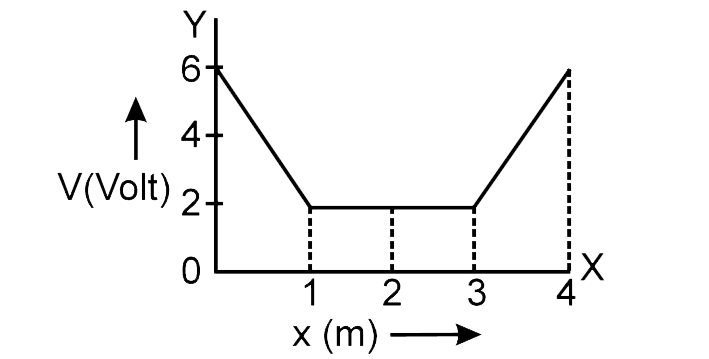 02:15View Solution
02:15View SolutionA wire loop confined in a plane is rotated in its oen plane with some angular velocity. A uniform magnetic field exists in the region. Fid the emf induced in the loop.
05:59View SolutionSpace is divided by the line AD into two regions. Region I is field free and the Region II has a unifrom magnetic field B direction into the plane of the paper. ACD is a simicircular conducting loop of radius r with center at O, hte plane of the loop being in the plane of the paper. The loop is now made to rotate with a constant angular velocity omega about an axis passing through O and the perpendicular to the plane of the paper. The effective resistance of the loop is R. (i) obtain an expression for hte magnitude of the induced cureent in the loop. (ii) Show the direction of the current when the loop is entering into the Rigion II. Plot a graph between the induced e.m.f and the time of roation for two periods or rotation.
05:59View SolutionA square loop of wire carrying current I is lying in the plane of paper as shown in Fig. 1.141. The magnetic field is present in the region as shown. The loop will tend to rotate
 10:35View Solution
10:35View SolutionThere is a uniform magnetic field directed perpendicular and into the plane of the paper. An irregular shaped conducting loop is slowly changing into a circular loop in the plane of the paper. Then
07:40View SolutionA space is divided by the line AD into two regions. Region I is field free and the region II has a uniform magnetic field B directed into the paper. ACD is a semicircular conducting loop of radius r with centre at O, the plane of the loop being in the plane of the paper. The loop is now made to rotate with a constant angular velocity about an axis passing through O, and perpendicular to the plane of the paper in the clockwise direction. The effective resistance of the loop is R. Show the direction of the current when the loop is entering into the region II.
03:56View SolutionA space is divided by the line AD into two regions. Region I is field free and the region II has a uniform magnetic field B directed into the paper. ACD is a semicircular conducting loop of radius r with centre at O, the plane of the loop being in the plane of the paper. The loop is now made to rotate with a constant angular velocity about an axis passing through O, and perpendicular to the plane of the paper in the clockwise direction. The effective resistance of the loop is R. Plot a graph between the induced emf and the time of rotation for two periods of rotation.
01:38View SolutionA uniform circular loop of radius a and resistance R is pulled at a constant velocity v out of a region of a uniform plane of the loop and the velocity are both perpendicular to B. Then the electrical power in the circular loop at the instant when the arc (of the circular loop) outside the region of magnetic field subtends an angle (pi)/(3) at the centre of the loop is
02:21View SolutionThe magnetic field B shown in is directed into the plane of the paper. ACDA is a semicircular conducting loop of radius r with the centre at O. The loop is now made ot rotate clockwise with a constant angular velocity ω about on axis passing through O and perpendicular to the plane of the paper. The resistance of the loop is R. Obtain an expression for the magnitude of the induced current in the loop. Plot a graph between the induced current i and ωt, for two periods of rotation.
 02:52View Solution
02:52View SolutionA wire loop confined in a plane is rotated in its open plane with some angular velocity. A uniform magnetic field exists in the region. Find the emf induced in the loop.
09:07View SolutionThere is a uniform magnetic field directed perpendicular and into the plane of the paper. An irregular shaped conducting loop is slowly changing into a circular loop in the plane of the paper. Then
09:14View SolutionThere is a uniform magnetic field directed perpendicular and into the plane of the paper. An irregular shaped conducting loop is slowly changing into a circular loop in the plane of the paper. Then
06:12View Solution

