Radius ol a circular luop placed in a perpendicular uniform magnetic field is increasing at a constant rate of roms1. If at any instant radius of the loop is r, then emf induced in the loop at that instant will be
−2brr0
−2Bπr
−Bπr0r
−2Bπr0r
The correct Answer is:D
To solve the problem, we need to find the induced EMF in a circular loop whose radius is increasing at a constant rate in a uniform magnetic field. We will use Faraday's law of electromagnetic induction, which states that the induced EMF (ε) in a closed loop is equal to the negative rate of change of magnetic flux (Φ) through the loop.
1. Identify the Variables:
- Let the radius of the circular loop at any instant be r.
- The area A of the circular loop is given by the formula:
A=πr2
- The magnetic field B is uniform and perpendicular to the loop.
2. Calculate the Magnetic Flux (Φ):
- The magnetic flux Φ through the loop is given by:
Φ=B⋅A=B⋅(πr2)
3. Differentiate the Magnetic Flux with Respect to Time:
- To find the induced EMF, we need to differentiate the magnetic flux with respect to time t:
dΦdt=ddt(B⋅πr2)
- Since B is constant, we can take it out of the differentiation:
dΦdt=B⋅π⋅d(r2)dt
4. Apply the Chain Rule:
- Using the chain rule, we differentiate r2:
d(r2)dt=2rdrdt
- Given that drdt=r0 (the rate at which the radius is increasing), we can substitute this into our equation:
d(r2)dt=2r⋅r0
5. Substitute Back into the Flux Derivative:
- Now substituting back into the expression for dΦdt:
dΦdt=B⋅π⋅(2r⋅r0)=2πBrr0
6. Calculate the Induced EMF (ε):
- According to Faraday's law:
ε=−dΦdt
- Therefore, the induced EMF is:
ε=−2πBrr0
Final Answer:
The induced EMF in the loop at that instant is:
ε=−2πBrr0
- Let the radius of the circular loop at any instant be r.
- The area A of the circular loop is given by the formula:
A=πr2
- The magnetic field B is uniform and perpendicular to the loop.
- The magnetic flux Φ through the loop is given by:
Φ=B⋅A=B⋅(πr2)
- To find the induced EMF, we need to differentiate the magnetic flux with respect to time t:
dΦdt=ddt(B⋅πr2)
- Since B is constant, we can take it out of the differentiation:
dΦdt=B⋅π⋅d(r2)dt
- Using the chain rule, we differentiate r2:
d(r2)dt=2rdrdt
- Given that drdt=r0 (the rate at which the radius is increasing), we can substitute this into our equation:
d(r2)dt=2r⋅r0
- Now substituting back into the expression for dΦdt:
dΦdt=B⋅π⋅(2r⋅r0)=2πBrr0
- According to Faraday's law:
ε=−dΦdt
- Therefore, the induced EMF is:
ε=−2πBrr0
The induced EMF in the loop at that instant is:
ε=−2πBrr0
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBook:AAKASH INSTITUTEChapter:ELECTROMAGNETIC INDUCTIONExercise:ASSIGNMENT(SECTION - A) (OBJECTIVE TYPE QUESTIONS)
Explore 30 Videos
- ELECTROMAGNETIC INDUCTIONBook:AAKASH INSTITUTEChapter:ELECTROMAGNETIC INDUCTIONExercise:ASSIGNMENT(SECTION - B) (OBJECTIVE TYPE QUESTIONS)
Explore 11 Videos
- ELECTROMAGNETIC INDUCTIONBook:AAKASH INSTITUTEChapter:ELECTROMAGNETIC INDUCTIONExercise:Assignment (SECTION - J)
Explore 4 Videos
- ELECTRIC CHARGES AND FIELDS Book:AAKASH INSTITUTEChapter:ELECTRIC CHARGES AND FIELDS Exercise:SECTION -J(Aakash Challengers Questions)
Explore 5 Videos
- ELECTROMAGNETIC WAVESBook:AAKASH INSTITUTEChapter:ELECTROMAGNETIC WAVESExercise:ASSIGNMENT SECTION - D Assertion-Reason Type Questions
Explore 25 Videos
Similar Questions
A certain elastic conducting material is stretched into a circular loop of 12.0 cm radius. It is placed with its plane perpendicular to a uniform 0.800 T magnetic field. When released, the radius of the loop starts to shrink at an instantaneous rate of 75.0 emfs. What emf is induced in the loop at that instant?
View SolutionA conducting cirular loop is placed in a unifrom transcerse magnetic field of 0.02 T . Somehow , the radius of the loop begins to decrease at a constant rate of 1. 0 mm/s . Find the emf induced in the loop at the instant when the radius is 2 cm.
View Solution
Knowledge Check
Radius of a circular loop placed in a perpendicular uniform magnetic field is increasing at a constant rate of roms−1 If at any instant radius of the loop is r, then emf induced in the loop at that instant will be
A−2Brr0B−2BπrC−Bπrr0D−2Bπrr0Metal ring of radius R is placed perpendicular to uniform magnetic field B. Magnetic field starts changing at a rate α
AElectric field at any point on the ring is zero.BElectric field at any point on the ring is α(R)/(2)CEmf induced in the ring is zeroDEmf induced in the ring is πaR2A circular loop is kept perpendicular to a uniform magnetic field. Loop is rotated about its diameter with constant angular velocity.
AMagnitude of emf induced will be minimum when magnitude of flux is maximumBMagnitude of emf is maximum when magnitude of flux is minimumCPhase difference between emf and flux is π2DNone of these
A metallic loop is placed in a nonuiform magnetic field. Will an emf be induced in the loop?
View SolutionA uniform magnetic field B exists in a circular region of radius R. the field is perpendicular to the plane and is increasing at a constant rate of dBdt=α. There is a straight conducing rod AB of length 2R. Find the emf induced in the rod when it is placed as shown in figure (a) & (b). Point C is midpoint of the rod in figure (b)
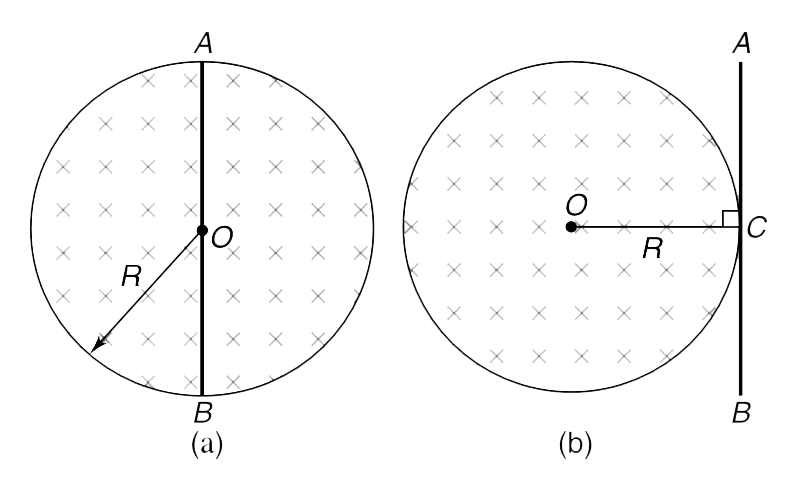
View SolutionA conducing circular loop is placed in a uniform magnetic field of 0.02 T, woth its plane perpendicular to the field. If the redius of the loop starts shrinking at a constant rate of 1.0mm/s, then find the emf induced in the loop, at the instant when radius is 4 cm.
View SolutionA conduction circular loop is placed in a uniform magnetic field 0.02T with its place normal to the field . The radius of loop starts shrinking at a rate of 1.0mm/sec. Then induced emf in the loop at an instant when the radius is 2 cm :-
View SolutionA conducing circular loop is placed in a uniform magnetic field of indution B tesla with its plane normal to the field. Now, radius of the loop starts shrinking at the rate (dr/dt). Then the induced e.m.f. at the instant when the radius is r is:
View Solution
