For a reaction of type
is equal to
The correct Answer is:C
To solve the question regarding the reaction of type aA+bB→products and to express −d(A)dt in terms of the stoichiometric coefficients, we can follow these steps:
1. Understanding the Reaction:
The reaction is given as aA+bB→products. Here, A and B are the reactants, and a and b are their respective stoichiometric coefficients.
2. Rate of Reaction:
The rate of reaction can be expressed in terms of the change in concentration of the reactants over time. For reactant A, the rate of disappearance is given by:
−d(A)dt
For reactant B, the rate of disappearance is:
−d(B)dt
3. Relating Rates to Stoichiometry:
The relationship between the rates of disappearance of the reactants and the formation of products can be expressed using their stoichiometric coefficients:
−1ad(A)dt=−1bd(B)dt
This means that the rate of disappearance of A is proportional to its stoichiometric coefficient a, and similarly for B with coefficient b.
4. Expressing −d(A)dt:
Rearranging the above equation gives:
−d(A)dt=ab(−d(B)dt)
This indicates that the rate of disappearance of A can be expressed in terms of the rate of disappearance of B and their stoichiometric coefficients.
5. Final Expression:
Thus, the expression for −d(A)dt in terms of the concentration change of B is:
−d(A)dt=ab(−d(B)dt)
The reaction is given as aA+bB→products. Here, A and B are the reactants, and a and b are their respective stoichiometric coefficients.
The rate of reaction can be expressed in terms of the change in concentration of the reactants over time. For reactant A, the rate of disappearance is given by:
−d(A)dt
For reactant B, the rate of disappearance is:
−d(B)dt
The relationship between the rates of disappearance of the reactants and the formation of products can be expressed using their stoichiometric coefficients:
−1ad(A)dt=−1bd(B)dt
This means that the rate of disappearance of A is proportional to its stoichiometric coefficient a, and similarly for B with coefficient b.
Rearranging the above equation gives:
−d(A)dt=ab(−d(B)dt)
This indicates that the rate of disappearance of A can be expressed in terms of the rate of disappearance of B and their stoichiometric coefficients.
Thus, the expression for −d(A)dt in terms of the concentration change of B is:
−d(A)dt=ab(−d(B)dt)
Topper's Solved these Questions
- CHEMICAL KINETICSBOOK - AAKASH INSTITUTECHAPTER - CHEMICAL KINETICSEXERCISE - ASSIGNMENT (SECTION B : Objective Type Questions)
20 Videos
- CHEMICAL KINETICSBOOK - AAKASH INSTITUTECHAPTER - CHEMICAL KINETICSEXERCISE - ASSIGNMENT (SECTION C : Previous Year Questions)
62 Videos
- CHEMICAL KINETICSBOOK - AAKASH INSTITUTECHAPTER - CHEMICAL KINETICSEXERCISE - EXERCISE
30 Videos
- CHEMICAL BONDING AND MOLECULAR STRUCTURE BOOK - AAKASH INSTITUTECHAPTER - CHEMICAL BONDING AND MOLECULAR STRUCTURE EXERCISE - Assignment Section J (Aakash Challengers Questions)
10 Videos
- CHEMISTRY IN EVERYDAY LIFE BOOK - AAKASH INSTITUTECHAPTER - CHEMISTRY IN EVERYDAY LIFE EXERCISE - Assignment ( SECTION - A)
45 Videos
Similar Questions
Knowledge Check
In the reaction 3A→2B, rate of reaction +d[B]dt is equal to :
A−32d[A]dtB−23d[A]dtC−13d[A]dtD+2d[A]dtIn the reaction, 3A → 2B rate of reaction, +d[B]dt is equal to
A−13d[A]dtB−23d[A]dtC+2d[A]dtD−32d[A]dtIn the reaction 3A→2B, rate of reaction +d[B]dt is equal to :
A13d[A]dtB23d[A]dtC+2d[A]dtD32d[A]dt
3A→2B, rate of reaction +d[B]dt is equal to:
View SolutionIn the reaction 3A→2B, rate of reaction +d[B]dt is equal to :
View SolutionThe initial rate, −d[A]dt at t=o was found to be 2.6×102 mol L−1s_1 for the reaction
A+2B→products
The initial rate, −d[B]dt, at t=o is
View SolutionFor the reaction A+B+C→ products , the term (−d[C]/dt) in rate equation refers to
View SolutionFor the reaction A→ Product, −d[A]dt=k and at different time interval, [A] values are :
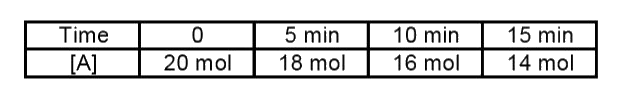
At 20 minute, rate will be :
View Solution
