The volume of the solid formed by revolving the area of the circle about y axis is :
.
The correct Answer is:D
Topper's Solved these Questions
- APPLICATIONS OF INTEGRATIONBOOK - PREMIERS PUBLISHERSCHAPTER - APPLICATIONS OF INTEGRATIONEXERCISE - Answer the following questions.
18 Videos
- APPLICATIONS OF INTEGRATIONBOOK - PREMIERS PUBLISHERSCHAPTER - APPLICATIONS OF INTEGRATIONEXERCISE - SOLUTION TO EXERCISES 9.10
20 Videos
- APPLICATIONS OF DIFFERENTIAL CALCULUSBOOK - PREMIERS PUBLISHERSCHAPTER - APPLICATIONS OF DIFFERENTIAL CALCULUSEXERCISE - Problems For Practice (Answer the following question)
39 Videos
- APPLICATIONS OF MATRICES AND DETERMINANTS BOOK - PREMIERS PUBLISHERSCHAPTER - APPLICATIONS OF MATRICES AND DETERMINANTS EXERCISE - PROBLEMS FOR PRACTICE (II. Answer the following)
15 Videos
Similar Questions
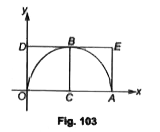
Given: the cycloid x=a(t−sint),y=a(1−cost),0≤t≤2π
Compute. (a) the areas of the surface formed by revolving the arc OBA about the x and y-axis,
(b) the volumes of the solids generated by revolving the figure OBAO about the y-axis and the axis BC,
(c ) the area of the surface generated by revolving the are BA about the axis BC,
(d) the volume of the solid generated by revolving the figure ODBEABO about the tangent line DE touching the figure at the vertex B,
(e ) the area of the surface formed by revolving the are of the cycloid (see item (d)]
View SolutionCompute the volume of the solid generated by revolving about the x-axis the area bounded by the axes of coordinates and the parabola x12+y12=a12.
View Solution
Knowledge Check
The volume of the solid formed by rotating the area between the curve y=x2 and the line y = 1 about y = 1 is (in cubic unit) :
A9π5B4π5C8π5D7π5The volume of the solid formed by rotating the area between the curve y=x2 and the line y = 1 about y = 1 is (in cubic unit) :
A9π5B4π5C8π5D7π5The volume of the solid generated by the revolution of the curve y=a3a2+x2 about x-axis is :
A12π3a2Bπ3a2C12π2a3Dπ2a3
Find the volume of the solid generated by revolving about the x-axis the figure enclosed by the astroid : x=acos3t,y=asin3t.
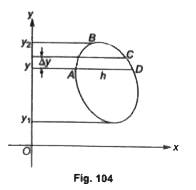
View SolutionProve that if the figure S is bounded by a simple convex contour and is situated between the ordinates y1andy2, then the volume of the solid generated by revolving this figure about the x-axis can be expressed by the formula
V=2π∫y2y1yhdy,
where h=x2(y)−x1(y),x=x1(y) being the equation of the left portion of the contour and x=x2(y) that of the right portion.
View SolutionThe volume of the solid generated by the revolution of the curve y=a3a2+x2 about x-axis is :
View SolutionThe volume of the solid formed by rotating the area enclosed between the curve y2=4x,x=4 and x = 5 about X-axis is (in cubic units)
View SolutionThe volume of the solid obtained by revolving about y-axis the area enclosed between the ellipse x2+9y2=9 and the strangth line x+3y=3, in the first quadrant is
View Solution
