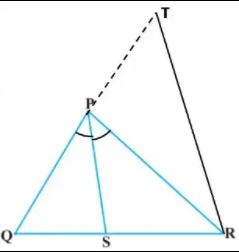
In figure PS is the bisector of ∠QPRof ΔPQR. Prove that QSSR=PQPR.

Video Solution
Text Solution
In △QRT
RT∣∣SP [By construction]
And PS intersects QT and QR at two distinct points P and Q.
Therefore,
QT and QR will be divided in the same ratio
QSSR=PTPQ......(1)
[Basic proportionality theorem: If a line is drawn parallel to one side of a triangle, intersecting the other two sides at distinct points, then the other two sides are divided in the same ratio.]
Now,
RT∣∣SP
and PR is the transversal
Therefore,
∠SPR=∠PRT....(2)
[Alternate interior angles]
and
∠QPS=∠PTR....(3)
[Corresponding angles]
Also, given the
PS is the bisector of ∠QPR∠QPR=∠SPR
From (2) and (3)
∠PTR=∠PRT
Therefore, PT=PR [Sides opposite to equal angles of a triangle are equal]
Putting PT=PR in (1)
QSSR=PQPT
Hence proved.
|
Topper's Solved these Questions
- TRIANGLESBOOK - NCERTCHAPTER - TRIANGLESEXERCISE - EXERCISE 6.3
16 Videos
- TRIANGLESBOOK - NCERTCHAPTER - TRIANGLESEXERCISE - SOLVED EXAMPLES
14 Videos
- TRIANGLESBOOK - NCERTCHAPTER - TRIANGLESEXERCISE - Exercise 6.2
10 Videos
- SURFACE AREAS AND VOLUMESBOOK - NCERTCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - EXERCISE 13.2
8 Videos
Similar Questions
Knowledge Check
If P is the husband of Q and R is the mother of S and Q, what is R to P?
A(a) MotherB(b) SisterC(c) AuntD(d) Mother-in-law
If P + Q = R and P - Q = S, prove that R2+S2=2(P2+Q2)
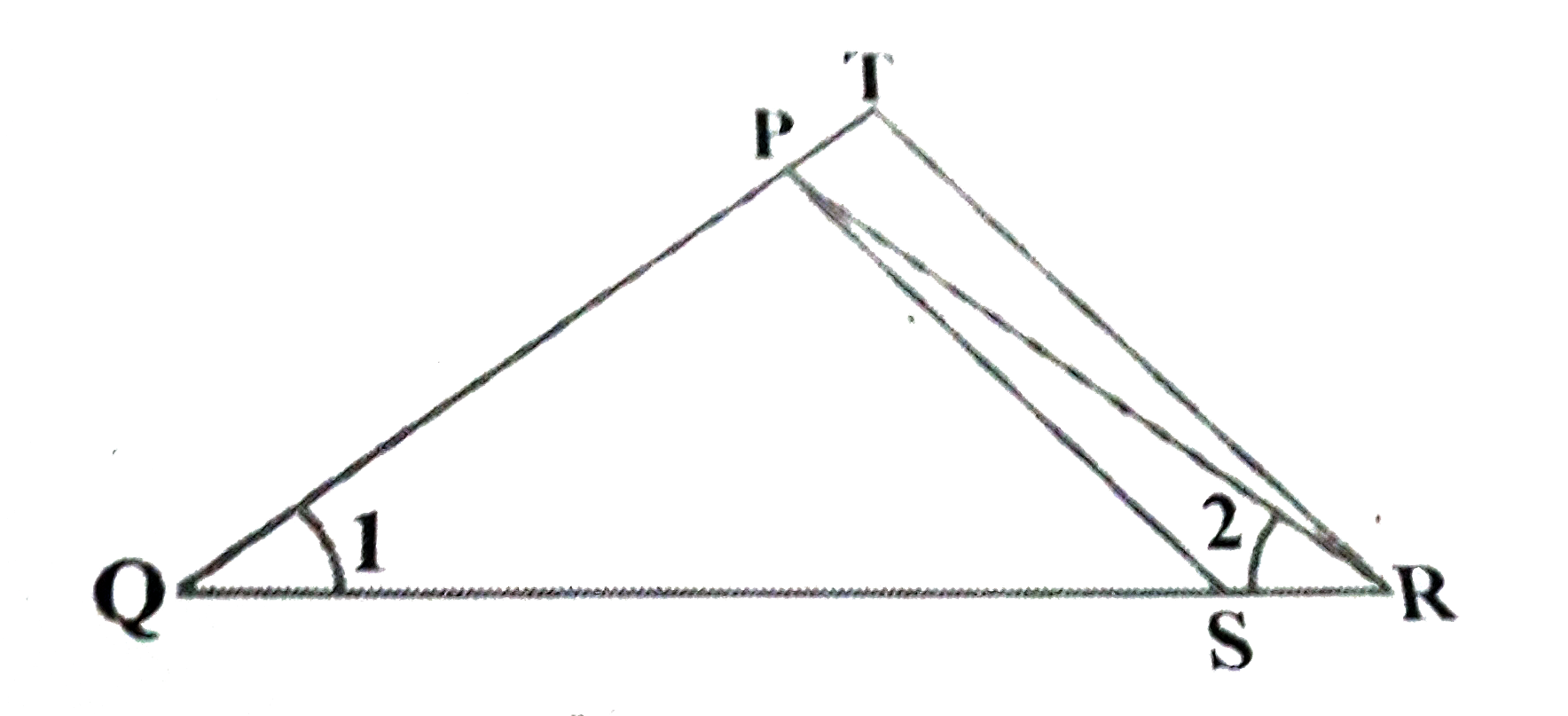
View SolutionIn figure QRQS=QTPRand ∠1=∠2. Show that ΔPQS~ΔTQR.
View SolutionIn Fig. 6.15, ∠PQR=∠PRQ, then prove that ∠PQS=∠PRT.

View SolutionIn Fig. 4.90, if PSQR , prove that POS~ROQ . (FIGURE)
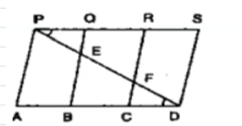
View SolutionIn Figure, PSDA is a parallelogram in which PQ=QR=RS and APBQCR. Prove that ar ( PQE)= ar ( CFD).
View SolutionIn Figure, PQR is a triangle and S is any point in its interior, show that SQ+SR<PQ+PR Given : S is any point in the interior of PQR To Prove : SQ+SR<PQ+PR Construction: Produce QS to meet PR in T
View SolutionIn Figure, ∠QPR=∠PQR and M and N are respectively on sides QR and PR of PQR such that QM=PN . Prove that OP=OQ, where O is the point of intersection of PM and QN.

View Solution


