Two identical rods each of mass m and length L, are tigidly joined and then suspended in a vertical plane so as to oscillate freely about an axis normal to the plane of paper passing through 'S' (point of supension). Find the time period of such small oscillations 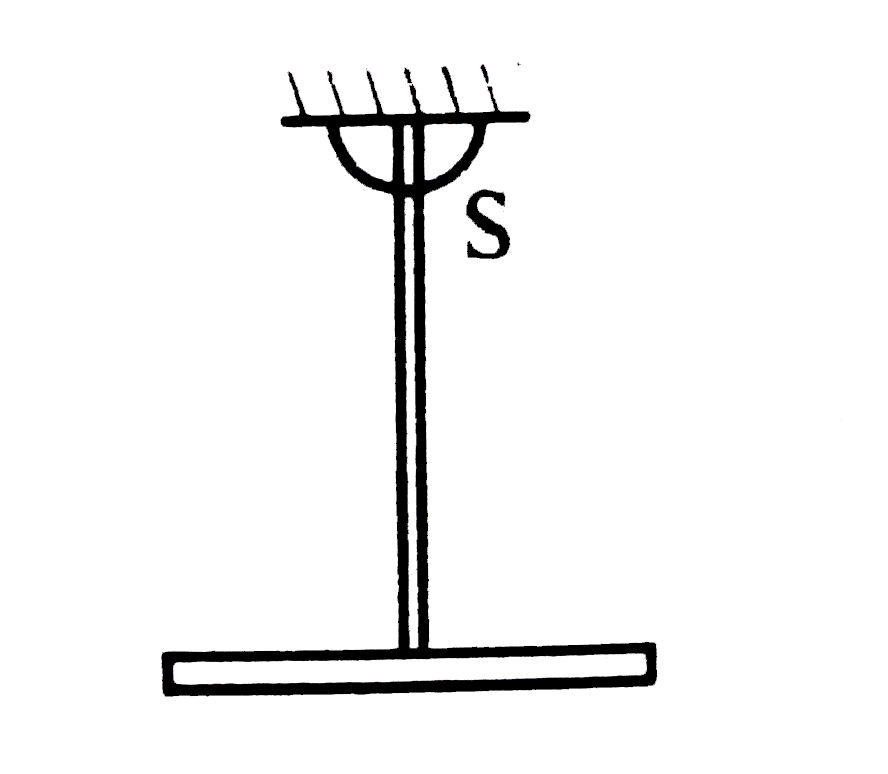
The correct Answer is:A, B
T=2π√Imgl where
I=mL33+(mL212+mL2)
=17mL212 & l=3L4
(Distance of centre of mass form hinge)
⇒T=2π√17mL212(2mg(3L/4))=2π√17L18g
Topper's Solved these Questions
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-05 [A]39videos
SIMPLE HARMONIC MOTION
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise-05 [B]12videos
SIMPLE HARMONIC MOTION
- BOOK - ALLENCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Comprehension Base Questions (5)3videos
SIMPLE HARMONIC MOTION
- BOOK - ALLENCHAPTER - RACEEXERCISE - Basic Maths (Wave Motion & Dopplers Effect) (Stationary waves & doppler effect, beats)25videos
RACE
- BOOK - ALLENCHAPTER - TEST PAPEREXERCISE - PHYSICS4videos
TEST PAPER
Similar Questions
Two identical rods each of mass M and length L are kept according to figure. Find the moment of inertia of rods about an axis passing through O and perpendicular to the plane of rods.
 View Solution
View SolutionA uniform rod of mass 'm' and length 'l' is hinged at one end 'A'. It can rotate freely about a horizontal axis passing through 'A'. If it is given a sloght angular displacement and left to itself then it oscillate. Find the time period of small oscillations.
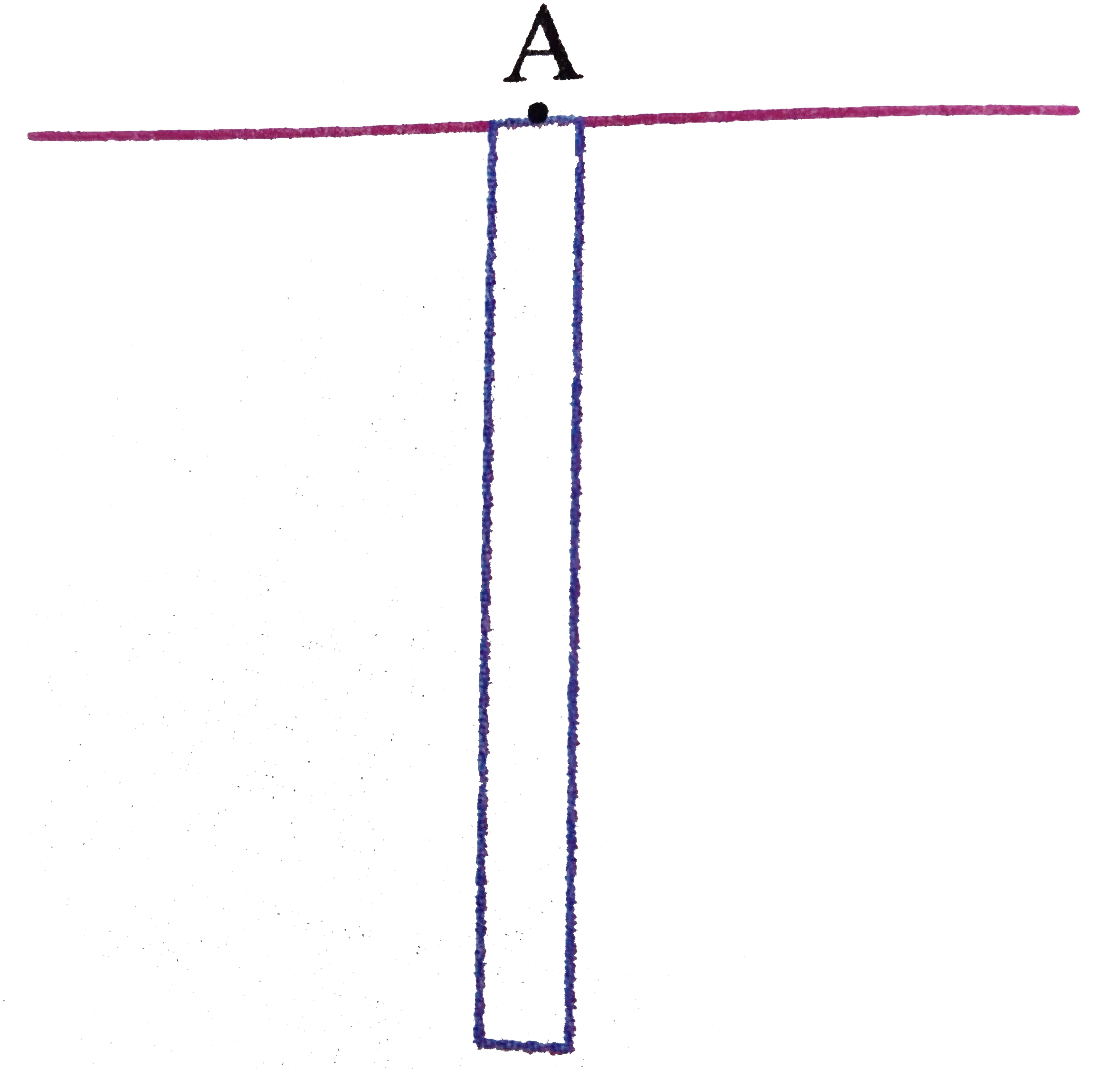 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
For identical rods each of mass m and length l make a square frame in xy plane as shown. Calculate its momen of inertia about the x-axis.
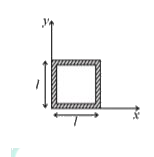 A53ml2B43ml2Cml2DNone of these
A53ml2B43ml2Cml2DNone of these - Question 2 - Select One
Two identacal rods each of length l and mass m weided toeather at right angle and edge suspended from a kinetic sides as shown Angular frequency of small oscillation of the system in its then plane about the total of suspension is
 A√3g4√2lB√3g2√2lC√3g√2lDNone of these
A√3g4√2lB√3g2√2lC√3g√2lDNone of these - Question 3 - Select One
A uniform rod of mass m and length l is suspended about its end. Time period of small angular oscillations is
 A2π√lgB2π√2lgC2π√2l3gD2π√l3g
A2π√lgB2π√2lgC2π√2l3gD2π√l3g
Consider a physical pendulum consisting of an inverted T shaped rigid body made out, of two identical, thin, uniform rods of mass M and Iength L each joined together and hinged at the top point as shown, free to rotate in a vertical plane about an axis perpendicular to the plane of the figure, the time period for 'small' angular'oscillations is T=2π√αLβg. Find |α−β|.
'(##CEN_KSR_PHY_JEE_C16_E01_034_Q18##)'View SolutionThree thin, uniform, indentical rods each or mass M and length L are joined as shown. Find the M.I. about an axis passing through O and perpendicular to the plane.
View SolutionThree identical roads each of mass M and L are joined to form an equilateral triangle. Find the M.I. about an axis perpendicular to the plane of triangle and passing through
(a) center of triangle
(b) mid-point of a rod and
(c ) one vertex of triangle.View SolutionA disc is made to oscillate about a horizontal axis passing through mid point of its radius. Determine time period.
View SolutionFour identical rods each of mass m and length L are joined to form a square. Find the M.I. about
(a) the x-axis,
(b) the y-axis,
(c) the z-axis,
(d) about 1−1,
(e) an axis passing through one corner and perpendicular to the plane.View Solution

