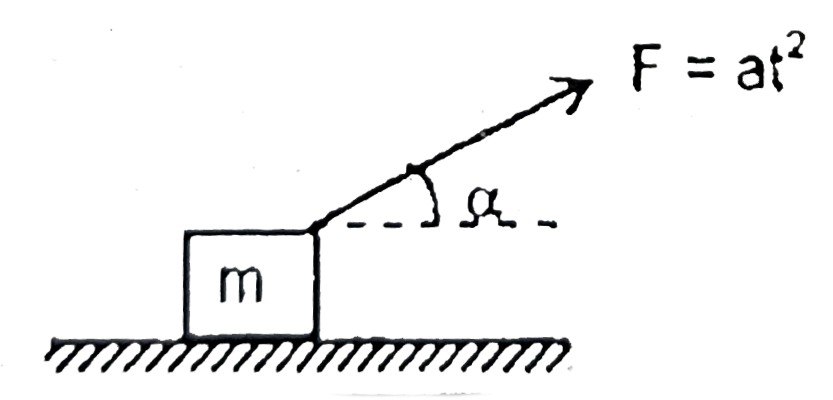
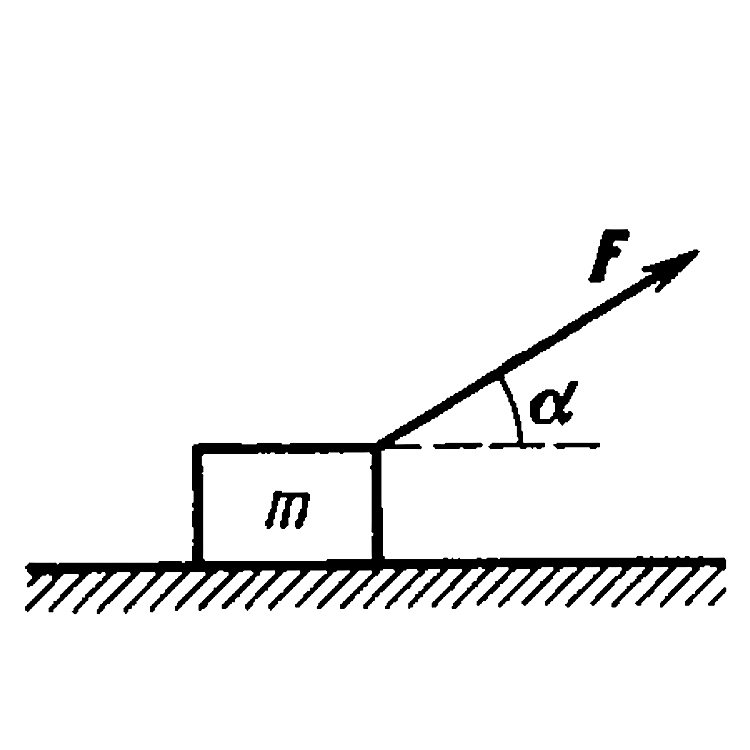
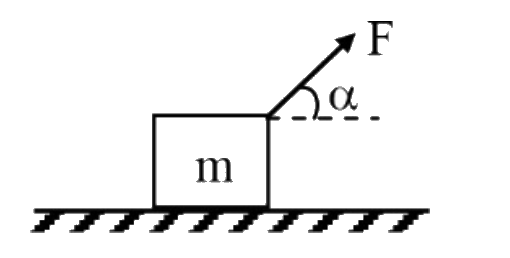
At $t = 0$ , a force $F = a t^{2}$ is applied to s small body of mass m at an angle $α$ resting on a smooth horizontal plane.

A

Velocity of the body at the moment it breaks off the plane is $\sqrt{\frac{m g^{3}}{9 a {tan}^{2} α sin α}}$

B

The distance travelled by the body before breaking off the plane is $\frac{m g^{2}}{12 a sin α tan α}$

C

Its acceleration at the time of breaking off the plane is $g cot α$ .

D

Time at which it breaks off the plane is $\sqrt{\frac{m g}{a sin α}}$

Text Solution

Verified by Experts

The correct Answer is:A, B, C, D

For breaking off the plane
$F sin α = m g$
$\Rightarrow a t_{0}^{2} sin α = m g \Rightarrow t_{0} = \sqrt{\frac{m g}{a sin α}}$
Speed at time of breaking off,
$v = t_{0} \int 0 \frac{a t^{2} cos α}{m} d t = \frac{a t_{0}^{2} cos α}{3 m}$
$= \frac{a cos α}{3 m} . \frac{m g}{a sin α} \sqrt{\frac{m g}{a sin α}}$
$\sqrt{\frac{m g^{3}}{9 a {tan}^{2} α sin α}}$
$= \frac{F cos α}{m} = \frac{a t_{0}^{2} cos α}{m} = \frac{a m g}{a sin α} . \frac{cos α}{m} = g$
$cot α$
$s = \int v d t = t_{0} \int 0 \frac{a t^{3}}{3 m} cos α d t$
$= \frac{a}{12 m} t_{0}^{2} = \frac{a}{12 m} \frac{m^{2} g^{2} cos α}{a^{2} {sin}^{2} α}$
$= \frac{m g^{2}}{12 a tan α sin α}$

|

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
At the moment t=0 force F=kt is applied to a small body of mass m resting on a smooth horizontal plane (k is a constant) The direction of this force always forms an angle $θ$ with the horizontal as shown.

Find the time t at which moment, the body breaks off the plane:
A $\frac{m g}{2 k sin θ}$
B $\frac{m g}{k sin θ}$
C $\frac{m g}{k {sin}^{2} θ}$
D $\frac{2 m g}{k sin θ}$
Question 2 - Select One
A horizontal force F is applied to a small object P of mass m on a smooth plane inclined to the horizontal at an angle $θ$ . If F is just enough to keep P in equilibrium. The magnitude of F is
A $m g {cos}^{2} θ$
B $m g {sin}^{2} θ$
C $m g cos θ$
D $m g tan θ$

BANSAL-TEST PAPERS-PHYSICS PART A

Home
Ask Doubt
Profile