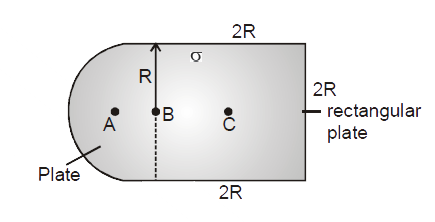
Find out the position vector of centre of mass of the trapezium made out of uniform thin rods of same material.
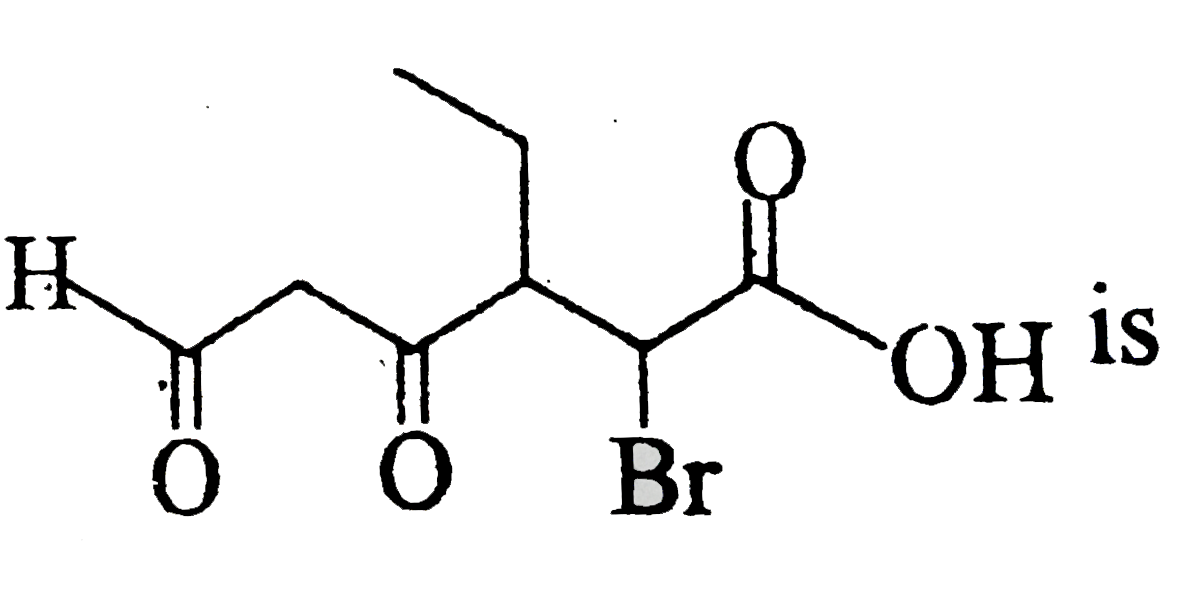
A
(√2+11)ˆi−(√2+3)ˆj√2+4
B
(√2+3)ˆi+(√2+11)ˆj√2+4
C
(√2+11)ˆi+(√2+3)ˆj√2+4
D
2ˆi+ˆj
Text Solution
The correct Answer is:C
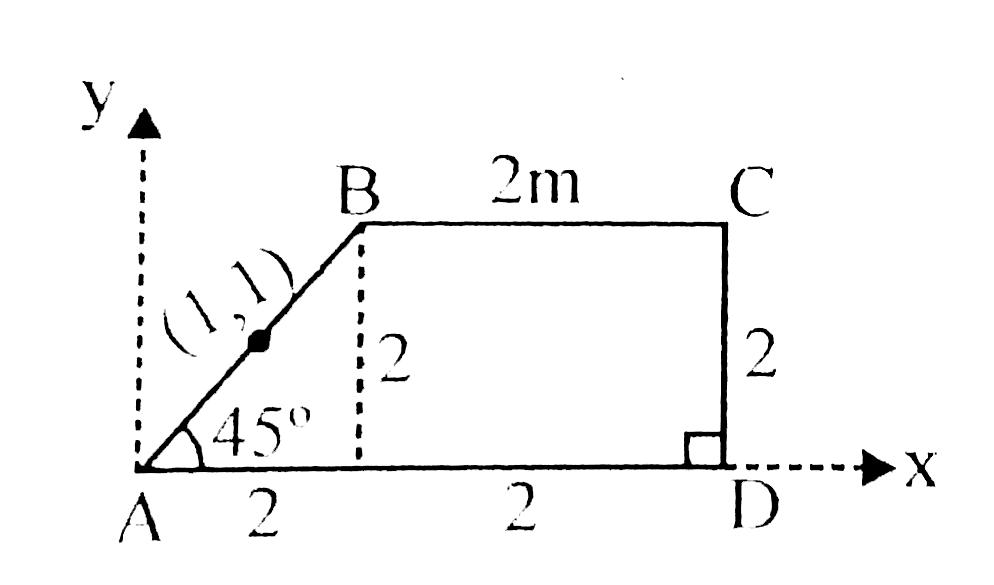
rCOM=λ(2√2)[ˆi+ˆj]+λ(2)[3ˆi+2ˆj]+λ(2)[4ˆi+ˆj]+λ(4)[2ˆi]λ(2√2)+λ(2)+λ(2)+λ(4)
=(2√2+6+8+8)ˆi+(2√2+4+2)ˆj2√2+8
=(2√22+22)ˆi+(2√2+6)ˆj2√2+8
=(√2+11)ˆi+(√2+3)ˆj√2+4
|
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICA PART B
1 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICA PART C
5 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART B
3 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
Knowledge Check
The position of the centre of mass of a cube of uniform mass density will be at
Athe centre of one faceBthe centre of the interaction of diagonals of one face.Cthe geometric centre of the cubeDthe edge of a cubeThe position of the centre of mass of a cube of uniform mass density will be at
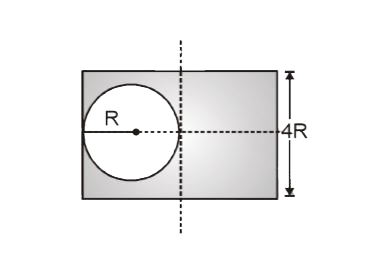
Athe centre of one faceBthe centre of the interaction of diagonals of one face.Cthe geometric centre of the cubeDthe edge of a cubeFind the position of centre of mass of a uniform disc of radius R from which a hole of radius is cut out. The centre of the hole is at a distance R/2 from the centre of the disc.
 ARr22(R2−r2) towards right of OBRr22(R2−r2) towards left of OC2Rr2(R2+r2) towards right of OD2Rr2(R2+r2) towards left of O
ARr22(R2−r2) towards right of OBRr22(R2−r2) towards left of OC2Rr2(R2+r2) towards right of OD2Rr2(R2+r2) towards left of O
Find out the position of centre of mass of the uniform lamina as shown in figure.
View SolutionFind the position of centre of mass of the uniform lamina shown in figure.

View SolutionFind the position of centre of mass of the uniform lamina shown in figure.

View SolutionFind out the position of centre of mass of the uniform lamina as shown in figure.from center of cavity

View SolutionTwo identical uniform rods AB and CD, each of length L are jointed to form a T-shaped frame as shown in figure. Locate the centre of mass of the frame. The centre of mass of a uniform rod is at the middle point of the rod.
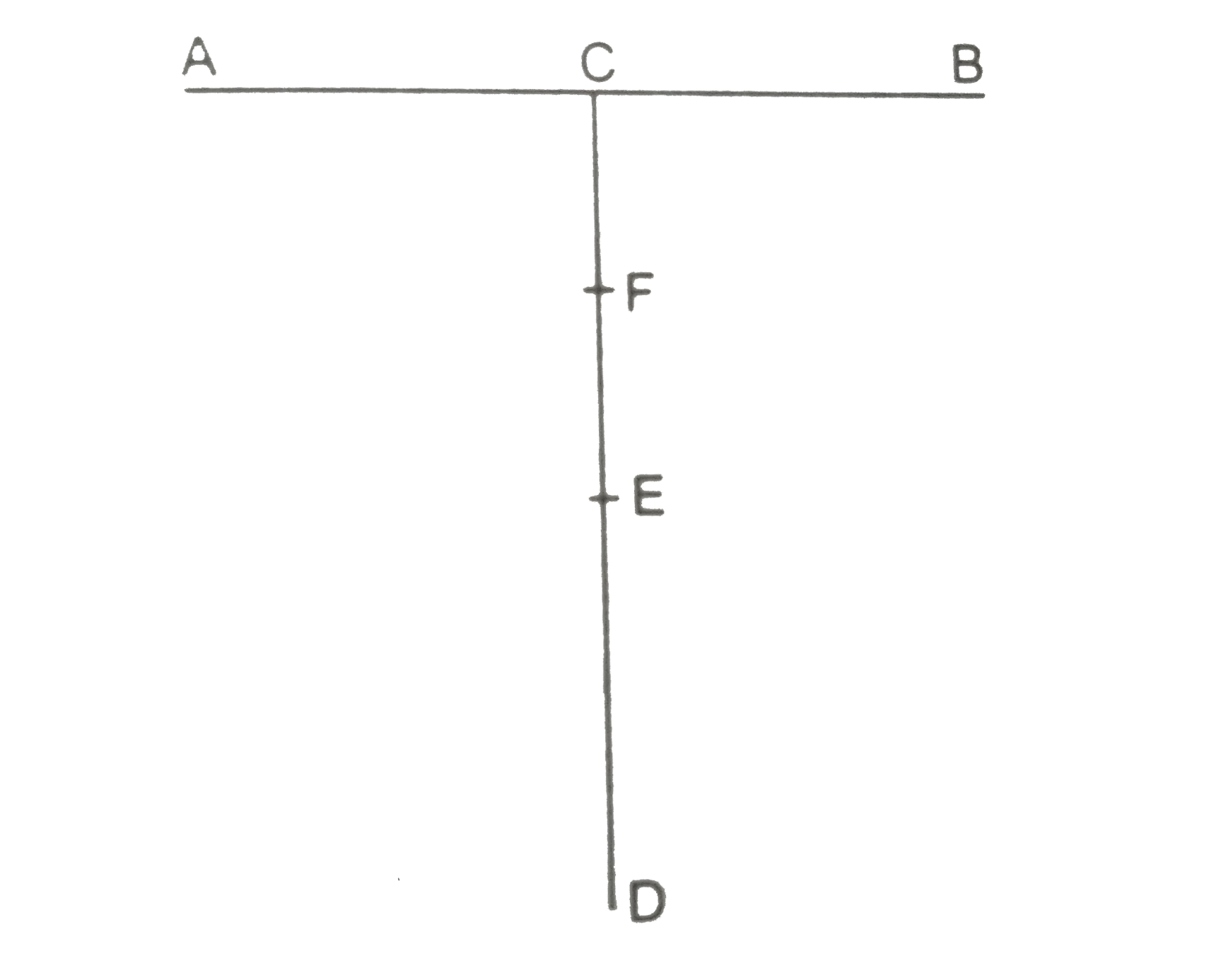
View Solution