An asteroids as moving towards a planet of mass M and radius R,from a large distance with intial speed v0 and impact parameter d as shown in the figure. The minimum value of v0 such that the asteriod does not his the planet is

√2GMRd2−R2
√4GMrd2−R2
√2GMRd2+R2
√4GMRd2+Rr2
The correct Answer is:A
Using conservation of angular momentum
mvR=mv0d
Energy conservation requires
12mv20=12mv2−GMmR
v0=√2GMRd2−R2
Topper's Solved these Questions
Similar Questions

A small asteroid is approaching a planet of mass M and radius R from a large distance. Initially its velocity (u) is along a tangent to the surface of the planet. It fall on the surface making an angle of 302 with the vertical. Calculate u.
View Solution_S01_010_Q01.png)
A particle of mass m0 is projected from the surface of body (1) with initial velocity v0 towards the body (2). As shown in figure find the minimum value of v0 so that the particle reaches the surface of body (2). Radius of body (1) is R1 and mass of body (1) is M1. Radius body (2) is R2 and mass of the body (2) is M2 distance between te center the center of two bodies is D.
View Solution
Knowledge Check
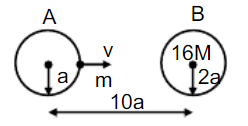
Two planets of mass M and 16M of radius a and 2a respectively, are at distance 10a. Find minimum speed of a particle of mass m at surface of smaller planet so that it can reached from smaller planet to larger planet.A√(59)GMaB√(49)GMaC√(39)GMaD√(29)GMa
_S01_010_Q01.png)
A particle of mass m0 is projected from the surface of body (1) with initial velocity v0 towards the body (2). As shown in figure find the minimum value of v0 so that the particle reaches the surface of body (2). Radius of body (1) is R1 and mass of body (1) is M1. Radius body (2) is R2 and mass of the body (2) is M2 distance between the center of two bodies is d.
View Solution
Two planets of mass M and 16M of radius a and 2a respectively, are at distance 10a. Find minimum speed of a particle of mass m at surface of smaller planet so that it can reached from smaller planet to larger planet.
View SolutionThe earth is a homogeneous sphere of mass M and radius R. There is another spherical planet of mass M and radius R whose density changes with distance r from the centre as p=p0r. (a) Find the ratio of acceleration due to gravity on the surface of the earth and that on the surface of the planet. (b) Find p0.
View SolutionA body is moving in a low circular orbit about a planet of mass M and radius R. The radius of the orbit can be taken to be R itself. Then the ratio of the speed of this body in the orbit to the escape velocity from the planet is :
View SolutionA body is moving in a low circular orbit about a planet of mass M and radius R. The radius of the orbit can be taken to be R itself. Then the ratio of the speed of this body in the orbit to the escape velocity from the planet is :
View SolutionA particle of charge q and mass m is moving with a velocity −vˆi(v≠0) towards a large screen placed int eh Y-Z plane at a distance d. If there is a magnetic field →B=B0ˆk , the minimum value of v for which the particle will not hit the screen is :
View SolutionA particle of charge q and mass m is moving with a velocity −vˆi(v≠0) towards a large screen placed in the Y-Z plane at a distance d. If there is a magnetic field →B=B0ˆk , the minimum value of v for which the particle will not hit the screen is :
View Solution
