A particle of charge q and mass m is moving with a velocity −vˆi(v≠0) towards a large screen placed in the Y-Z plane at a distance d. If there is a magnetic field →B=B0ˆk , the minimum value of v for which the particle will not hit the screen is :
qdB03m
2qdB0m
qdB0m
qdB02m
To solve the problem, we need to determine the minimum velocity v of a charged particle so that it does not hit a screen placed in the Y-Z plane at a distance d. The particle has a charge q, mass m, and is moving with a velocity −v^i in the presence of a magnetic field →B=B0^k.
1. Identify the Forces Acting on the Particle:
The particle experiences a magnetic force due to its motion in the magnetic field. The magnetic force →FB is given by:
→FB=q(→v×→B)
2. Calculate the Magnetic Force:
Given that →v=−v^i and →B=B0^k, we can calculate the cross product:
→v×→B=(−v^i)×(B0^k)=−vB0(^i×^k)=−vB0(−^j)=vB0^j
Therefore, the magnetic force becomes:
→FB=qvB0^j
3. Determine the Radius of the Circular Path:
The magnetic force acts as the centripetal force required to keep the particle in circular motion. The centripetal force Fc is given by:
Fc=mv2R
Setting the magnetic force equal to the centripetal force:
qvB0=mv2R
4. Solve for the Radius R:
Rearranging the equation to find the radius R:
R=mvqB0
5. Condition for Not Hitting the Screen:
For the particle to not hit the screen, the radius of the circular path must be less than or equal to the distance d from the particle to the screen:
R≤d
Substituting for R:
mvqB0≤d
6. Solve for the Minimum Velocity v:
Rearranging the inequality gives:
v≤qB0dm
Thus, the minimum value of v for which the particle will not hit the screen is:
v=qB0dm
Final Answer:
The minimum value of v for which the particle will not hit the screen is:
qB0dm
The particle experiences a magnetic force due to its motion in the magnetic field. The magnetic force →FB is given by:
→FB=q(→v×→B)
Given that →v=−v^i and →B=B0^k, we can calculate the cross product:
→v×→B=(−v^i)×(B0^k)=−vB0(^i×^k)=−vB0(−^j)=vB0^j
Therefore, the magnetic force becomes:
→FB=qvB0^j
The magnetic force acts as the centripetal force required to keep the particle in circular motion. The centripetal force Fc is given by:
Fc=mv2R
Setting the magnetic force equal to the centripetal force:
qvB0=mv2R
Rearranging the equation to find the radius R:
R=mvqB0
For the particle to not hit the screen, the radius of the circular path must be less than or equal to the distance d from the particle to the screen:
R≤d
Substituting for R:
mvqB0≤d
Rearranging the inequality gives:
v≤qB0dm
Thus, the minimum value of v for which the particle will not hit the screen is:
v=qB0dm
The minimum value of v for which the particle will not hit the screen is:
qB0dm
Topper's Solved these Questions
Similar Questions
A charge particle of charge q and mass m is moving with velocity v as shown in fig In a uniform magnetic field B along -ve z-direction.Select the correct alternative (s).

View SolutionA particle of mass m and charge Q moving with a velocity v enters a region on uniform field of induction B Then its path in the region is s
View Solution
Knowledge Check
A particle of mass m and charge q has and initial velocity →v=υ0ˆj. If an electric field →E=→–––(0)ˆi and magnetic field →B=B0ˆi act on the particle, its speed will double after a time :
At=2mv0qE0Bt=2B0qmv0Ct=√3B0qmv0Dt=√3mv0qE0
A particle of charge q and mass m moving with a velocity v along the x-axis enters the region x>0 with uniform magnetic field B along the ˆk direction. The particle will penetrate in this region in the x-direction upto a distance d equal to
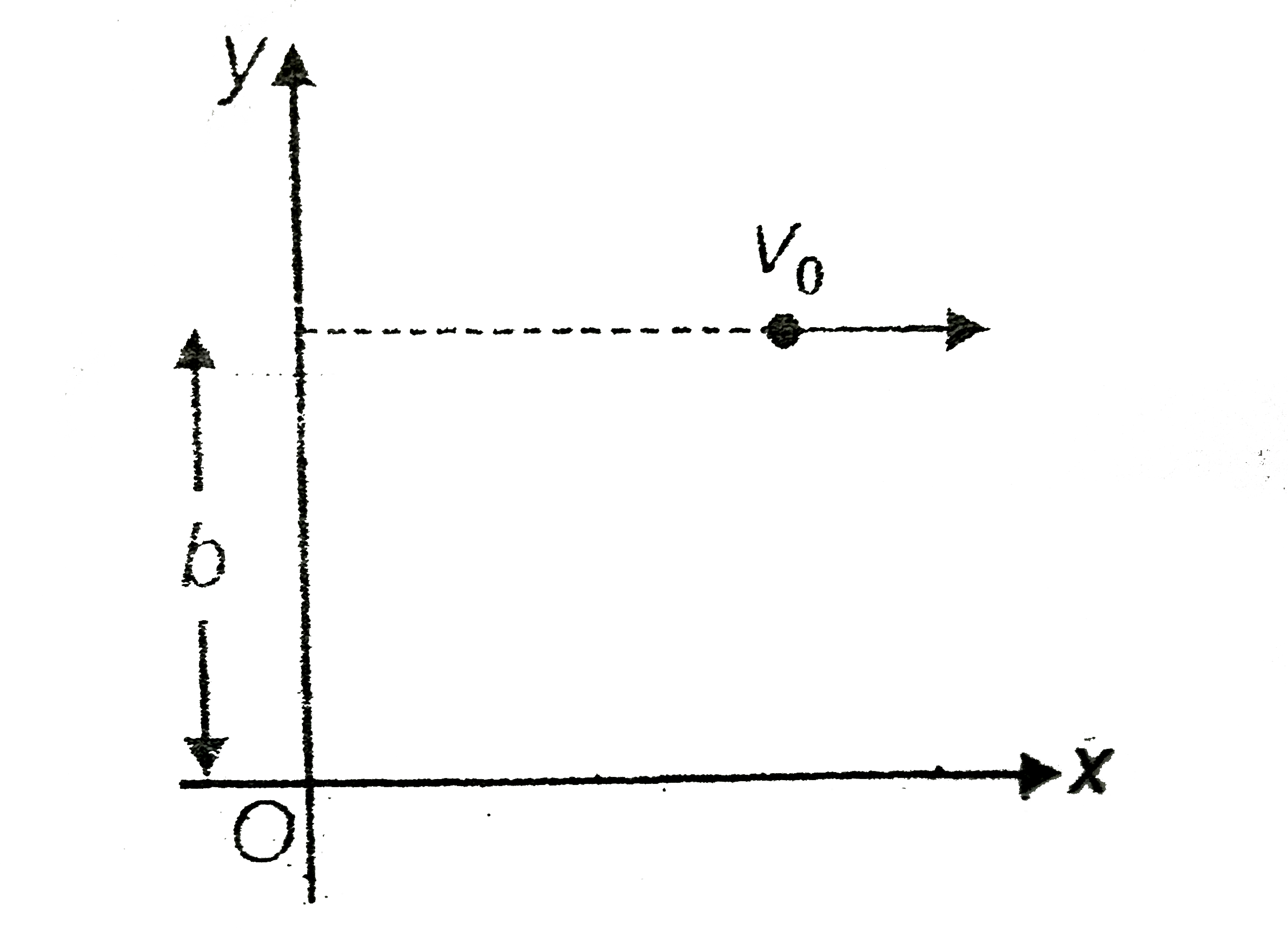
View SolutionA particle of mass m is moving with constant velocity v0 along the line y=b. At time t=0 it was at the point (0,b). At time t=bv0, the
View SolutionA particle of charge q and mass m released from origin with velocity →v=v0ˆi into a region of uniform electric and magnetic fields parallel to y-axis. i.e., →E=E0ˆjand→B=B0ˆj. Find out the position of the particle as a functions of time
Strategy : Here →E∣∣→B
The electric field accelerates the particle in y-direction i.e., component of velocity goes on increasing with acceleration
ay=Fym=Fem=qE0m
The magnetic field rotates the particle in a circle in x-z plane (perpendicular to magnetic field) The resultant path of the particle is a helix with increasing pitch.
Velocity of the particle at time t would be
→v(t)=vxˆi+vyˆj+vzˆk
View SolutionConsider the situation described in the previous example. A particle of mass m having a charge q is placed at a distance d from the metal sheet and is projected towards it. Find the maximum velocity of projection for which the particle does not hit the sheet.
View SolutionA particle of charge q and mass m is projected from the origin with velocity v=v0ˆi in a non uniformj magnetic fiedl B=−B0xˆk. Here v0 and B0 are positive constants of proper dimensions. Find the maximum positive x coordinate of the particle during its motion.
View SolutionA particle of charge q and mass m starts moving from the origin under the action of an electric field →E=E0ˆiand→B=B0ˆi with a velocity →v=v0ˆj. The speed of the particle will become 2v0 after a time.
View SolutionA particle of mass m and charge q has and initial velocity →v=υ0ˆj. If an electric field →E=→–––(0)ˆi and magnetic field →B=B0ˆi act on the particle, its speed will double after a time :
View Solution
