The linear mass density of a thin rod AB of length L varies from A to B as λ(x)=λ0(1+xL). Where x is the distance from A. If M is the mass of the rod then its moment of inertia about an axis passing through A and perpendicualr to the rod is :
512ML2
718ML2
25ML2
37ML2
To find the moment of inertia of a thin rod AB of length L with a varying linear mass density given by λ(x)=λ0(1+xL), we will follow these steps:
Step 1: Understand the mass density function
The linear mass density λ(x) varies along the length of the rod. At point A (where x=0), the density is λ0 and at point B (where x=L), the density is λ0(1+1)=2λ0.
Step 2: Define an infinitesimal element
Consider an infinitesimal element of the rod at a distance x from point A with a length dx. The mass dm of this element can be expressed as:
dm=λ(x)dx=λ0(1+xL)dx
Step 3: Write the expression for moment of inertia
The moment of inertia dI of this infinitesimal mass about point A is given by:
dI=x2dm=x2⋅λ0(1+xL)dx
Step 4: Integrate to find total moment of inertia
To find the total moment of inertia I of the rod about point A, integrate dI from x=0 to x=L:
I=∫L0dI=∫L0x2⋅λ0(1+xL)dx
Step 5: Expand the integrand
Expanding the integrand:
I=λ0∫L0(x2+x3L)dx
Step 6: Compute the integrals
Now, compute the integrals separately:
1. ∫L0x2dx=(x33)L0=L33
2. ∫L0x3dx=(x44)L0=L44
Substituting these results back into the expression for I:
I=λ0(L33+L44L)=λ0(L33+L34)
Step 7: Combine the fractions
Finding a common denominator (which is 12):
I=λ0L3(412+312)=λ0L3⋅712
Step 8: Relate λ0 to total mass M
To express I in terms of M, we need to find the total mass M of the rod:
M=∫L0dm=∫L0λ0(1+xL)dx
Calculating this integral:
M=λ0(∫L01dx+∫L0xLdx)=λ0(L+L22L)=λ0(L+L2)=3λ0L2
Step 9: Solve for λ0
From the equation for M:
λ0=2M3L
Step 10: Substitute λ0 back into I
Substituting λ0 into the moment of inertia:
I=2M3L⋅L3⋅712=7ML218
Final Answer
Thus, the moment of inertia of the rod about an axis passing through A and perpendicular to the rod is:
I=718ML2
The linear mass density λ(x) varies along the length of the rod. At point A (where x=0), the density is λ0 and at point B (where x=L), the density is λ0(1+1)=2λ0.
Consider an infinitesimal element of the rod at a distance x from point A with a length dx. The mass dm of this element can be expressed as:
dm=λ(x)dx=λ0(1+xL)dx
The moment of inertia dI of this infinitesimal mass about point A is given by:
dI=x2dm=x2⋅λ0(1+xL)dx
To find the total moment of inertia I of the rod about point A, integrate dI from x=0 to x=L:
I=∫L0dI=∫L0x2⋅λ0(1+xL)dx
Expanding the integrand:
I=λ0∫L0(x2+x3L)dx
Now, compute the integrals separately:
1. ∫L0x2dx=(x33)L0=L33
2. ∫L0x3dx=(x44)L0=L44
I=λ0(L33+L44L)=λ0(L33+L34)
Finding a common denominator (which is 12):
I=λ0L3(412+312)=λ0L3⋅712
To express I in terms of M, we need to find the total mass M of the rod:
M=∫L0dm=∫L0λ0(1+xL)dx
Calculating this integral:
M=λ0(∫L01dx+∫L0xLdx)=λ0(L+L22L)=λ0(L+L2)=3λ0L2
From the equation for M:
λ0=2M3L
Substituting λ0 into the moment of inertia:
I=2M3L⋅L3⋅712=7ML218
Thus, the moment of inertia of the rod about an axis passing through A and perpendicular to the rod is:
I=718ML2
Topper's Solved these Questions
Similar Questions
The linear density of a thin rod of length 1m lies as λ=(1+2x), where x is the distance from its one end. Find the distance of its center of mass from this end.
View SolutionA thin rod AB of length a has variable mass per unit length ρ0(1+xa) where x is the distance measured from A and ρ0 is a constant.
Find moment of inertia of the rod about an axis passing through A and perpendicular to AB.
View Solution
Knowledge Check
Moment of inertia of a thin rod of mass m and length l about an axis passing through a point l/4 from one end and perpendicular to the rod is
Aml212Bml23C7ml248Dml29The moment of inertia of thin rod of linear density λ and length l about an axis passing through one end and perpendicular to its length is
Aλl212Bλl23Cλl312Dλl33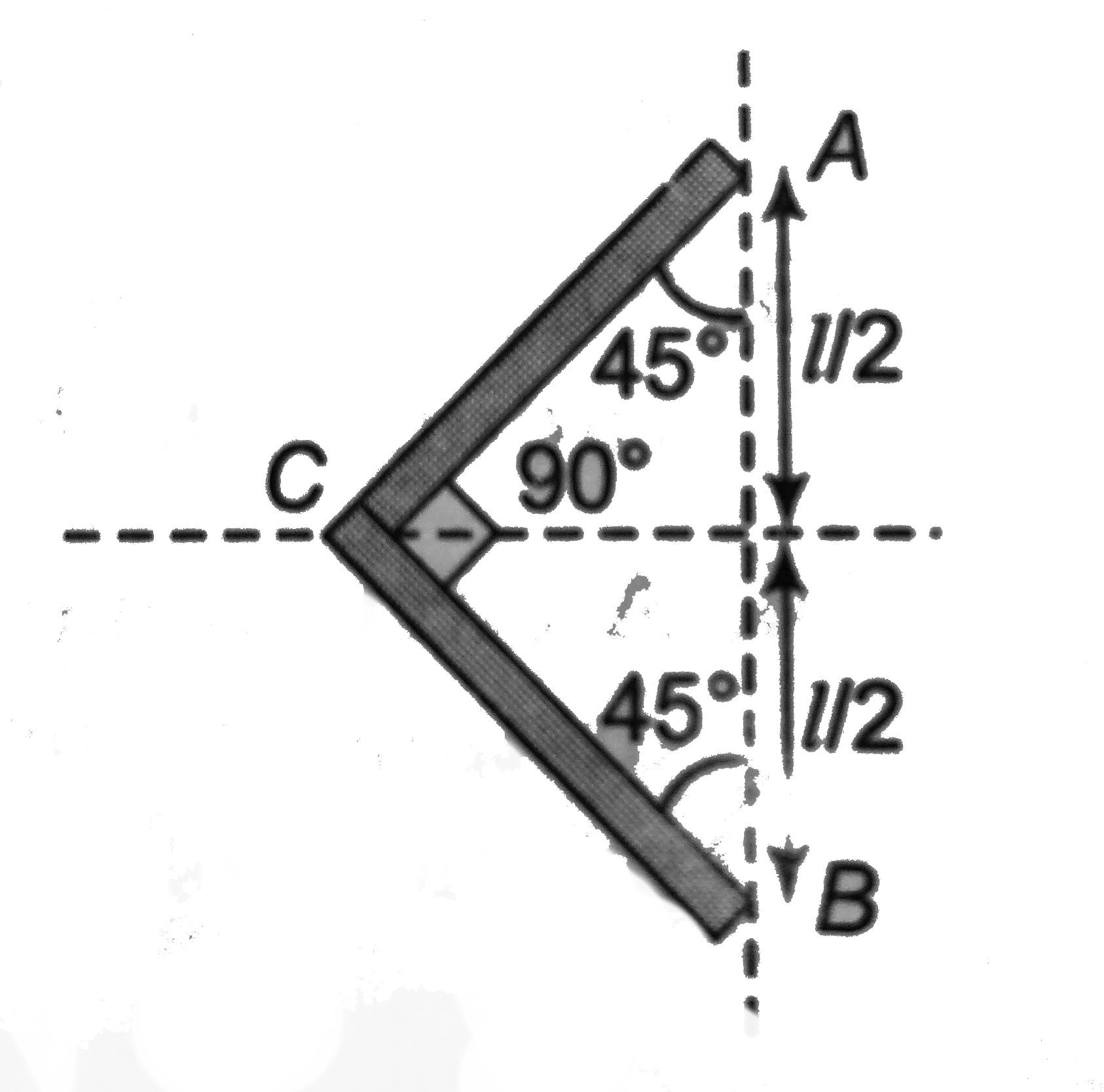
Linear mass density of the two rods system, AC and CD is x. moment of inertia of two rods about an axis passing through AB isAxl34√3Bxl3√2Cxl34Dxl36√2
The linear mass density of a rod of length 2L varies with distance (x) from center as λ=λ0(1+xL) .The distance of COM from center is nL.Then n is
View SolutionLinear mss density (mass/length) of a rod depends on the distanec from one end (say A) as λx=(αx+β) here α and β are constants, find the moment of inertia of this rod about an axis passing through A and perpendicular to the rod. Length of the rod is l.
View SolutionLinear mass density of a rod AB ( of length 10 m) varied with distance x from its end A as λ=λ0x3 (λ0 is positive constant). Distance of centre of mass the rod, form end B is
View SolutionThe linear mass density (i.e. Mass per unit length) of a rod of length L is given by ρ=ρ0xL, where ρ0 is constant and x is the distance from one end A. Find the M.I. about an axis passing through A and perpendicular to length of rod. Express your answer in terms of mass of rod M and length L.
View SolutionA rod of length 2m has uniform cross- section but linear mass density varies as λ=(1+x)kgm .It is placed as shown in figure.The moment of inertia of rod about an axis passing through O and perpendicular to rod is (x is in metre )
View Solution
