The period of small oscillations of a simple pendulum of length if its point of suspension moves with a constant acceleration with respect to earth is ( and are unit vectors in horizontal in horizontal and vertically upward directions respectively)
The correct Answer is:A
Point is moving as shown
So . Of particle
So
So time period

Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - II1videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 2, PART - III12videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Exercise- 1, PART - II37videos
SIMPLE HARMONIC MOTION
- BOOK - RESONANCECHAPTER - SEMICONDUCTORSEXERCISE - Exercise 388videos
SEMICONDUCTORS
- BOOK - RESONANCECHAPTER - TEST PAPERSEXERCISE - FST-330videos
TEST PAPERS
Similar Questions
Find the period of oscillation of a pendulum of length l if its point of suspension is
(a) moving vertically up with acceleration a
(b) moving vertically down with acceleration a(<g)
(c) failing freely under gravity
moving horizontal with acceleration a.View SolutionFind the period of small oscillations of a mathematical pendulum of length l if its point of suspension O moves relative to the Earth's surface in an arbitrary directio with a constant acceleration w (figure). Calculate that period if l=21cm,w=g/2, and the angle between the vectors w and g equals beat=120∘.
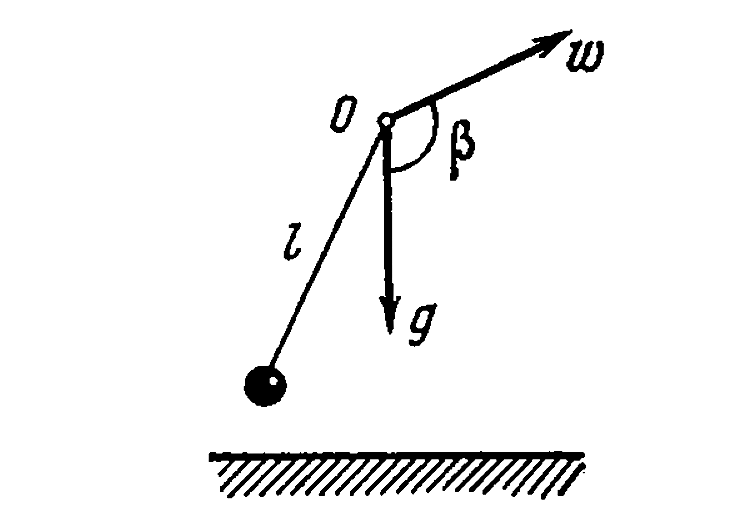 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
An aeroplane is flying in vertical plane at an angle of 30∘ with the horizontal (north) and wind is is blowing from west.A package is dropped from an aeroplane. The velocity of the wind if package hits a kite flying in the space with a position vector →R=(400√3ˆi+80ˆj+200ˆk)m with respect to the point of dropping. (Here ˆi and ˆj are the unit vectors along north and vertically up respectively and ˆk be the unit vector due east. Assume that the bag is light enough to get carried away by the wind)
A50 m/secB25 m/secC20 m/secD10 m/sec - Question 2 - Select One
A simple pendulum is suspended from the roof of a trolley which moves in a horizontal direction with an acceleration α, then the time period is given by T=2π√(IT) where g is equal to
AgBg−αCg+αD√(g2+α2) - Question 3 - Select One
A projectile is given an initial velocity of (ˆi+2ˆj) The Cartesian equation of its path is (g=10ms−1) ( Here , ˆi is the unit vector along horizontal and ˆj is unit vector vertically upwards)
Ay=2x−5x2By=x−5x2C4y=2x−5x2Dy=2x−25x2
At the given instan four particle having masses and accleration as shown in the shown in the figure lie at verties of a square. Acceleration on the center of mass of system is : (A) 15(∧i+∧j) (B) 15(∧j+∧i) (C) 15(∧i−∧j) (D) −15(∧i+∧j)
View SolutionIf →u,→v,→w are three non- coplanar unit vectors and α,β,γ the angles between →u and →v,→v and →w,→w and →u respectively and →x,→y,→z are unit vectors along the bisectors of the angles α,β,γ respectively . Prove that
[→x×→y→y×→z→z×→x]=116[→u→v→w]2 sec2.α2 sec2.β2 sec2.γ2View SolutionA simple pendulum of length l is suspended from the ceilling of a car moving with a speed v on a circular horizontal road of radius r. a. Find the tension in the string when it is at rest with respect to the car. b.Find the time period of small oscillation.
View SolutionThe position vector of a point R which divides the line joining two points P and Q whose position vectors are ˆi+2ˆj−ˆkand−ˆi+ˆj−ˆk respectively, in the ratio 2:1 externally is
View SolutionIf the vectors 5ˆi−xˆj+3ˆkand−3ˆi+2ˆj−yˆk are parallel, the value of xandy respectively are
View Solution

