A tower AB leans towards west making an angle $α$ with the vertical . The anlgular elevation of B , the topmost point of the tower is $β$ as obsreved from a point C due east of A at distance d from A.If the angular elevation of B from a pont D at a distance 2d due east of C is $γ$ , then prove that 2 tan $α$ = cot $γ$ -cot $β$

A

$2 tan α = 2 cot β - cot γ$

B

$2 tan α = 3 cot β - cot γ$

C

$tan α = cot β - cot γ$

D

None of these

Video Solution

Text Solution

Verified by Experts

Observe the following figure

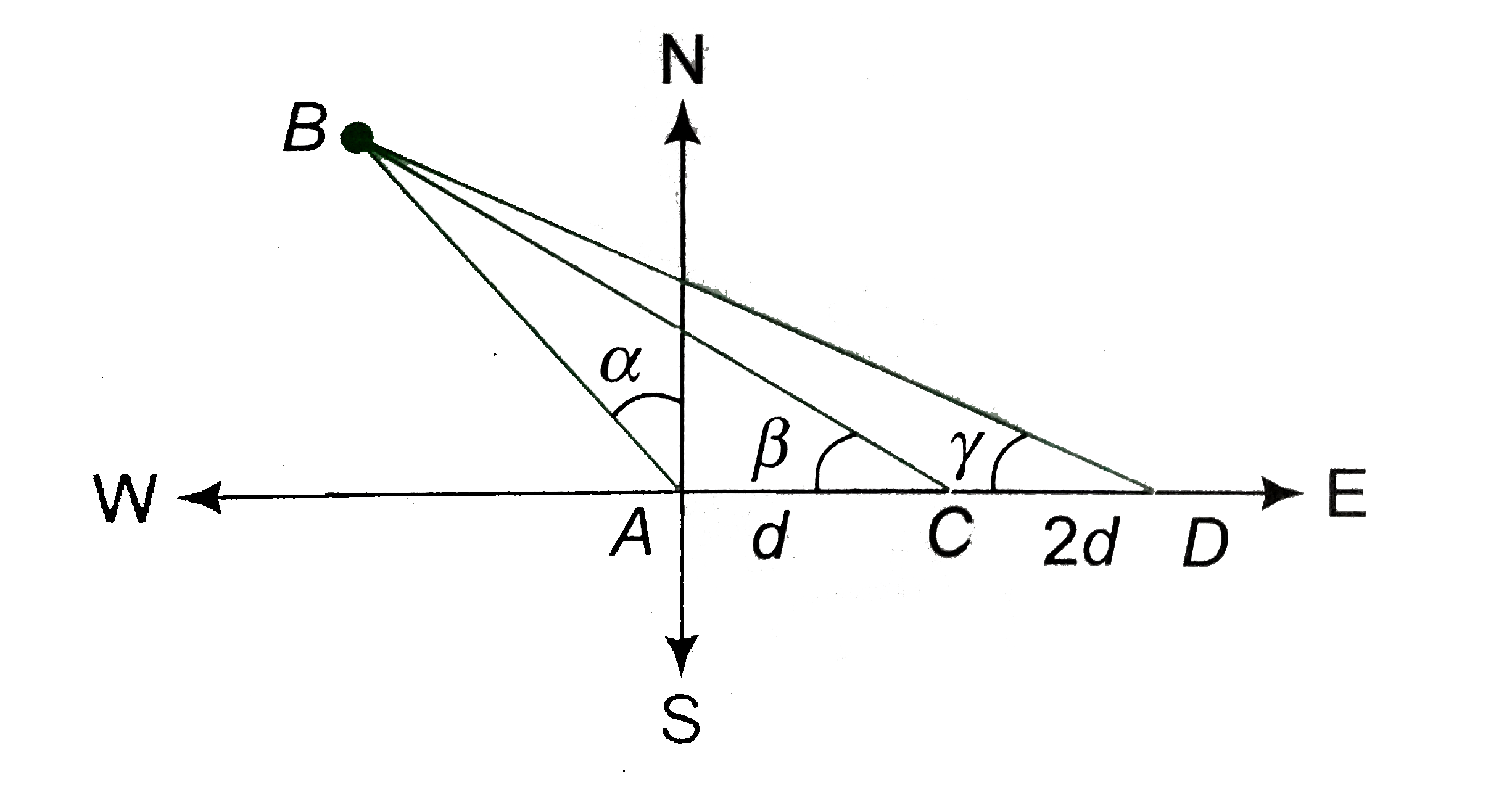
Using m-n theorem in triangle ABD we have
$(d + 2 d) cot B η = d cot α - 2 d cot (90 + α)$
$\Rightarrow 3 d cot β = cot α + 2 d tan α$
$∴ 2 tan α = 3 cot β - cot α$

|

Updated on:21/07/2023

Class 12 MATHS HIGHT AND DISTANCE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A tower leans towards west making an angle $α$ with the vertical. The angular elevation of B, the top most point of the tower, is $75^{\circ}$ as observed from a point C due east of A at a distance of 20 units. If the angular elevation of B from a point due east of C at a distance of 20 units from C is $45^{\circ}$ , then $tan α$ is equal to
A $\frac{\sqrt{3} + 1}{2}$
B $\frac{\sqrt{3} + 1}{\sqrt{3} - 1}$
C $3 - 2 \sqrt{3}$
D $\frac{\sqrt{3} - 1}{2}$
Question 2 - Select One
A tower AB leans towards west making an angle $α$ with vertical. The angular elevation of B, the top most point of the tower, is $60^{\circ}$ as observed from a point C due east of A at a elevation of 10 ft from A. If the angular elevation of B from a point D due east of C at a distance of 20 ft from C is $45^{\circ}$ , then the value of $2 tan α$ is equal to
A $\sqrt{3} + 1$
B $\frac{\sqrt{3} + 1}{\sqrt{\sqrt{3}} - 1}$
C $\sqrt{3} - 1$
D $\frac{\sqrt{3} - 1}{\sqrt{3} + 1}$
Question 3 - Select One
The angle of elevation of the top of a tower at a point A due south of the tower is $α$ and at $β$ due east of the tower is $β$ . If AB=d, then height of the tower is
A $d \sqrt{{cot}^{2} α + {cot}^{2} β}$
B $\frac{d}{\sqrt{{cot}^{2} α + {cot}^{2} β}}$
C $d cot α$
DNone of these

CENGAGE-HIGHT AND DISTANCE -Exercise

Home
Ask Doubt
Profile