A Uniformly charged solid non-conducting sphere of uniform volume charge density ρ and radius R is having a concentric spherical cavity of radius r. Find out electric field intensity at following points, as shown in the figure :

(i) Point A (ii) Point B
(iii) Point C (iv) Centre of the sphere
Method-I :
(i) For point A : We can consider the solid part of sphere to be made of large number of spherical shells which have uniformly distributed charge on its surface. Now, since point A lies inside all spherical shells so electric field intensity due to all shells will be zero.
−→EA=0
(ii) For point B : All the spherical shells for which point B lies inside will make electric field zero at point B. So electric field will be due to charge present from radius r to OB.
So, −→EB=K43π(OB3−r3)ρOB2−−→OB=ρ3ε0[OB3−r3]OB3−−→OB
(ii) For point C, similarly we can say that for all the shell points C lies outside the shell
So, −→EC=K[43π(R3−r3)][OC]3−−→OC=ρ3ε0R3−r3[OC]3−−→OC
Method-II : We can consider that the spherical cavity is filled with charge density ρ and also −ρ, thereby making net charge density zero after combining. We can consider two concentric solid spheres : One ofradius R and charge density ρ and other of radius r and charge density −ρ. applying susperposition principle :
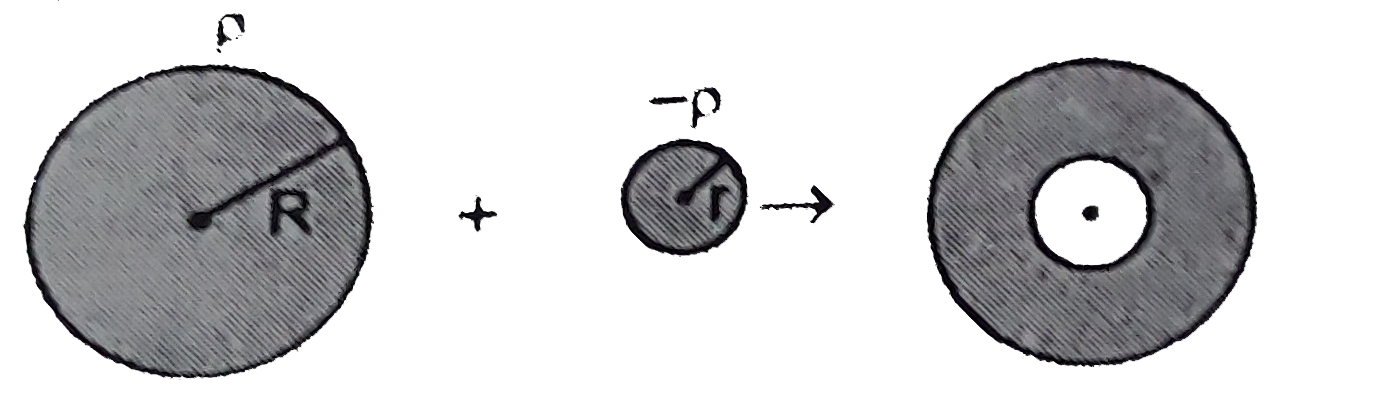
(i) −→EA=−→Eρ+−−→E−ρ=ρ(−−→OA)3ε0+[−ρ(−−→OA)]3ε0=0
(ii) −→EB=−→Eρ+−−→E−ρ=ρ(−−→OB)3ε0+K[43πr3(−ρ)](OB)3−−→OB
=[ρ3ε0−r3ρ3ε0(OB)3]−−→OB=ρ3ε0[1−r3OB3]−−→OB
(iii) −→EC=−→Eρ+−−→E−ρ=K(43πR3ρ)OC3−−→OC+K(43πr3(−ρ))OC3−−→OC=ρ3e0(OC)3[R3−r3]−−→OC
(iv) −→EO=−→Eρ+−−→E−ρ=0+0=0
Topper's Solved these Questions
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Problems
22 Videos
- ELECTROSTATICSBOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (A)
9 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P
19 Videos
- EXPERIMENTAL PHYSICSBOOK - RESONANCECHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II
10 Videos
Similar Questions
A solid sphere having uniform charge density ρ and radius R is shown in figure. A spherical cavity ofradius R2 is made in it. What is the potential at point O?

View SolutionA solid non conducting sphere of radius R and uniform volume density ρ has centre at origin. Find out electric field intensity in vector form at following positions :
(i) (R, 0, 0) (ii) (0, 0, R/2) (iii) (R, R, R)
View Solution
Knowledge Check
A nonconducting sphere of radius R is filled with uniform volume charge density −ρ. The center of this sphere is displaced from the origin by →d. The electric field →E at any point P having position vector inside the sphere is
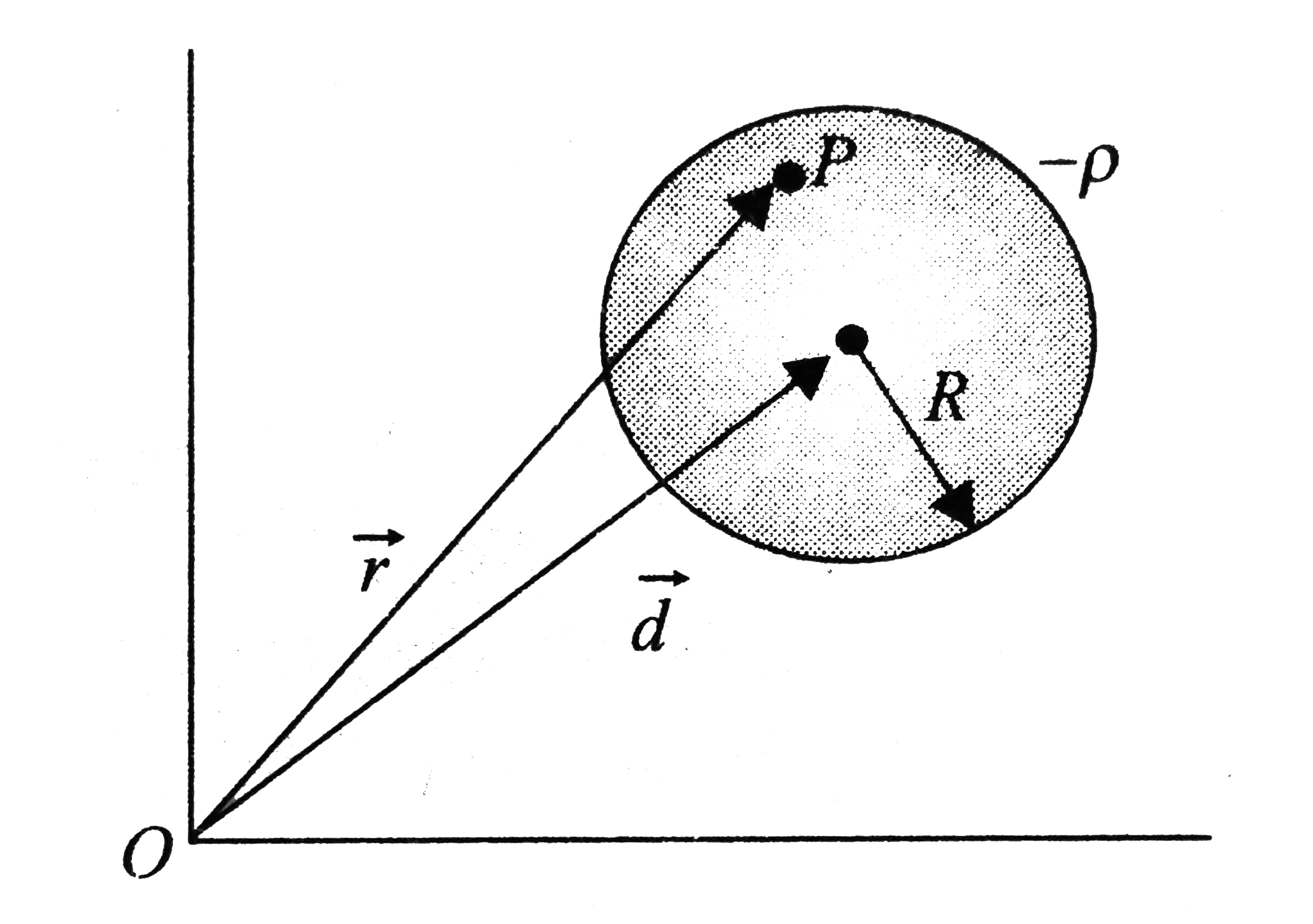 Aρ3ε0→dBρ3ε0(→r−→d)Cρ3ε0(→d−→r)Dρ3ε0(→r)
Aρ3ε0→dBρ3ε0(→r−→d)Cρ3ε0(→d−→r)Dρ3ε0(→r)
A non-conducting solid sphere has volume charge density that varies as ρ=ρ0 r, where ρ0 is a constant and r is distance from centre. Find out electric field intensities at following positions.
(i) r<R (ii) r≥R
View SolutionA solid uniformly charged ficed non-conducting sphere of total charge Q and radius R contains a tunnel of negligible diameter. If a point charge '-q' of mass 'm' is released at rest from point P as shown in figure then find out its velocity at following points

(i) At the surface of sphere
(ii) At the centre of the sphere
View SolutionDerive an expression for the electric field intensity at a point outside a charged conducting sphere.
View SolutionA solid sphere ofradius 'R' is uniformly charged with charge density ρin its volume. A spherical cavity of radius R2 is made in the sphere as shown in the figure. It is given that ρR2∈0=48V. Find the electric potential at the centre C of the sphere:
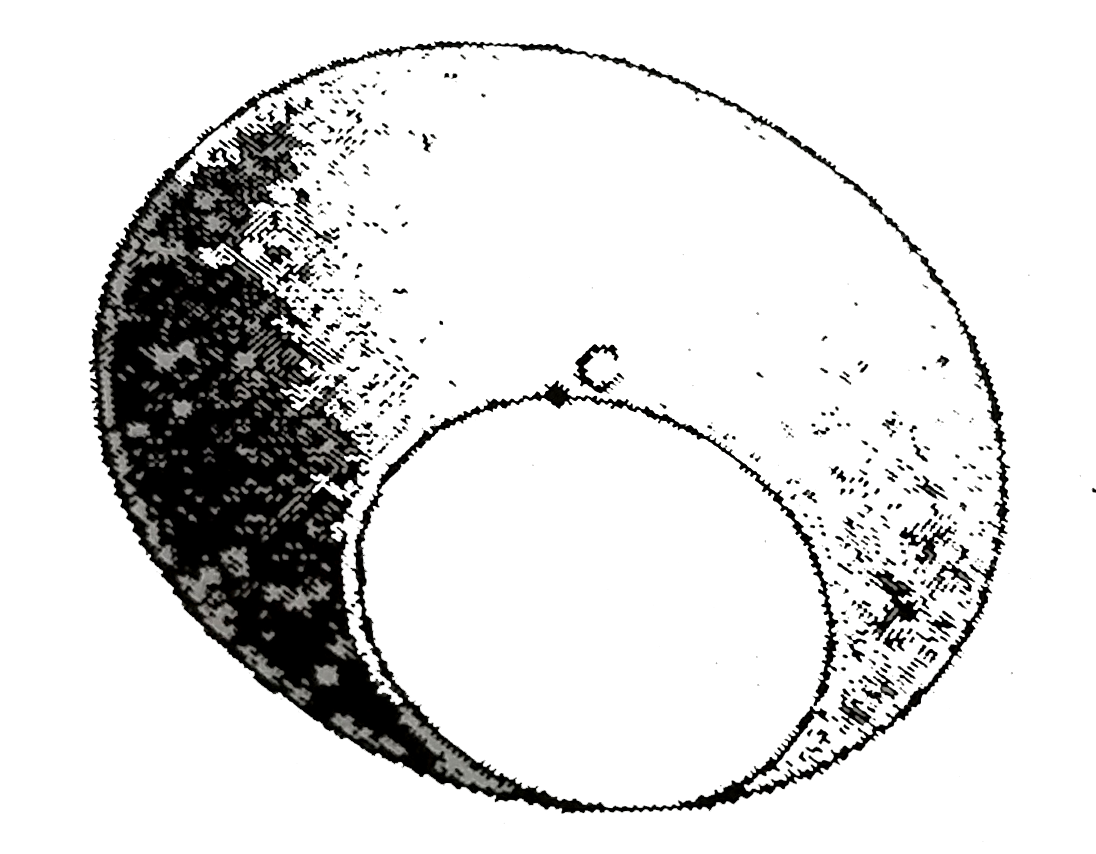
View SolutionA solid of radius 'R' is uniformly charged with charge density ρ in its volume. A spherical cavity of radius R2 is made in the sphere as shown in the figure. Find the electric potential at the centre of the sphere.

View SolutionFind out electric field intensity due to uniformly charged solid non-conducting sphere of volume charge density ρ and radius R at following points :
(i) At a distance r from surface of sphere (inside)
(ii) At a distance r from the surface of sphere (outside)
View SolutionFind out energy stored inside a solid non-conducting sphere of total charge Q and radius R. [Assume charge is uniformly distributed in its volume.]
View Solution