A solid non conducting sphere of radius R and uniform volume density ρ has centre at origin. Find out electric field intensity in vector form at following positions :
(i) (R, 0, 0) (ii) (0, 0, R/2) (iii) (R, R, R)
For uniformly charged non-conducting sphere, electric field inside the sphere :
→E=kQ→rR3=ρ→r3ε0(for r<R)
and electric field outside the sphere
→E0=KQr2.ˆr=14πε0.ρ43πR3r2ˆr=ρR33ε0r2.ˆr(for r≥R)
(i) (R, 0, 0) means it is at the surface →r=Rˆr and ˆr=ˆi
∴→E0=ρR33ε0R2(ˆi)=ρR3ε0.ˆi
(ii) (0,0,R2)
means point is inside the sphere
→r=R2ˆk ⇒ →E=ρR6ε0ˆk
(iii) For position (R, R, R)
→r=R(ˆi+ˆj+ˆk)⇒ ˆr=ˆi+ˆj+ˆk√3,r=R√3
means point (R, R, R) is outside the sphere
∴→E=ρR33ε0(3R2).(ˆi+ˆj+ˆk)√3=ρR9√3ε0(ˆi+ˆj+ˆk)
Topper's Solved these Questions
- BOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (A)9videos
ELECTROSTATICS
- BOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - Exercise-1 Section (B)12videos
ELECTROSTATICS
- BOOK - RESONANCECHAPTER - ELECTROSTATICSEXERCISE - HLP39videos
ELECTROSTATICS
- BOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - A.l.P19videos
ELECTROMAGNETIC INDUCTION
- BOOK - RESONANCECHAPTER - EXPERIMENTAL PHYSICSEXERCISE - PART -II10videos
EXPERIMENTAL PHYSICS
Similar Questions
A solid non conducting sphere of radius R and uniform volume charge density ρ has its centre at origin. Find out electric field intensity in vector form at following positions :
(i) (R/2,0,0) (ii) (R√2,R√2,0) (iii) (R,R,0)View SolutionA Uniformly charged solid non-conducting sphere of uniform volume charge density ρ and radius R is having a concentric spherical cavity of radius r. Find out electric field intensity at following points, as shown in the figure :

(i) Point A (ii) Point B
(iii) Point C (iv) Centre of the sphereView Solution
Knowledge Check
- Question 1 - Select One
A non-conducting solid sphere of radius R is uniformly charged. The magnitude of the electric filed due to the sphere at a distance r from its centre
AIncreases as r increases inside the sphereBDecreases as r increases inside as well as outsideCIncreases as increases outside the sphereDIs discontinuousat r=R - Question 2 - Select One or More
There is a solid non-conducting sphere of radius R. Sphere is uniformly charged over the volume. Electric field due to this where in observed.
AElectric field intensity at the centre of sphere can never be non-zero.BMagnitude of electric field intensity at a point inside the sphere is directly proportional to the distance of the point from centre of sphere.CMagnitude of electric field intensity is directly proportional to 1r2 for points outside the sphere where r is the distance of point from the centre of sphereDMagnitude of electric field intensity at any point is less than its magnitude on sphere's surface. - Question 3 - Select One or More
A non-conducting solid sphere of radius R is uniformly charged. The magnitude of the electric filed due to the sphere at a distance r from its centre
A(a) increases as r increases, for r<R.B(b) decreases as r increases, for 0<r<∞.C(c) decreases as r increases, for R<r<∞.D(d) is discontinuous at r=R.
There is a hemisphere of radius R having a uniform volume charge density ρ. Find the electric potential and field at the centre
View SolutionA sphere of radius 2R has a uniform charge density p. The difference in the electric potential at r = R and r = 0 from the centre is:
View SolutionA non-conducting solid sphere has volume charge density that varies as ρ=ρ0 r, where ρ0 is a constant and r is distance from centre. Find out electric field intensities at following positions.
(i) r<R (ii) r≥RView SolutionA nonconducting sphere of radius R is filled with uniform volume charge density −ρ. The center of this sphere is displaced from the origin by →d. The electric field →E at any point P having position vector inside the sphere is
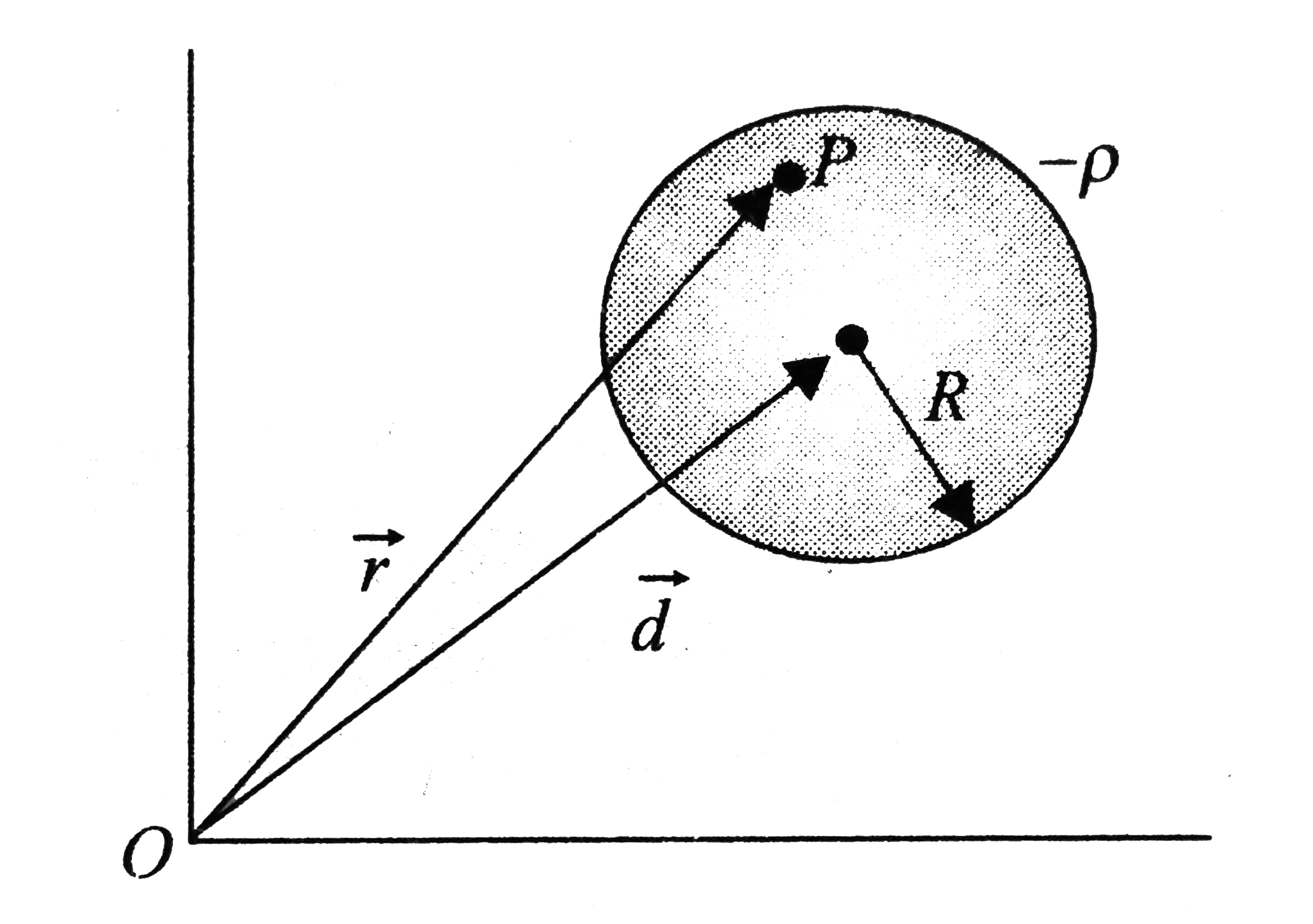 View Solution
View SolutionAn insulated sphere of radius R has a uniform volume charge density λ. The electric field at a point A, which is at distance r from its center is given by (R>r)
View Solution

