A square of side d, made from a thin insulating plate, is uniformly charged and carries a total charge of Q. A point charge q is placed on the symmetrical normal axis of the square at a distance d/2 from the plate. How large is the force acting on the point charge ?
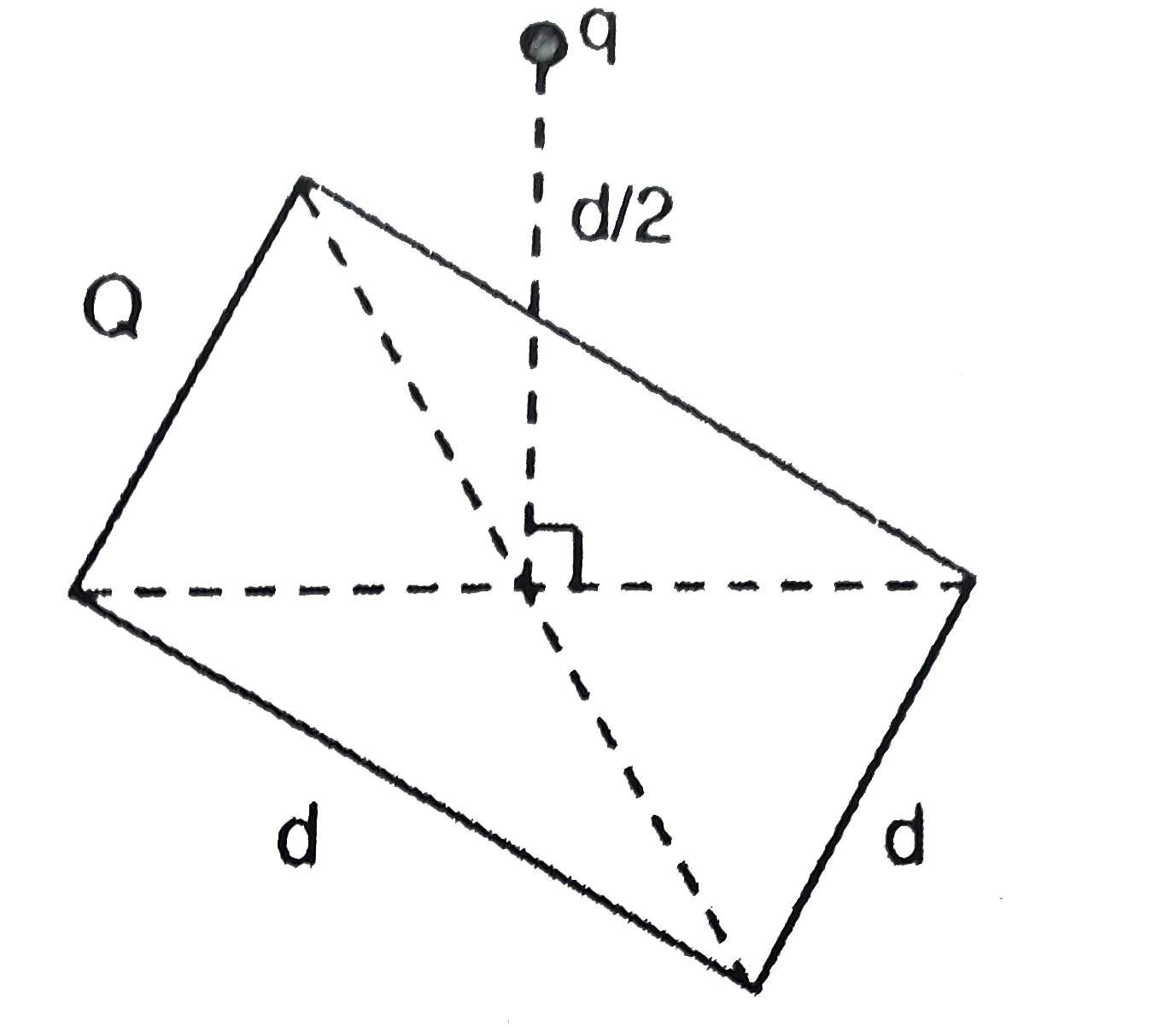
According to Newton's third law, the insulating plate acts on the point charge with a force of the same magnitude (but opposite direction) as the point charge does on the plate. We calculate the magnitude of this latter force.
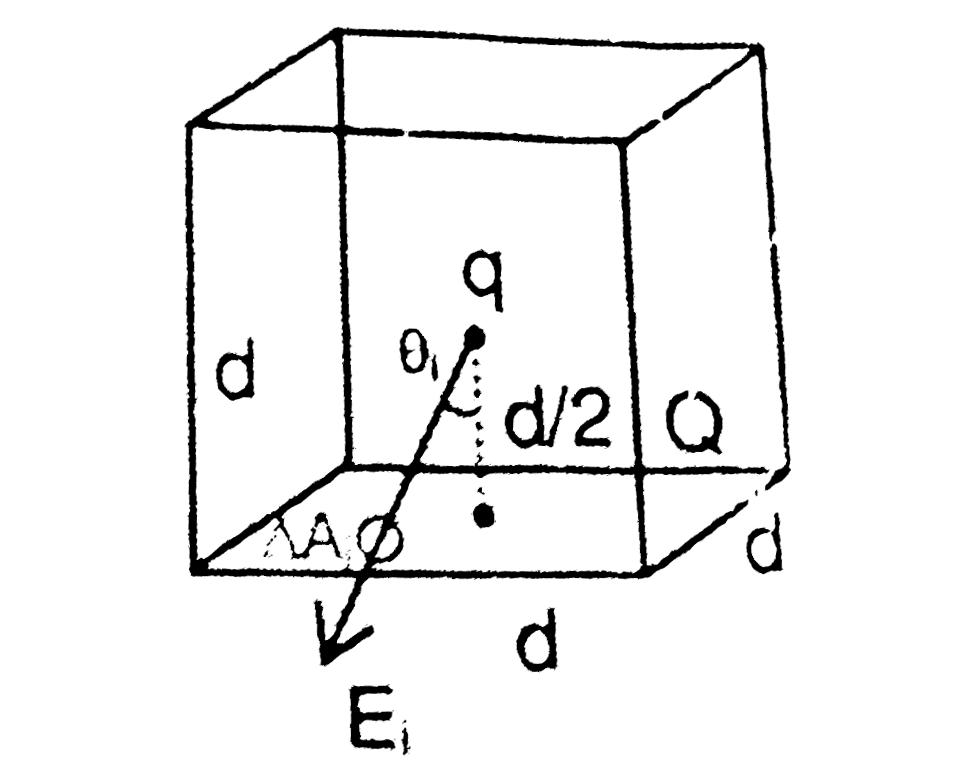
Divide the plate (notionally) inot small pieces, and denote the area of the ith piece by ΔAi. Because of the uniform charge distibution, the charge on this small piece is ΔQi=Qd2ΔAi. and so the electric force acting on it is Fi=EiQi, where Ei is the magnitude of the electric field produced by the point charge q at the position of the small piece.
. The force acting on the insulating plate, as a whole, can be calculated as the vector sum of the forces acting on the individual pieces of the plate. Because of the axial symmetery, the net force is erpendicular to the plate, and so it is sufficient to sum the perpendicular components of the forces: :br" F=∑iFicosθi=∑iEiqd2ΔAicosθi=Qd2∑iEiΔAicosθi
Where θi is the angle between the normal to the plate and the line that connects the point charge to the its piece of it.
The Sum in the given expression is nothing other than the electric flux through the square sheet produced by the point charge q:ψ=q6ε0
Using this and our previous observations, we calculate the magnitude of the force acting on the point charge due to the presence of the charged insulating plates as F=Qq6e0d2
Topper's Solved these Questions
Similar Questions
Figure shows a uniformly charged sphere of radius R and total charge Q. A point charge q is situated outside the sphere at a distance r from centre of sphere. Find out the following :
(i) Force acting on the point charge q due to the sphere.
(ii) Force acting on the sphere due to the point charge.

View SolutionFigure shows a uniformly charged sphere of radius R and total charge Q. A point charge q is situated outside the sphere at a distance r from centre of sphere. Find out the following :
(i) Force acting on the point charge q due to the sphere.
(ii) Force acting on the sphere due to the point charge.

View Solution
Knowledge Check
A charged spherical conductor of radius R carries a charge Q. a point charge q is placed at an outside point at a distance x from the surface of the sphere. The force experienced by the point charge will be proportional to
A1x2B(R+x)2C1(R+x)2D1(R−x)2A uniformly charged square plate having side L carries a uniform surface charge density σ. The plate lies in the y−z plane with its centre at the origin. A point charge q lies on the x-axis. The flux of the electric field of q through the plate is ϕ0, while the foce on the point charge q due to the plate is F0, along the x-axis. Then,
Aσ=F0ϕ0LBσ=F0ϕ0Cσ=F0Lϕ0Dσ=ϕ0F0A point charge q is placed at a distance a/2 directly above the center of a square of side a. The electric flux through the square is
Aqε0Bqπε0Cq4ε0Dq6ε0
A uniformly charged square plate having side L carries a uniform surface charge density σ. The plate lies in the y−z plane with its centre at the origin. A point charge q lies on the x-axis. The flux of the electric field of q through the plate is ϕ0, while the foce on the point charge q due to the plate is F0, along the x-axis. Then,
View SolutionA point charge q is placed at a distance a/2 directly above the centre of a square of side a. The electric flux through the square is
View SolutionConsider a thin conducting shell of radius R carrying total charge Q. Two point charges Q and 2Q are placed on points A and B, which are at a distances of R2 and 2R from the center of the cell respectively as shown in the figure. If the cell is earthed how much charge will flow to the earth?
View SolutionA point charge +q is placed at a distance d from an isolated conducting plane. The field at a point P on the other side of plane is
View SolutionConsider a thin conducting shell of radius R carrying total charge Q. Two point charges Q and 2Q are placed on points A and B, which are at a distances of R2 and 2R from the center of the cell respectively as shown in the figure. If the cell is earthed how much charge will flow to the eart?

View Solution

