Two sides of a triangle are parallel to the coordinate axes. If the slopes of the medians through the acute angles of the triangle are 2 and m, the m= 12 (b) 2 (c) 4 (d) 8
1/2
2
4
8
The correct Answer is:A, D
m1=Slope of AD=−a2b
m2=Slope of BE=−2ab
∴m1m2=14
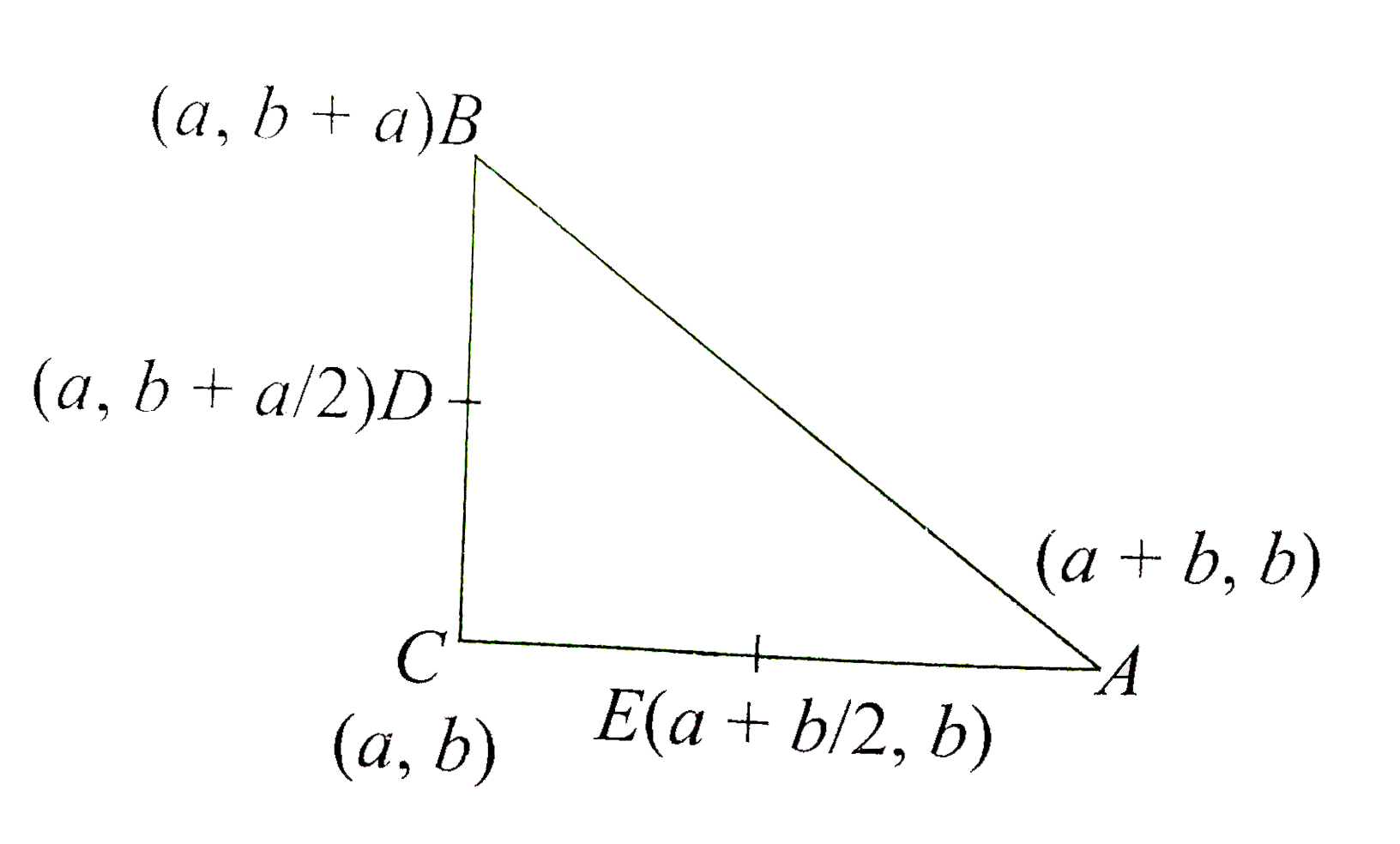
If m1=2, then m2=m=8
If m2=2,then m1=m=1/2.
Topper's Solved these Questions
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Comprehension)27videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Matrix)8videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINESEXERCISE - Exercise (Single)82videos
STRAIGHT LINES
- BOOK - CENGAGECHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGECHAPTER - THEORY OF EQUATIONSEXERCISE - JEE Advanced Previous Year9videos
THEORY OF EQUATIONS
Similar Questions
The lengths of the medians through acute angles of a right-angled triangle are 3 and 4. Find the area of the triangle.
View SolutionTwo sides of a triangle are along the coordinate axes and the medians through the vertices (other than the origin) are mutually perpendicular. The number of such triangles is/are zero (b) two (c) four (d) infinite
View Solution
Knowledge Check
- Question 1 - Select One
The vertices of a triangle are A (3,-2), B (-2, 1) and C (5,2). Then the length of the median through B is
A√67 unitsB√37 unitsC√35 unitsD6 units - Question 2 - Select One
If a plane meets the coordinate axes at A,B and C such that the centroid of the triangle is (1,2,4) then the equation of the plane is
Ax+2y+4z=12B4x+2y+z=12Cx+2y+4z=3D4x+2y+z=3 - Question 3 - Select One
If a plane meets the coordinate axes at A, B and C such that the centroid of the triangle is (1,2,4),then the equation of the plane is
Ax+2y+4z=12B4x+2y+z=12Cx+2y+4z=3D4x+2y+z=3
The sides of a triangle are m, n and √m2+n2+mn., What is the sum of the acute angles of the triangle?
View SolutionIt is desired to construct a right angled triangle ABC (∠C=π2) in x-y plane so that its sides are parallel to coordinates axis and the medians through A and B lie on the lines y=3x+1 and y=mx+2 respectively. The values of m for which such a triangle is possible is/are
View SolutionIf a2+b2+c2=ab+bc+ca where a, b, c are the sides of a triangle, then the largest angle of that triangle is
View SolutionA triangle must have (1) Exactly two acute angles (2) At least two acute angles (3) Exactly three acute angles (4) At most one acute angle
View SolutionIf two sides of an obtuse angled triangle are 8 cm and 15 cm and third sides is x, then
View Solution

