A heavy vehicle moving with velocity 15 m/s strikes an object of very small mass at rest head on elastically. Velocity of object after collision is
15 m/s
25 m/s
20 m/s
30 m/s
The correct Answer is:D
To solve the problem of a heavy vehicle colliding elastically with a small mass at rest, we can follow these steps:
Step 1: Understand the Problem
We have a heavy vehicle (mass M) moving with a velocity of 15m/s that collides head-on with a small object (mass m) at rest. We need to find the velocity of the small object after the collision.
Step 2: Apply Conservation of Momentum
In an elastic collision, the total momentum before the collision is equal to the total momentum after the collision. The equation can be written as:
M⋅15+m⋅0=M⋅V1+m⋅V2
Where:
- V1 is the velocity of the heavy vehicle after the collision.
- V2 is the velocity of the small object after the collision.
This simplifies to:
15M=M⋅V1+m⋅V2(1)
Step 3: Apply Conservation of Kinetic Energy
In an elastic collision, the total kinetic energy before the collision is equal to the total kinetic energy after the collision. The equation can be written as:
12M(15)2+12m(0)2=12MV21+12mV22
This simplifies to:
12M(15)2=12MV21+12mV22(2)
Step 4: Use the Velocity of Separation and Approach
For elastic collisions, the relative velocity of separation equals the relative velocity of approach:
V2−V1=15(3)
Step 5: Solve the Equations
From equation (3), we can express V1 in terms of V2:
V1=V2−15(4)
Now substitute equation (4) into equation (1):
15M=M(V2−15)+mV2
Expanding this gives:
15M=MV2−15M+mV2
Rearranging terms:
15M+15M=MV2+mV2
30M=(M+m)V2
Step 6: Approximate for Large Mass
Since M is much larger than m, we can approximate M+m≈M:
30M=MV2
Dividing both sides by M:
V2=30m/s
Conclusion
The velocity of the small object after the collision is 30m/s.
---
We have a heavy vehicle (mass M) moving with a velocity of 15m/s that collides head-on with a small object (mass m) at rest. We need to find the velocity of the small object after the collision.
In an elastic collision, the total momentum before the collision is equal to the total momentum after the collision. The equation can be written as:
- V1 is the velocity of the heavy vehicle after the collision.
- V2 is the velocity of the small object after the collision.
In an elastic collision, the total kinetic energy before the collision is equal to the total kinetic energy after the collision. The equation can be written as:
For elastic collisions, the relative velocity of separation equals the relative velocity of approach:
From equation (3), we can express V1 in terms of V2:
Since M is much larger than m, we can approximate M+m≈M:
The velocity of the small object after the collision is 30m/s.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBook:AAKASH INSTITUTEChapter:WORK, ENERGY AND POWERExercise:Assignment (SECTION - A)
Explore 67 Videos
- WORK, ENERGY AND POWERBook:AAKASH INSTITUTEChapter:WORK, ENERGY AND POWERExercise:Assignment (SECTION - B)
Explore 39 Videos
- WORK, ENERGY AND POWERBook:AAKASH INSTITUTEChapter:WORK, ENERGY AND POWERExercise:SECTION-J (AAKASH CHALLENGERS QUESTIONS)
Explore 14 Videos
- WAVESBook:AAKASH INSTITUTEChapter:WAVESExercise:ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
Explore 12 Videos
Similar Questions
Two identical bodies A and B moving with velocities 13 m/s and - 15 m/s respectively collide head-on elastically. What will be their velocities after collision?
View SolutionA ball of mass m moving with velocity u strikes another identical ball at rest. Find the velocities of the balls after the collision if the coefficient of restitution is e and the collision is head -on. Also , calculate the loss in K.E.
View Solution
Knowledge Check
A heavy vehicle moving with velocity 15 m/s strikes an object of very small mass at rest head on elastically. Velocity of object after collision is
A15 m/sB25 m/sC20 m/sD30 m/sA body of mass a moving with a velocity b strikes a body of mass c and gets embered into it. The velocity of the systems after collision is
Aa+cabBaba+cCab+cDaa+bAn object moving with a velocity of 50 ms−1 comes to rest after 20 s. What is its initial velocity ?
A0ms−1B50ms−1C60ms−1D10 ms−1
A particle of mass m1 moving on a smooth surface with some velocity strikes another particle of mass m2 at rest. The head - on elastic collision takes place. After the collision , particles move with equal speed in opposite direction . Determine the mass ratio.
View SolutionA body of mass m moving with a velocity v is approaching a second object of same mass but at rest. The kinetic energy of the two objects as viewed from the centre of mass is
View SolutionA particle of mass m moving with velocity v0 collides with sphere of same mass at rest , as shown. If the surface of contact is smooth and the collision is elastic, find the velocities of particle and sphere after the collision.
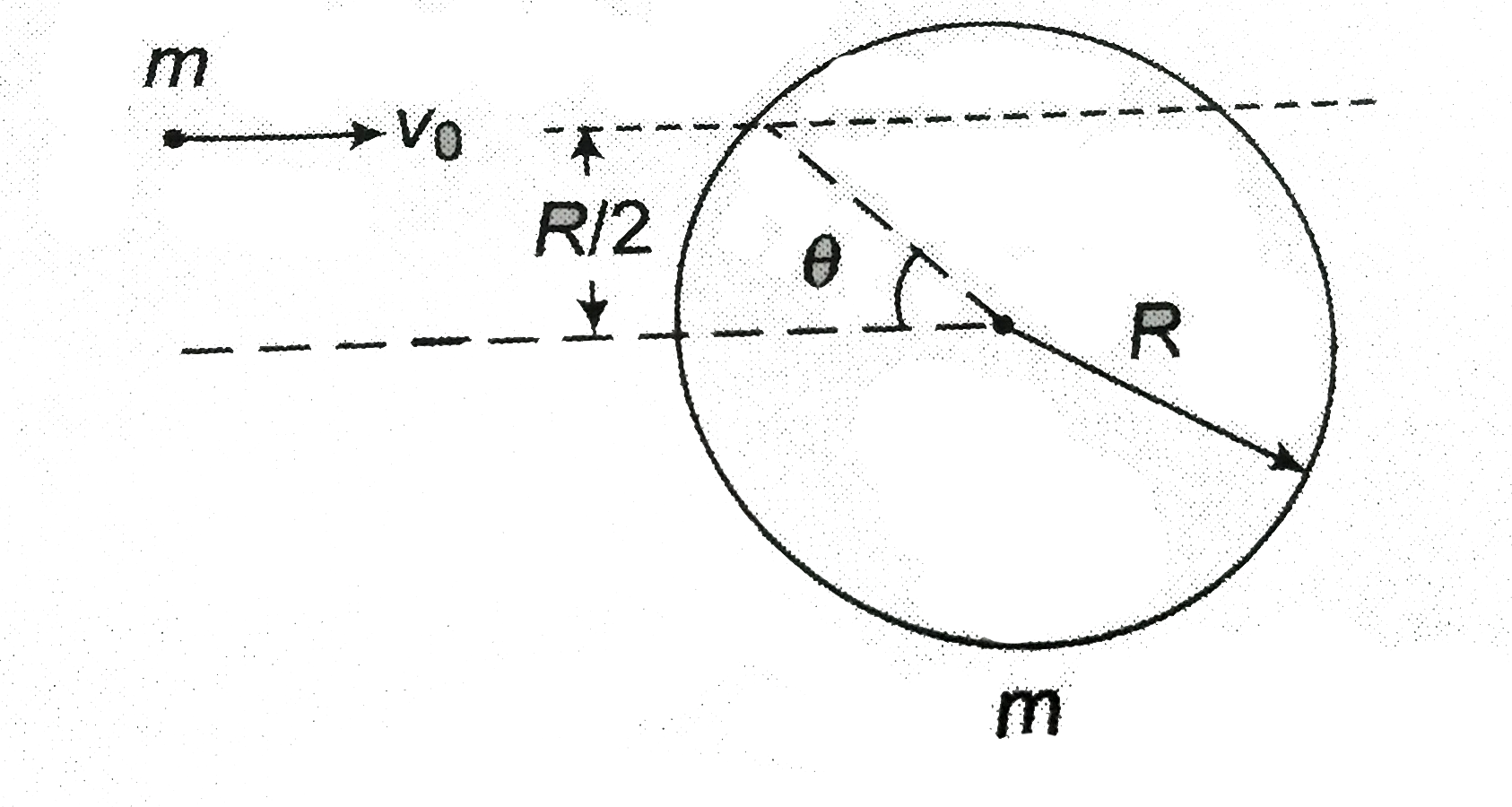
View SolutionA particle of mass m moving with some velocity on a smooth surface strikes another particle of mass ηm at rest , elasticity head - on. What fraction of incident K.E. is transferred to the particle of mass ηm.
View SolutionA heavy truck moving with a velocity of 60km/h collides with a light drum at rest. If the collision is elastic, then the velocity of the drum immediately after collision will be
View Solution