a sphere rolling with velocity V collides a vertical wall. The friction cefficients μ1,μ2 are as shown. Mathc the column, analysing for just after the collision.
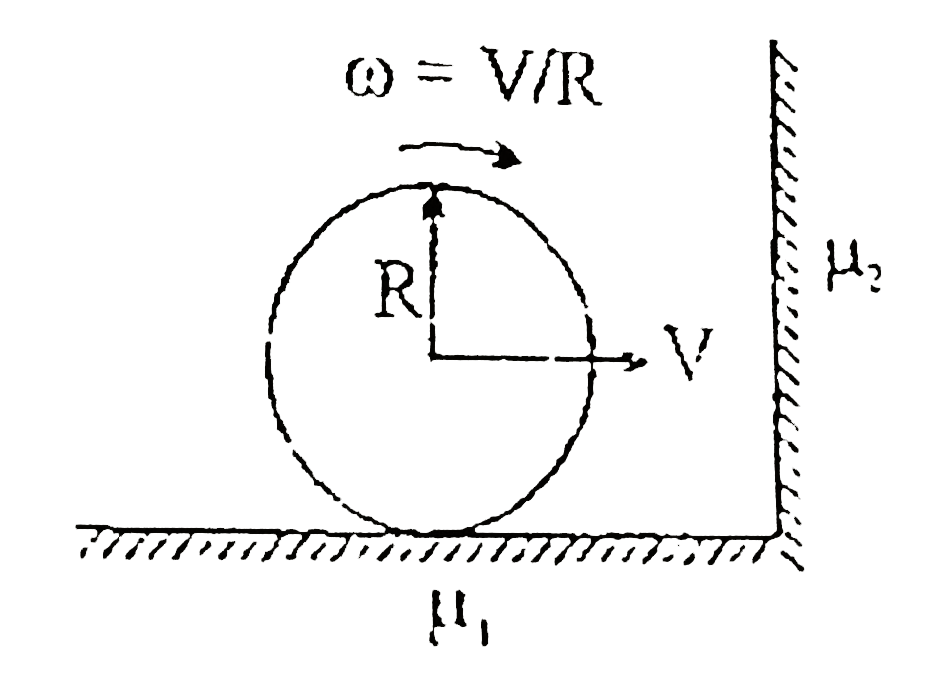
Mechanical energy is lost in the form of sound. Think of the torque due to impulsive forces.
Topper's Solved these Questions
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (A)
32 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART (B)
3 Videos
- TEST PAPERSBOOK - BANSALCHAPTER - TEST PAPERSEXERCISE - PHYSICS PART- C
4 Videos
- SEMICONDUCTORSBOOK - BANSALCHAPTER - SEMICONDUCTORSEXERCISE - CBSE Question
32 Videos
Similar Questions
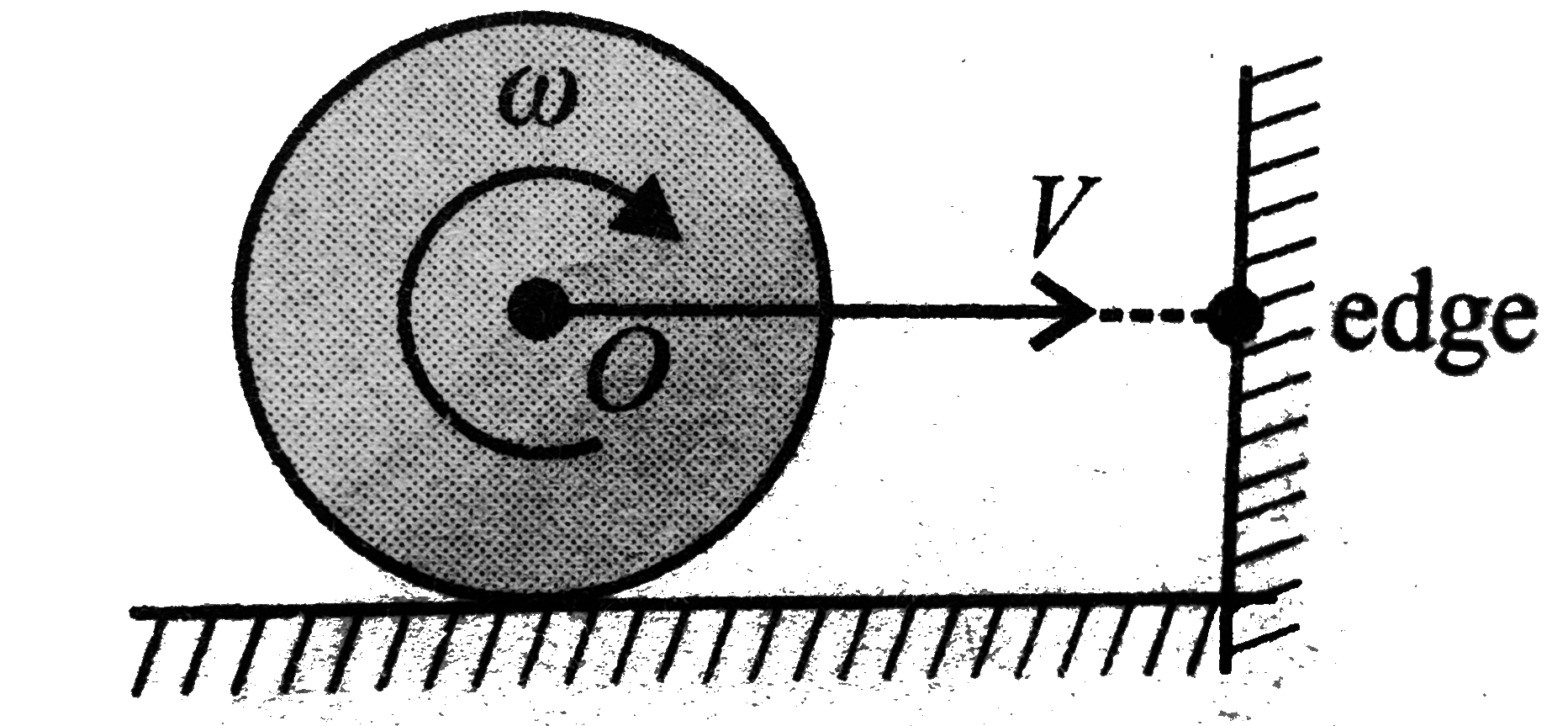
A uniform solid sphere of radius r is rolling on a smooth horizontal surface with velocity V and angular velocity ω(V=ωr). The sphere collides with a sharp edge on the wall as shown in Fig. The coefficient of friction between the sphere and the edge μ=1/5. Just after the collision the angular velocity of the sphere becomes equal to zero. The linear velocity of the sphere just after the collision is equal to
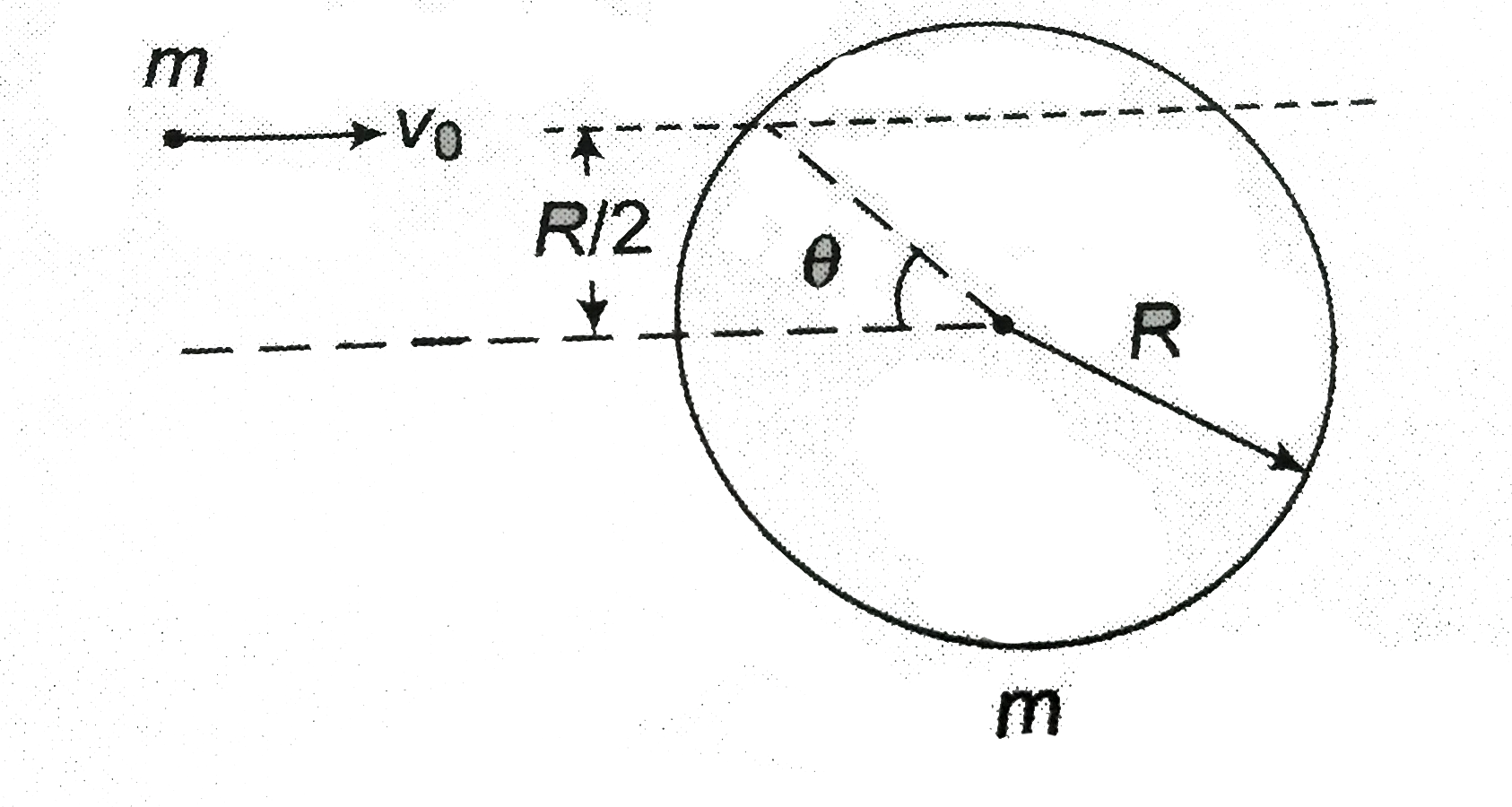
View SolutionA particle of mass m moving with velocity v0 collides with sphere of same mass at rest , as shown. If the surface of contact is smooth and the collision is elastic, find the velocities of particle and sphere after the collision.
View Solution
Knowledge Check
A uniform solid sphere of radius r is rolling on a smooth horizontal surface with velocity V and angular velocity ω=(V=ωr). The sphere collides with a sharp edge on the wall as shown in Fig. The coefficient of friction between the sphere and the edge μ=1/5. Just after the collision the angular velocity of the sphere becomes equal to zero. The linear velocity of the sphere just after the collision is equal to
 AVBV5C3V5DV6
AVBV5C3V5DV6A uniform solid sphere of radius r is rolling on a smooth horizontal surface with velocity v and angular velocity ω=(v=ωr). The sphere collides with a sharp edge on the wall as shown in figure. The coefficient of friction between the sphere and the edge μ=1/5. Just after the collision the angular velocity of the sphere becomes equal to zero. The linear velocity of the surface just after the collision is equal to
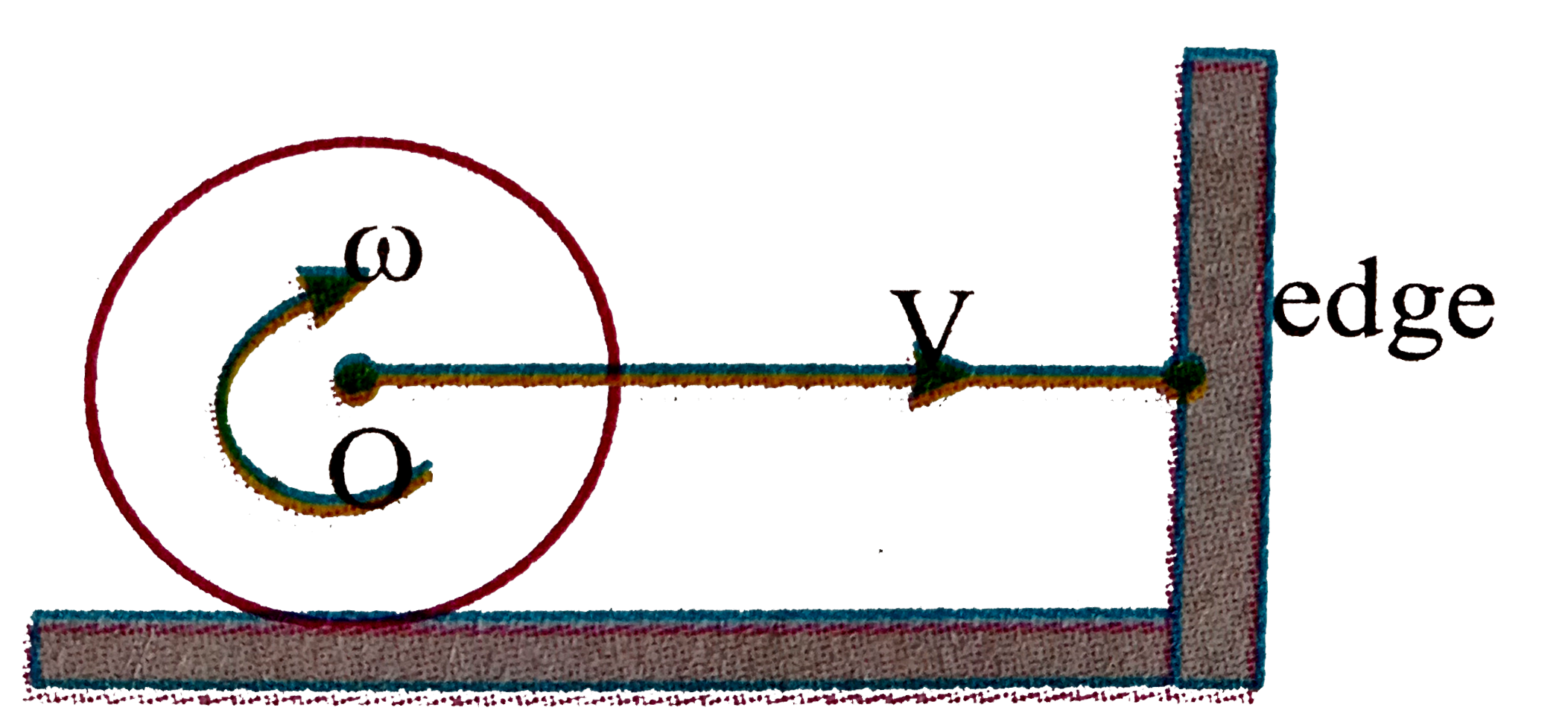 AvBv5C3v5Dv6
AvBv5C3v5Dv6A solid sphere has linear velocity v0=4 m/s and angular velocity ω0=9 rad/s as shown. Ground on which it is moving , is smooth . It collides elastically with a rough wall of coefficient of friction μ . Radius of the sphere is 1m and mass is 2 kg .

If the sphere after colliding with the wall rolls without slipping on opposite direction, then coefdicient of friction μ isA12B23C13D14
A solid sphere has linear velocity v0=4 m/s and angular velocity ω0=9 rad/s as shown. Ground on which it is moving , is smooth . It collides elastically with a rough wall of coefficient of friction μ . Radius of the sphere is 1m and mass is 2 kg .

If the sphere after colliding with the wall rolls without slipping on opposite direction, then coefdicient of friction μ is
View SolutionA sphere rolling on a horizontal rough surface Collides elastically with a smooth vertical wall, as shown in Fig. State which of the following statements is true or false.
 a. After collision, the velocity of the centre of mass gets reversed.
a. After collision, the velocity of the centre of mass gets reversed.
b. Angular momentum of the sphere about the point of contact with the wall is conserved.
c. Angular momentum of the sphere about a stationary point on the horizontal surface is conserved.
d. Just after collision the point of contact with the horizontal surface is moving towards the wall.
e. After collision the friction force acts on the sphere such that it decreases the linear speed and increases the angular speed.
f. Finally, when the sphere starts rolling, it is moving away from the wall.
View SolutionA sphere rolling on a horizontal rough surface Collides elastically with a smooth vertical wall, as shown in Fig. State which of the following statements is true or false.
 a. After collision, the velocity of the centre of mass gets reversed.
a. After collision, the velocity of the centre of mass gets reversed.
b. Angular momentum of the sphere about the point of contact with the wall is conserved.
c. Angular momentum of the sphere about a stationary point on the horizontal surface is conserved.
d. Just after collision the point of contact with the horizontal surface is moving towards the wall.
e. After collision the friction force acts on the sphere such that it decreases the linear speed and increases the angular speed.
f. Finally, when the sphere starts rolling, it is moving away from the wall.
View SolutionA small uniform solid sphere A rolls down a fixed surface starting at a height h and collides elastically with a sphere B which is identical in size to A but has twice its mass. The speed of the sphere B, just after the collision is

View SolutionA small uniform solid sphere A rolls down a fixed surface starting at a height h and collides elastically with a sphere B which is identical in size to A but has twice its mass. The speed of the sphere B, just after the collision is

View Solution
