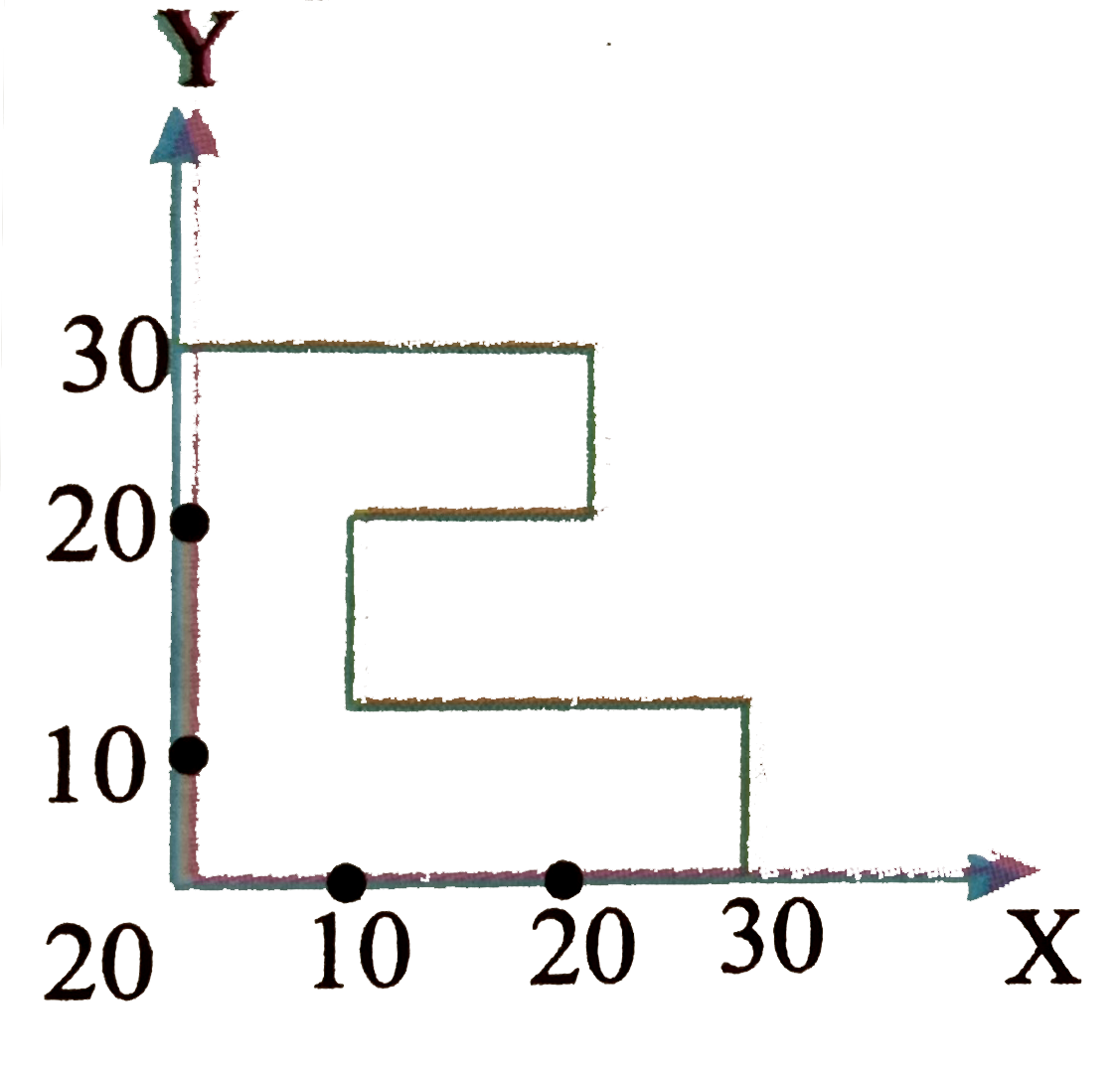
The coordinate of centre of mass of letter E which is cut from a uniform metal sheet are (take origin at bottom left corner and width of letter 2cm every where)

(2cm,4cm)
(2.4 cm , 5 cm)
(3 cm , 5cm)
(3.3cm,5cm)
The correct Answer is:B
xcm=m1x1+m2x2+m3x3+m4x4m1+m2+m3+m4
ycm=m1y1+m2y2+m3y3+m4y4m1+m2+m3+m4
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - III
43 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - IV
39 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NARAYNACHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - EXERCISE - II (C.W)
60 Videos
- SYSTEM OF PARTICLESBOOK - NARAYNACHAPTER - SYSTEM OF PARTICLESEXERCISE - Level-VI
78 Videos
- THERMAL PROPERTIES OF MATTERBOOK - NARAYNACHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - LEVEL - II (H.W.)
19 Videos
Similar Questions
Find the x coordinate of the centre of mass of the non - uniform rod of length L givne below. The origin is taken at the left end of the rod. The density of the rod as a function of its x- coordinates is ρ=ax2+bx+c, where a, b and c are constants.

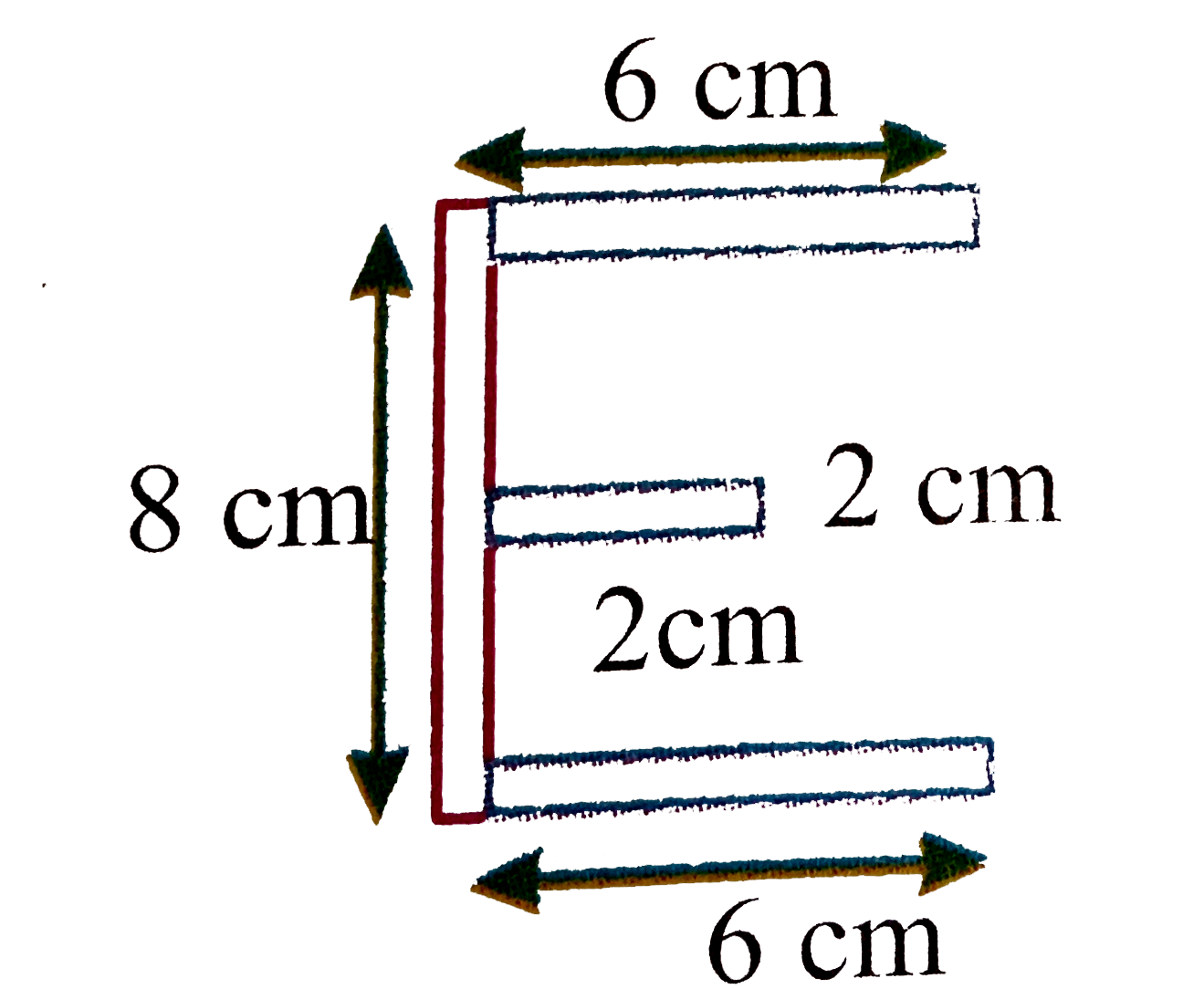
View SolutionA uniform piece of metal sheet is cut in the form as shown in the figure. Locate the centre of mass of the piece.
View Solution
Knowledge Check
The co-ordinates of centre of mass of letter E which is cut from a uniform metal sheet are (take origin at bottom left corner and width of letter 2cm every where)
 A(2cm,4cm)B(2.4cm,5cm)C(3cm,5cm)D(3.3cm,4cm)
A(2cm,4cm)B(2.4cm,5cm)C(3cm,5cm)D(3.3cm,4cm)The centre of mass of the letter F which is cut from a uniform metal sheet from point A is
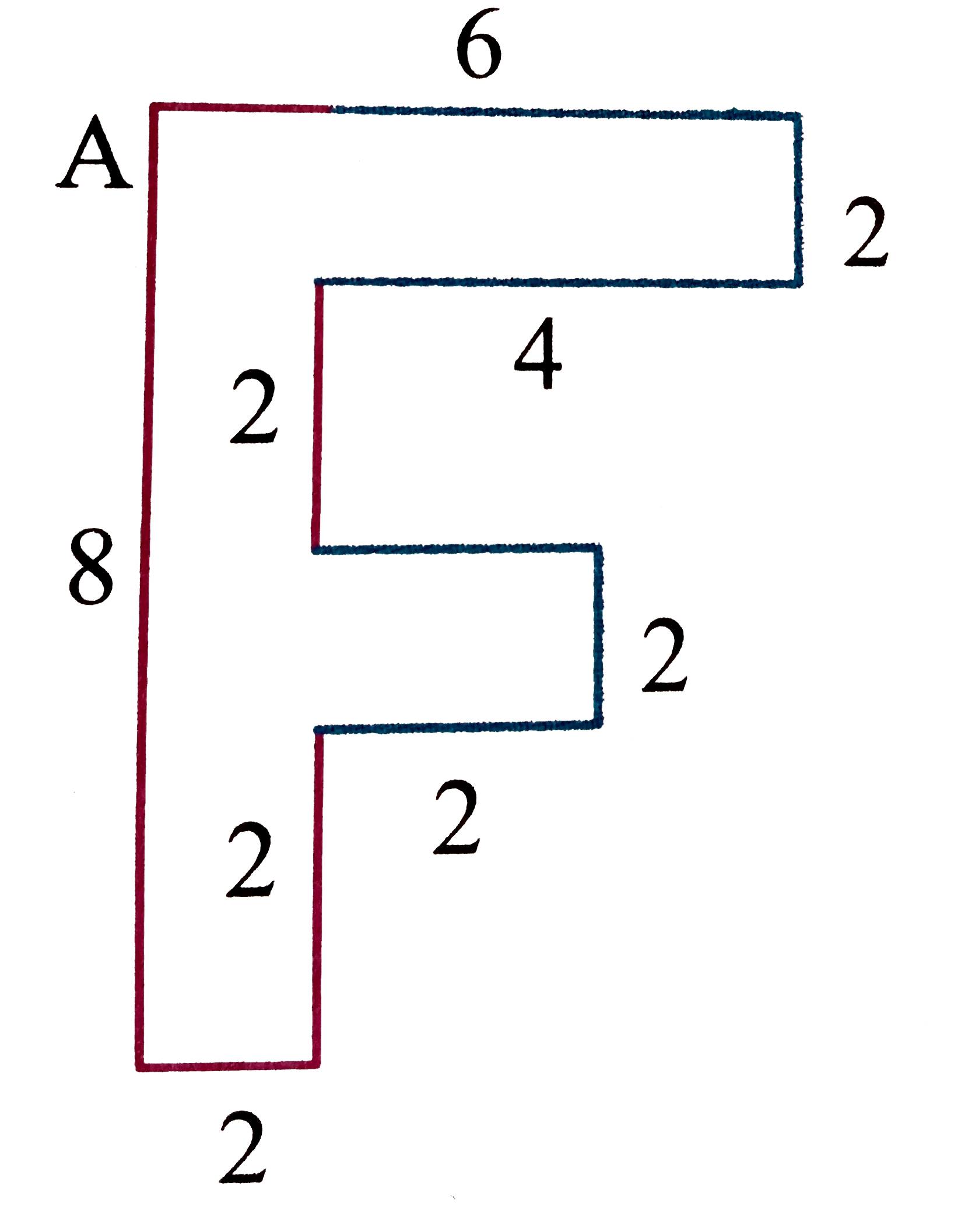 A15/7,33/7B15/7,23/7C22/7,33/7D33/7,22/7
A15/7,33/7B15/7,23/7C22/7,33/7D33/7,22/7Find the x coordinate of the centre of mass of the non - uniform rod of length L givne below. The origin is taken at the left end of the rod. The density of the rod as a function of its x- coordinates is ρ=ax2+bx+c, where a, b and c are constants.
 A2aL2+3bL2+6cL2(3aL2+4bL+8c)B4aL3+3bL2+2cL2(3aL2+2bL+c)C3aL2+4bL2+2cL4aL2+6bL+8cD3aL3+4bL2+6cL2(2aL2+3bL+6c)
A2aL2+3bL2+6cL2(3aL2+4bL+8c)B4aL3+3bL2+2cL2(3aL2+2bL+c)C3aL2+4bL2+2cL4aL2+6bL+8cD3aL3+4bL2+6cL2(2aL2+3bL+6c)
A disc of radius a2 is cut out from a uniform disc of radius a as shown in figure . Find the X Coordinate of centre of mass of remaining portion
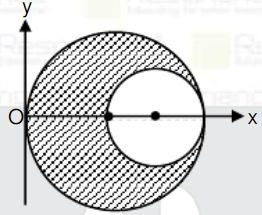
View SolutionFind the centre of mass of a thin, uniform disc of radius R from which a small concentric disc of radius r is cut.
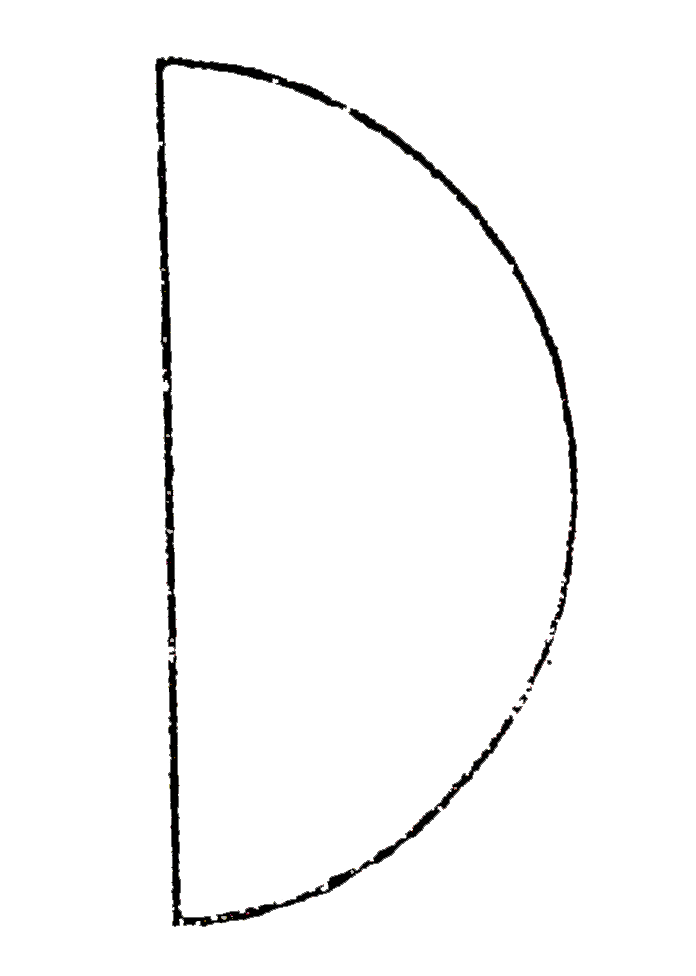
View SolutionFind the coordinates of centre of mass of a uniform semicircular closed wire frame with respect to the origin which is at its centre. Radius of the circular portion is R
View SolutionThe number of permutations of the letters a1,a2,a3,a4,a5 in which the first letter a1 does not occupy the first position (from the left) and the second letter a2 does not occupy the second position (from the left) is
View SolutionA square sheet of side 2 cm is cut from each corner of a rectangular piece of an aluminium sheet of length 12 cm and breadth 8 cm. Find the area of the leftover aluminium sheet.
View Solution
