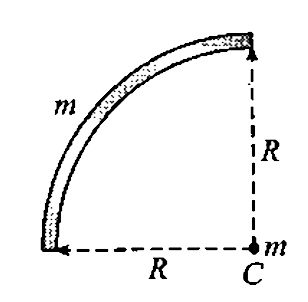
" The force of attraction on a particle of mass "m" placed at the centre of curvature of a semicircular wire of length L and mass "M" is "
Step by step video & image solution for " The force of attraction on a particle of mass "m" placed at the centre of curvature of a semicircular wire of length L and mass "M" is " by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
Knowledge Check
- Question 1 - Select One
The potential energy of interaction between the semi-circular ring of mass M and radius R, and the particle of mass M placed at the centre of curvature of the semi-circular arc is:
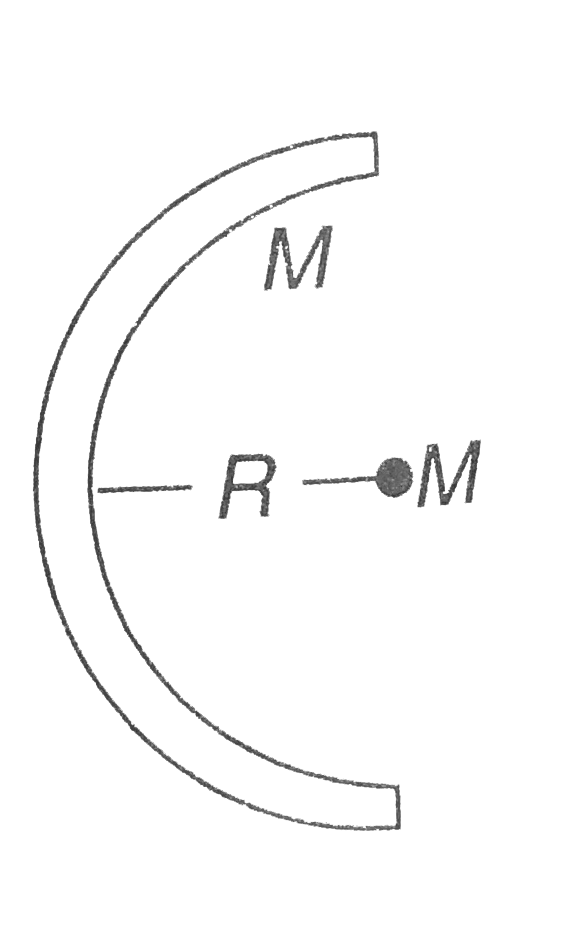 A−GM2RB−2GM2RC−GM2πRDnone of these
A−GM2RB−2GM2RC−GM2πRDnone of these - Question 2 - Select One
A particle of mass m is placed on centre of curvature of a fixed, uniform semi-circular ring of radius R and mass M as shown in figure. Calculate:
(a) interaction force between the ring and the particle and
(b) work required to displace the particle from centre of curvature to infinity.
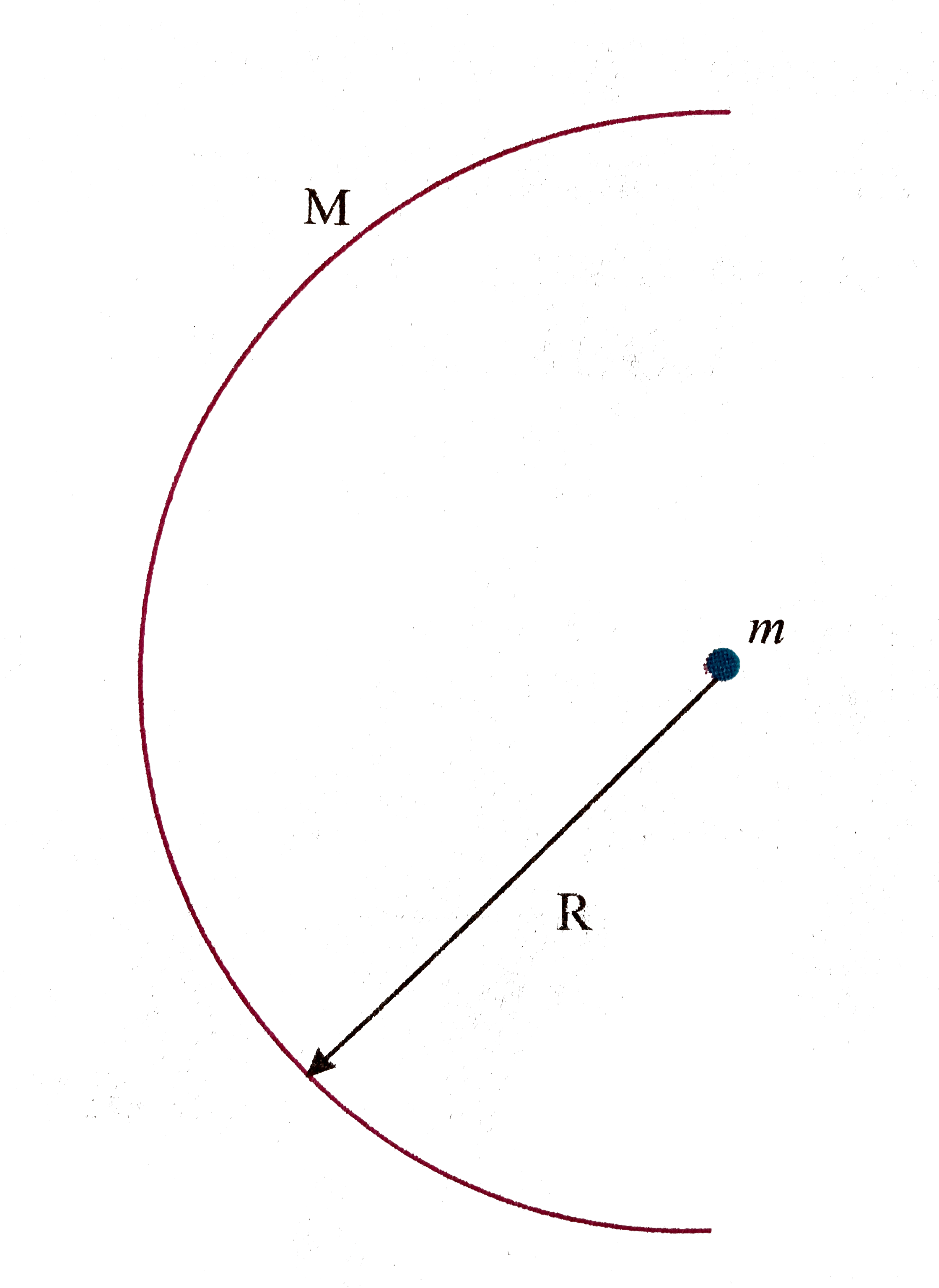 A(a)F=2GMπR2,(b)GMRB(a)F=2GMmπ2R,(b)GMmR2C(a)F=−2GMmπR2(b)−GMmRD(a)F=2GMmπR2(b)GMmR
A(a)F=2GMπR2,(b)GMRB(a)F=2GMmπ2R,(b)GMmR2C(a)F=−2GMmπR2(b)−GMmRD(a)F=2GMmπR2(b)GMmR - Question 3 - Select One
Four particles each of mass m are placed at the vertices of a square of side l. the potential at the centre of square is
A−2√2GmlB−3√2GmlC−2GmlD−4√2Gml
Find the coordination of the centre of mass of a uniform semicircular wire of radius R and mass M.
View SolutionFind the force of attraction between a solid sphere of mass M and a ring of mass m as shown.
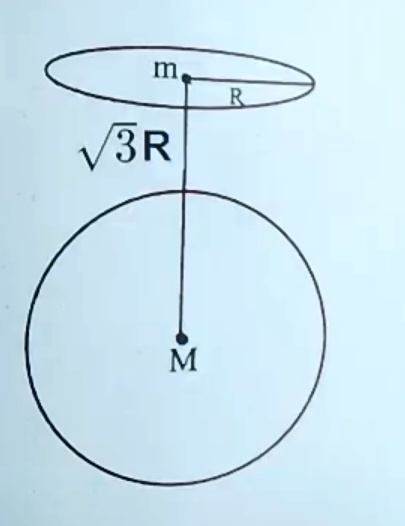 View Solution
View SolutionA thin rod of mass M and length L is bent in a semicircle as shown in figure (a). What is its gravitational force (both magnitude and direction) on a particle with mass m at O the centre of curvature ? (b) what would be the force on m if the rod is in the form of complete circle?
View SolutionA small point mass m is placed at the centre of curvature of acircular are of radius R and mass 3 m as shown in figure−6.31.Find the net gravitational force acting on the point mass.
 View Solution
View Solutionfind the gravitational force of attraction between a uniform sphere of mass M and a uniform rod of length L and mass m, placed such that r is the distance between the centre of the sphere and the near end of the road.
View Solution