In Young' s double-slit experiment, separation between the two slits is d and separation between plane of the slits and the screen is D. A small hole is made on the screen at a point directly in front of one of the slit. Some wavelengths are missing from the light coming out from hole on the other side. These wavelengths are
d2D
d22D
d23D
d24D
The correct Answer is:A, C
Let there be a point on the screen at a distance y from the centre. Path difference for this point can be written as follows:
Δx=ydD
For the point directly in front of one of the slits y=d/2, hence on substituting we get path difference as follows:
Δx=ydD=(d2)dD
⇒Δx=d22D
There will be destructive interference of those wavelengths which are missing, at this point and condition for destructive interference can be written as follows:
Δx=(2n+1)λ2
Here, λ is wavelength od light
⇒d22D=(2n+1)λ2
⇒λ=d2(2n+1)D
We can substitute n=0,1,2,3,............
⇒λ=d2D,d23D,d25D,.....
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - WAVE OPTICALEXERCISE - OBJECTIVE TYPE QUESTIONS (D. MULTIPLE CHOICE QUESTIONS)7videos
WAVE OPTICAL
- BOOK - MODERN PUBLICATIONCHAPTER - WAVE OPTICALEXERCISE - OBJECTIVE TYPE QUESTIONS (ASSERTION REASON TYPE QUESTIONS)10videos
WAVE OPTICAL
- BOOK - MODERN PUBLICATIONCHAPTER - WAVE OPTICALEXERCISE - OBJECTIVE TYPE QUESTIONS (JEE (ADVANCED) FOR IIT ENTRANCE)6videos
WAVE OPTICAL
- BOOK - MODERN PUBLICATIONCHAPTER - SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS EXERCISE - CHAPTER PRACTICE TEST FOR BOARD EXAMINATION12videos
SEMICONDUCTOR ELECTRONICS METERIALS DEVICES AND SIMPLE CIRCUITS
Similar Questions
In Young's double-slit experiment, the separation between the slits is halved and the distance between the slits and the screen in doubled. The fringe width is
View SolutionIn Young's double slit experiment, the separation between the slits is halved and the distance between the slits and the screen is doubled. The fringe width is
View Solution
Knowledge Check
- Question 1 - Select One
In Young's double-slit experiment, the separation between the slits is halved and the distance between the slits and the screen in doubled. The fringe width is
AunchangedBhalvedCdoubledDquadrupled - Question 2 - Select One
In Young's double slit experiment, the separation between the slits is halved and the distance between the slits and screen is doubled. The fringe-width will :
AunchangedBhalvedCdoubledDquadrupled - Question 3 - Select One
In Young's double slit experiment, the separation between the slits is halved and the distance between the slits and screen is doubled. The fringe-width will :
AunchangedBhalvedCdoubledDquadrupled
At a point on the screen directly in front of one of the slits, find the missing wavelengths.
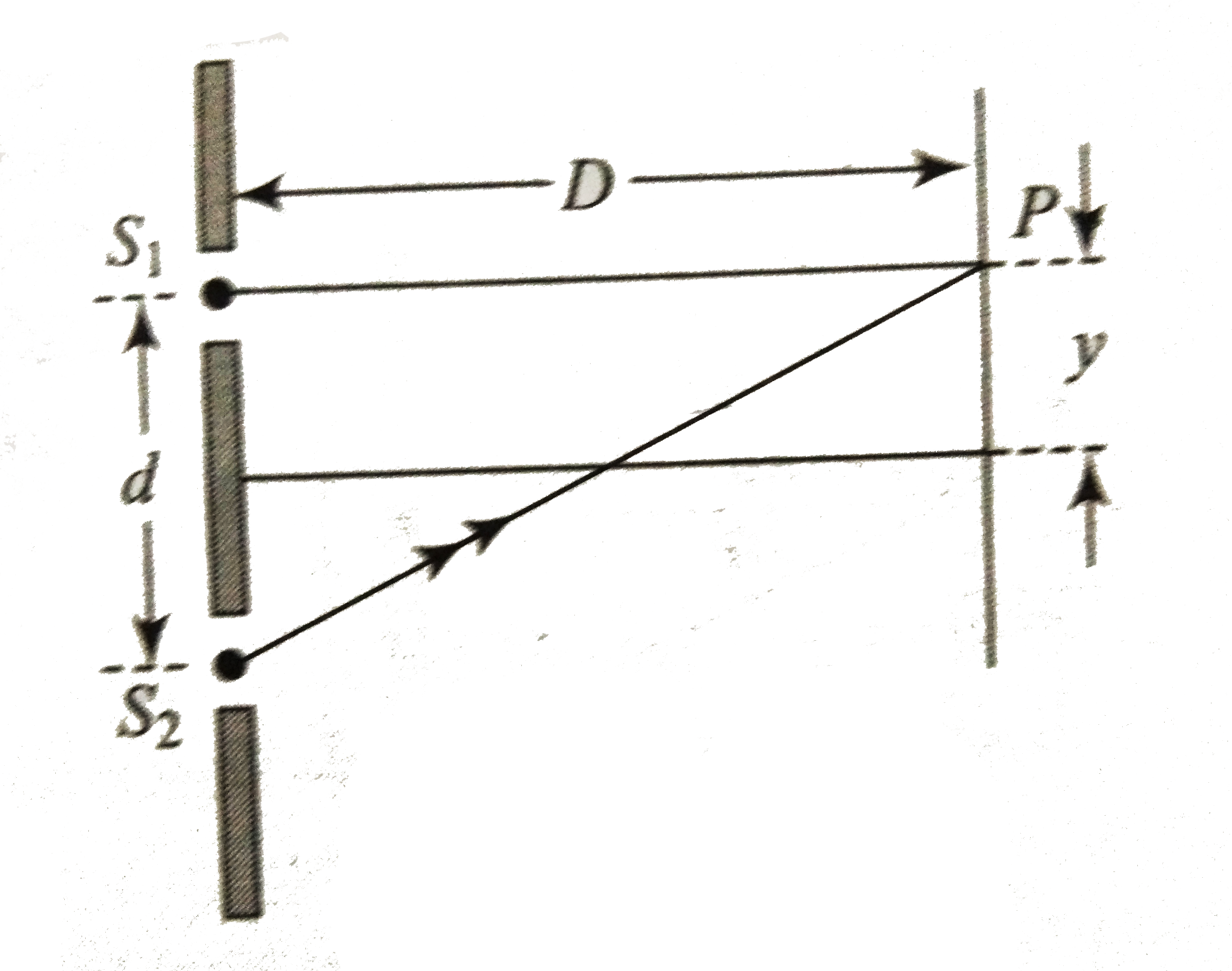 View Solution
View SolutionAt a point on the screen directly in front of one of the slits, find the missing wavelengths.
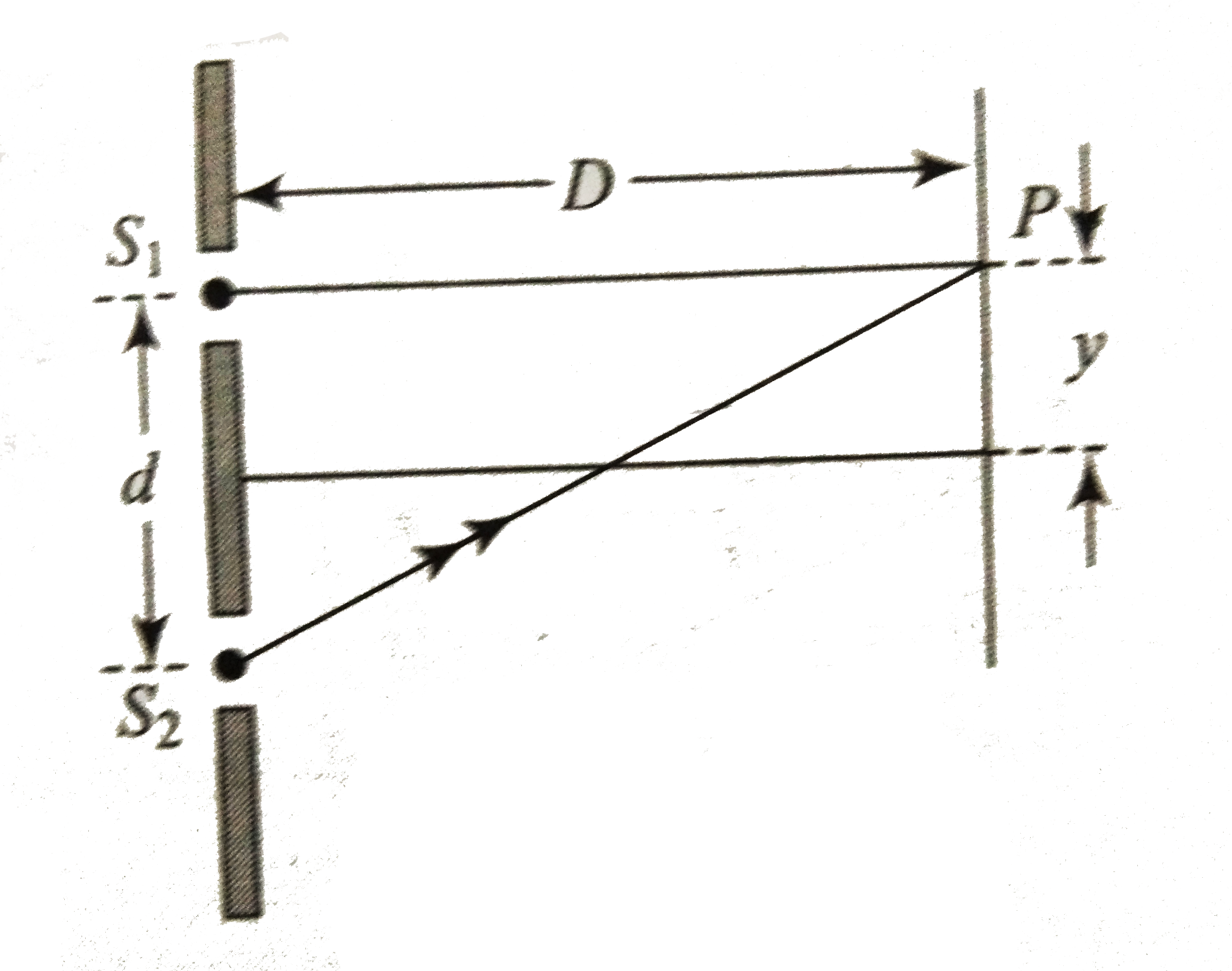 View Solution
View SolutionIn Young's double-slit experiment, the separation between the slits is d, distance between the slit and screen is D(D>>d), In the interference pattern, there is a maxima exactly in front of each slit. Then the possilbe wavelength(s) used in the experiment are
View SolutionIn Young's double-slit experiment , d is separation between the slits. Separation between plane of the slits and screen is D. Wavelength of light used is λ. Number of fringes per unit distance on the screen is
View SolutionIn a Young's double slit experiment, the separation between the slits is d, distance between the slit and screen is D(D>>d). In the interference pattern, there is a maximum exactly in front for each slit. Then the possible wavelength (s) used in the experiment are
View Solution

