Consider a function f whose domain is [-3, 4] and range is [-2, 2] with following graph.
Consider a function f whose domain is [-3, 4] and range is [-2, 2] with following graph. Domain and range of g(x)=f(|x|) is [a, b] and [c, d] respectively, then (b-a+c+d) is
Domain and range of g(x)=f(|x|) is [a, b] and [c, d] respectively, then (b−a+c+d) is

A

11

B

10

C

8

D

7

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:A

−3≤|x|≤4
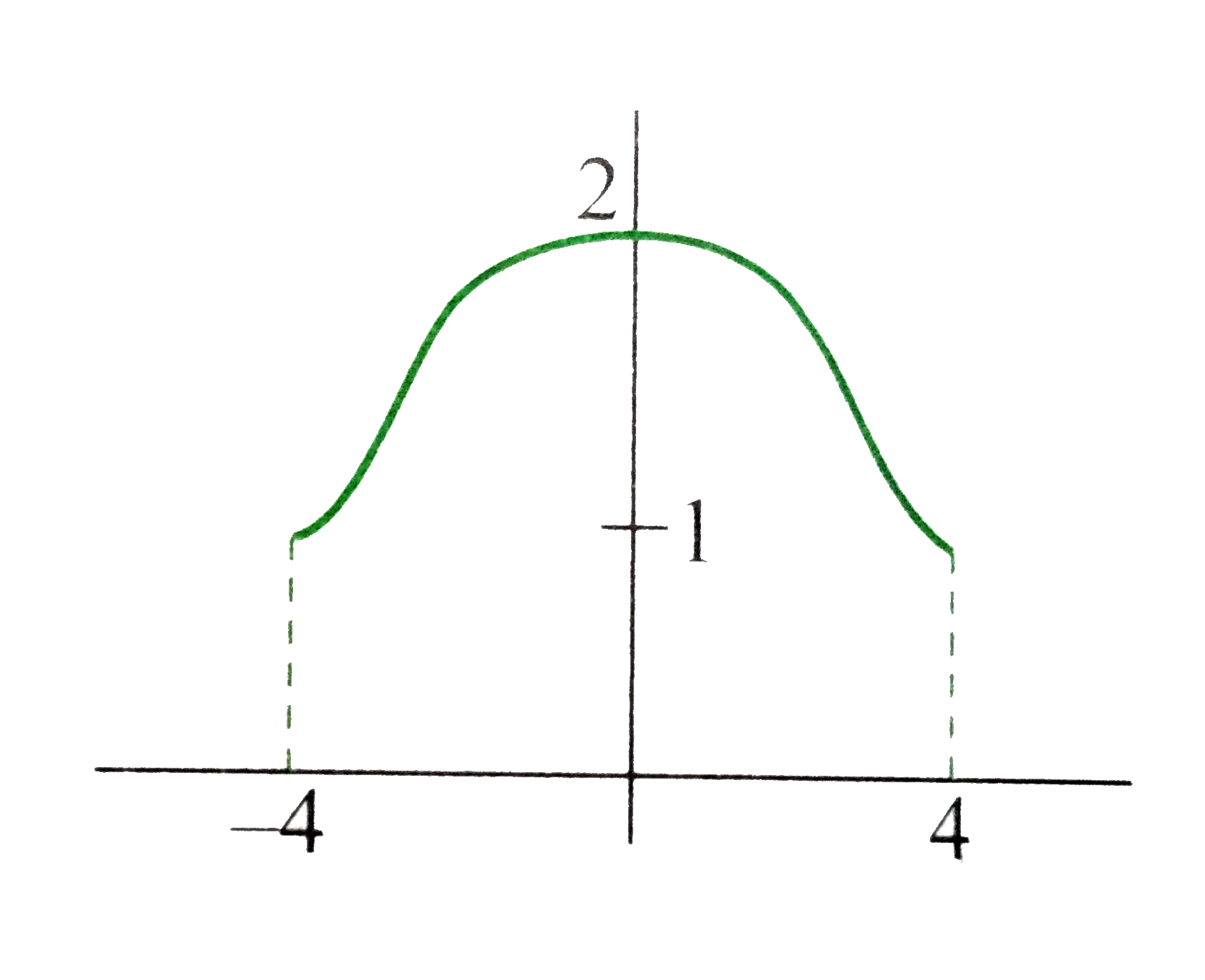
ocr_image
⇒0≤|x|≤4
⇒−4≤x≤4
⇒ Domain of g=[−4,4]
∴(b−a)=8
Also range of g(x)= is [1,2],(c+d)=3
∴(b−a+c+d)=11.

Updated on:21/07/2023
|
Class 12MATHSRELATIONS AND FUNCTIONS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Consider a function f whose domain is [-3, 4] and range is [-2, 2] with following graph.
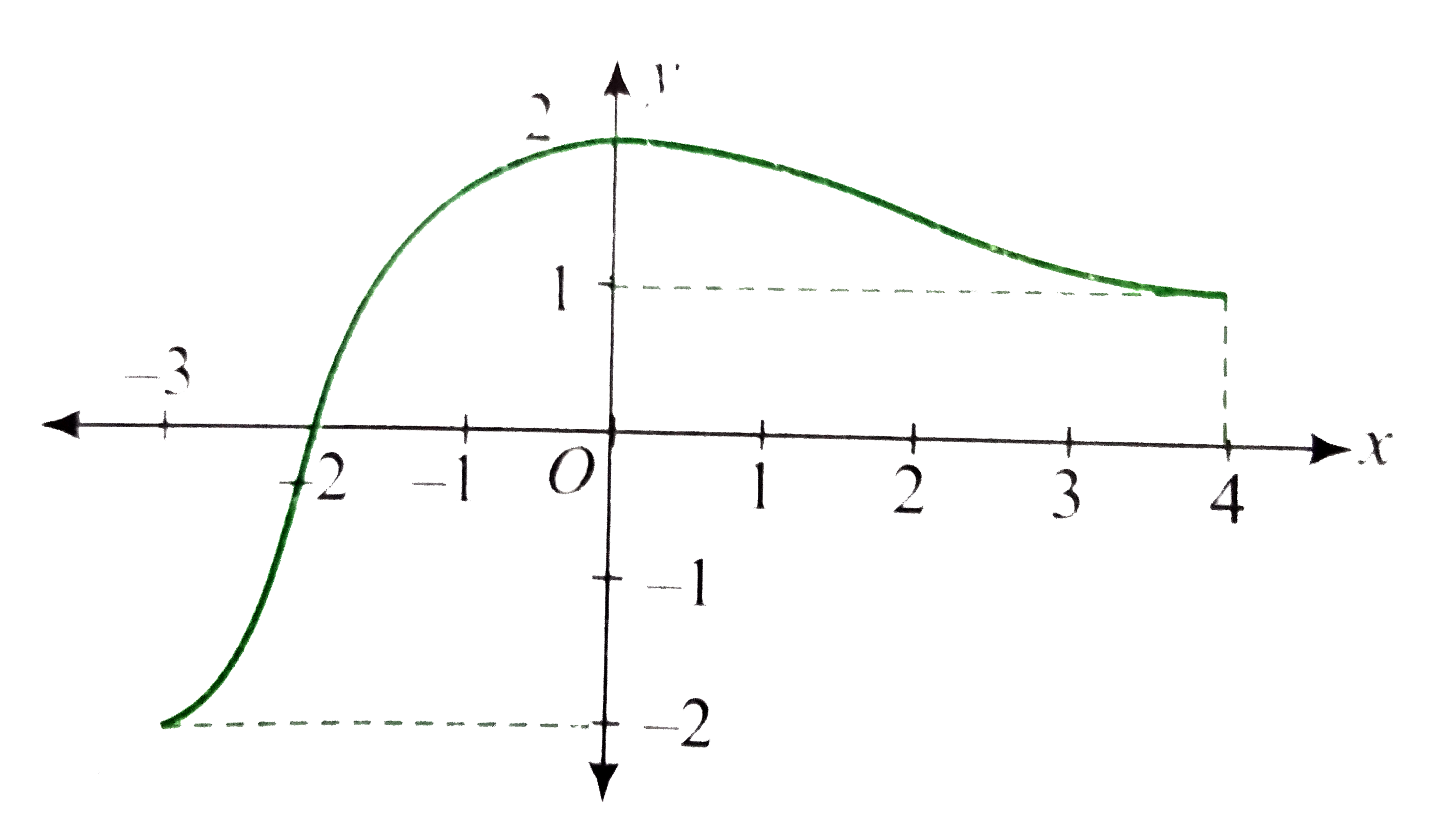
    ocr_image
    If h(x)=∣∣∣f(x)−32∣∣∣ has range [e, f] and n be number of real solutions of h(x)=14, then (n+e+2f) is

    A8
    B9
    C10
    D11

  • Domain and range of f(x)=|x−3|x−3 are respectively

    AR,[−1,1]
    BR−{3},{1,−1}
    CR+,R
    DNone of these

  • If rang of fraction f(x) whose domain is set of all real numbers is [−2,4], then range of functiong(x)=12f(2x+1) is equal to :

    A[−2,4]
    B[−1,.2]
    C[−3,9]
    D[−2,2]